Question

‘I’ is the perpendicular bisector of side BC of $△ABC$ meeting the circumference of the triangle of M opposite to A then
(a)$\mathrm{\angle }MBC=\mathrm{\angle }MCB$
(b) $\mathrm{\angle }BCM=\mathrm{\angle }BAM$
(c) $\mathrm{\angle }CMB=\mathrm{\angle }CAM$
(d) $\mathrm{\angle }BAM=\mathrm{\angle }CAM$

Answer 1

Hint: We start solving the problem by drawing the figure representing the given information. We then use the property that an arc in a circle subtends equal angles anywhere on the circumference. We then check whether both angles given in each option are satisfying this property to get the correct option.

Complete step-by-step solution:
Let us draw the figure representing the given information.

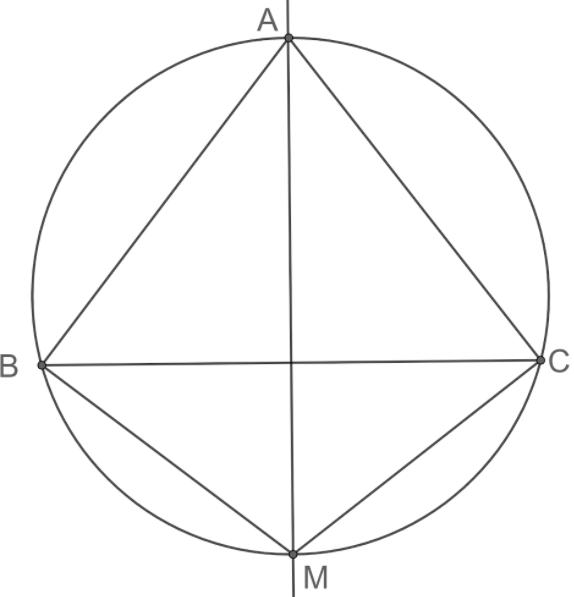
From the property of angle subtended by an arc, we know that an arc in a circle subtends equal angles anywhere on the circumference.
In this figure, BM is the chord.
$\mathrm{\angle }BAM\mathrm{\&}\mathrm{\angle }BCM$ is subtended at the end of this chord.
Hence, by this property,
$\mathrm{\angle }BCM=\mathrm{\angle }BAM$
Hence, option (b) is correct.
Now, we will start checking other options.
(A) $\mathrm{\angle }MBC=\mathrm{\angle }MCB$
Here, look at the angles $\mathrm{\angle }MBC\mathrm{\&}\mathrm{\angle }MCB$, we can see that the $\mathrm{\angle }MBC$ is subtended at the arc MC and the angle $\mathrm{\angle }MCB$ is subtended at the arc BM.
For the property to get applied, the angles should be subtended at the same arc.
Therefore, option (A) is incorrect.
(C) $\mathrm{\angle }CMB=\mathrm{\angle }CAM$
Here, look at the angles $\mathrm{\angle }CMB\mathrm{\&}\mathrm{\angle }CAM$, we can see that the $\mathrm{\angle }CMB$ is subtended at the arc BC and the angle $\mathrm{\angle }CAM$is subtended at the arc CM.
For the property to get applied, the angles should be subtended at the same arc.
Therefore, option (C) is incorrect.
(D) $\mathrm{\angle }BAM=\mathrm{\angle }CAM$
Here, look at the angles $\mathrm{\angle }BAM\mathrm{\&}\mathrm{\angle }CAM$, we can see that the $\mathrm{\angle }BAM$ is subtended at the arc BM and the angle $\mathrm{\angle }CAM$is subtended at the arc CM.
For the property to get applied, the angles should be subtended at the same arc.
Therefore, option (D) is incorrect.
Hence option B is the correct answer

Note: We can also find all the angles required by assuming one angle in the triangle and finding the other angles. We can see that the triangle ABC is an isosceles triangle with equal sides AB and AC. We can use the fact that the angles to equal sides in a triangle are equal and the sum of the angles in a triangle is ${180}^{\circ }$ to get the remaining angles. We can also see that the triangle MBC is also an isosceles triangle with equal sides MB and MC to get the other angles.