
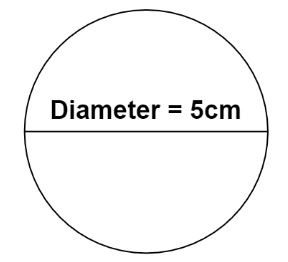
If the diameter of the circle is
Answer
393.9k+ views
Hint: Here in this question we want to find the area and circumference of a circle whose diameter is given to us as
Complete step by step answer:
The circle is a two dimensional figure and we have to determine the area, where the area is the region or space occupied by the circular field. To determine the area of a circle we have the standard formula
So,
So, we will get the area of the circle using the formula in the unit square centimetres.
To find the area of a circle, we use formula
By substituting, we get,
Therefore the area of a circle with a radius
Further simplifying the calculations, we have,
On further simplification and representing it in decimal expression, we have,
Hence the area of a circle whose diameter is
Now, the circumference of the circle can be calculated using the formula
So, we get,
Putting the value of
Therefore, the circumference of a circle with a diameter
Note: The circle is a two-dimensional figure and we have to determine the area, where the area is the region or space occupied by the circular field. The area of a circle is defined as the region occupied by the circular region. The radius is denoted by r or R. Value of
Complete step by step answer:
The circle is a two dimensional figure and we have to determine the area, where the area is the region or space occupied by the circular field. To determine the area of a circle we have the standard formula
So,
So, we will get the area of the circle using the formula in the unit square centimetres.
To find the area of a circle, we use formula
By substituting, we get,
Therefore the area of a circle with a radius
Further simplifying the calculations, we have,
On further simplification and representing it in decimal expression, we have,
Hence the area of a circle whose diameter is
Now, the circumference of the circle can be calculated using the formula
So, we get,

Putting the value of
Therefore, the circumference of a circle with a diameter
Note: The circle is a two-dimensional figure and we have to determine the area, where the area is the region or space occupied by the circular field. The area of a circle is defined as the region occupied by the circular region. The radius is denoted by r or R. Value of
Latest Vedantu courses for you
Grade 10 | CBSE | SCHOOL | English
Vedantu 10 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹35,000 per year
EMI starts from ₹2,916.67 per month
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Difference Between Plant Cell and Animal Cell

Given that HCF 306 657 9 find the LCM 306 657 class 9 maths CBSE

The highest mountain peak in India is A Kanchenjunga class 9 social science CBSE

What is the difference between Atleast and Atmost in class 9 maths CBSE

What was the capital of the king Kharavela of Kalinga class 9 social science CBSE




