Question

If the distance between foci of an ellipse is half the length of it’s latus rectum, then the eccentricity of the ellipse is,
( a ) $\dfrac{1}{2}$
( b ) $\sqrt{2}-1$
( c ) $\dfrac{\sqrt{2}-1}{2}$
( d ) $\dfrac{2\sqrt{2}-1}{2}$

Answer 1

Hint: To solve this question, what we will use the formula of latus rectum and foci and we will put them in equals as per the condition i.e. the distance between foci of an ellipse is half the length of it’s latus rectum and then, we will solve to get the value of eccentricity of ellipse which is equals to e.

Complete step-by-step answer:
Before we solve the question, let us see what an ellipse is.

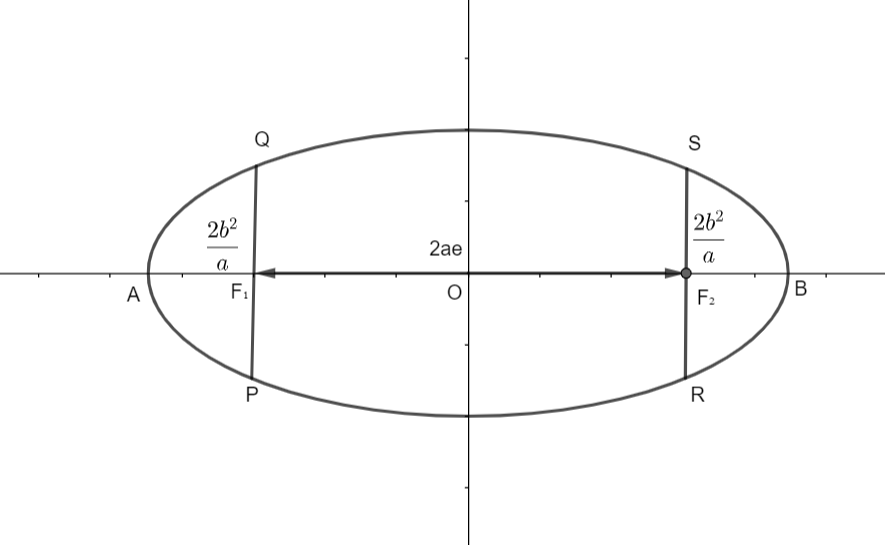
An ellipse is a plane curve surrounding two focal points, such that for all points on the curve, the sum of the two distances to the focal points is a constant. And, it’s equation is $\dfrac{{{x}^{2}}}{{{a}^{2}}}+\dfrac{{{y}^{2}}}{{{b}^{2}}}=1$ .
The given figure is of an ellipse. It has two foci ${{F}_{1}},{{F}_{2}}$ which are equidistant from the centre point and is equals to 2ae where e denotes eccentricity of ellipse.
PQ and RS denote latus rectum which is equal to $\dfrac{2{{b}^{2}}}{a}$ .
Now, we know that the distance between foci is 2ae and the latus rectum is equal to $\dfrac{2{{b}^{2}}}{a}$. In question it is given that distance between foci is half of that length of latus rectum.
So, we can write the above condition as,
$2ae=\dfrac{1}{2}\left( \dfrac{2{{b}^{2}}}{a} \right)$
On simplifying, we get
$2ae=\left( \dfrac{{{b}^{2}}}{a} \right)$
$2{{a}^{2}}e={{b}^{2}}$
We know that, ${{b}^{2}}={{a}^{2}}\left( 1-{{e}^{2}} \right)$
$2{{a}^{2}}e={{a}^{2}}\left( 1-{{e}^{2}} \right)$
On simplification, we get
$2e=\left( 1-{{e}^{2}} \right)$
${{e}^{2}}+2e-1=0$
Using quadratic formula $e=\dfrac{-b\pm \sqrt{{{b}^{2}}-4ac}}{2a}$on ${{e}^{2}}+2e-1=0$, to evaluate value of e, we get
$e=\dfrac{-1\pm \sqrt{{{4}^{2}}-4(1)(-1)}}{2a}$
On simplifying we get
$e=-1\pm \sqrt{2}$
From options, ( b ) is given
So, the correct answer is “Option b”.

Note: To solve this question, one must have knowledge of what an ellipse is and it’s diagram as it helps in visualising the question easily. Always remember that general equation of ellipse is $\dfrac{{{x}^{2}}}{{{a}^{2}}}+\dfrac{{{y}^{2}}}{{{b}^{2}}}=1$and for $a{{x}^{2}}+bx+c=0$ , quadratic formula to evaluate value of x is $x=\dfrac{-b\pm \sqrt{{{b}^{2}}-4ac}}{2a}$ . Calculation should be done very accurately and carefully as it may affect the answer and substitute the values in such a way to make complex equations simpler.