Question

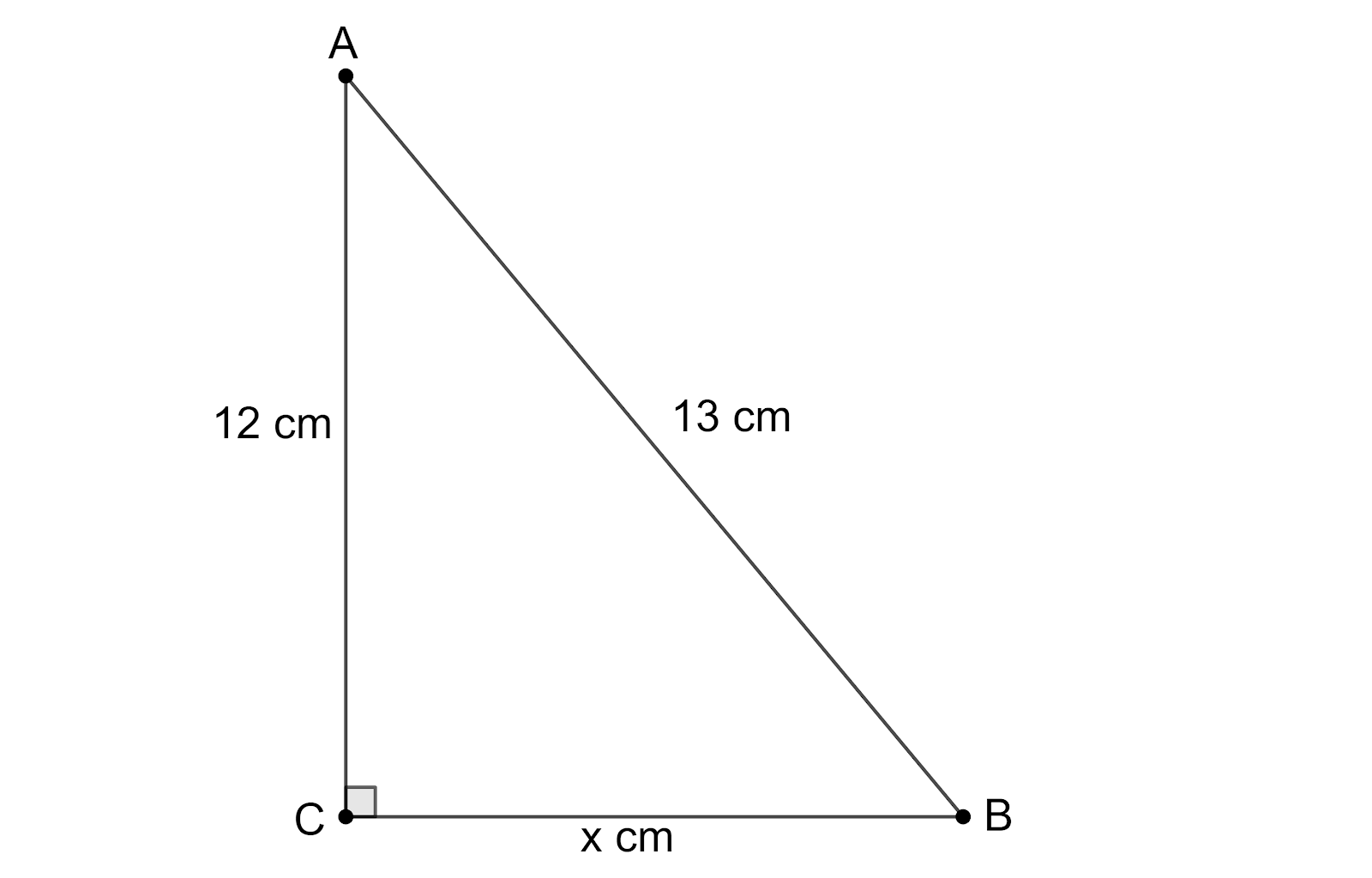
If the lengths of the sides of a right-angle triangle are given as 12 cm, x cm and 13 cm as shown below, then find the value of x?

Answer 1

Hint: We start solving the problem by finding the hypotenuse of the side by using the fact that the side opposite to the right angle in the right-angle triangle is defined as hypotenuse. We then recall the Pythagoras theorem as the square of the hypotenuse is equal to the sum of the squares of the remaining sides in a right-angle triangle. We apply Pythagora's theorem to the given sides of triangle and then make the necessary calculations to get the required value of x.

Complete step-by-step answer:
According to the problem, we are given that the lengths of the sides of the right-angle triangle are 12 cm, x cm and 13 cm as shown below. We need to find the value of x.
Let us redraw the given figure.

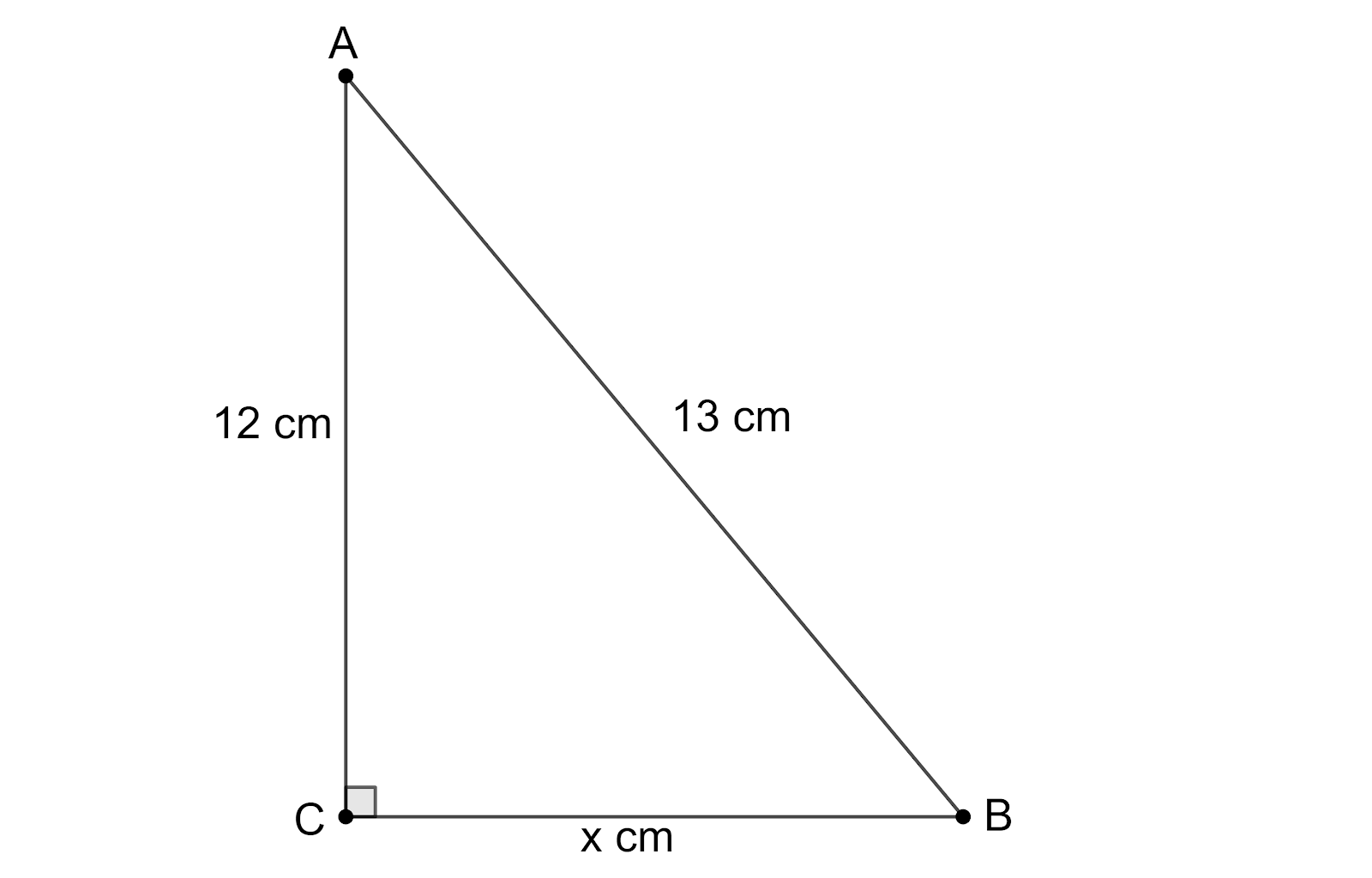
We know that the side opposite to the right angle in right-angle triangle is defined as hypotenuse, which is the largest side of the right-angle triangle.
From figure, we can see that AB is the hypotenuse.
From Pythagoras theorem, we know that the square of the hypotenuse is equal to the sum of the squares of the remaining sides in a right-angle triangle.
From figure, we have $A{{B}^{2}}=A{{C}^{2}}+C{{B}^{2}}$.
$\Rightarrow {{13}^{2}}={{12}^{2}}+{{x}^{2}}$.
$\Rightarrow 169=144+{{x}^{2}}$.
$\Rightarrow 25={{x}^{2}}$.
$\Rightarrow x=5cm$.
∴ The value of x is 5 cm.

Note: We can also find the solve the problem as shown below:

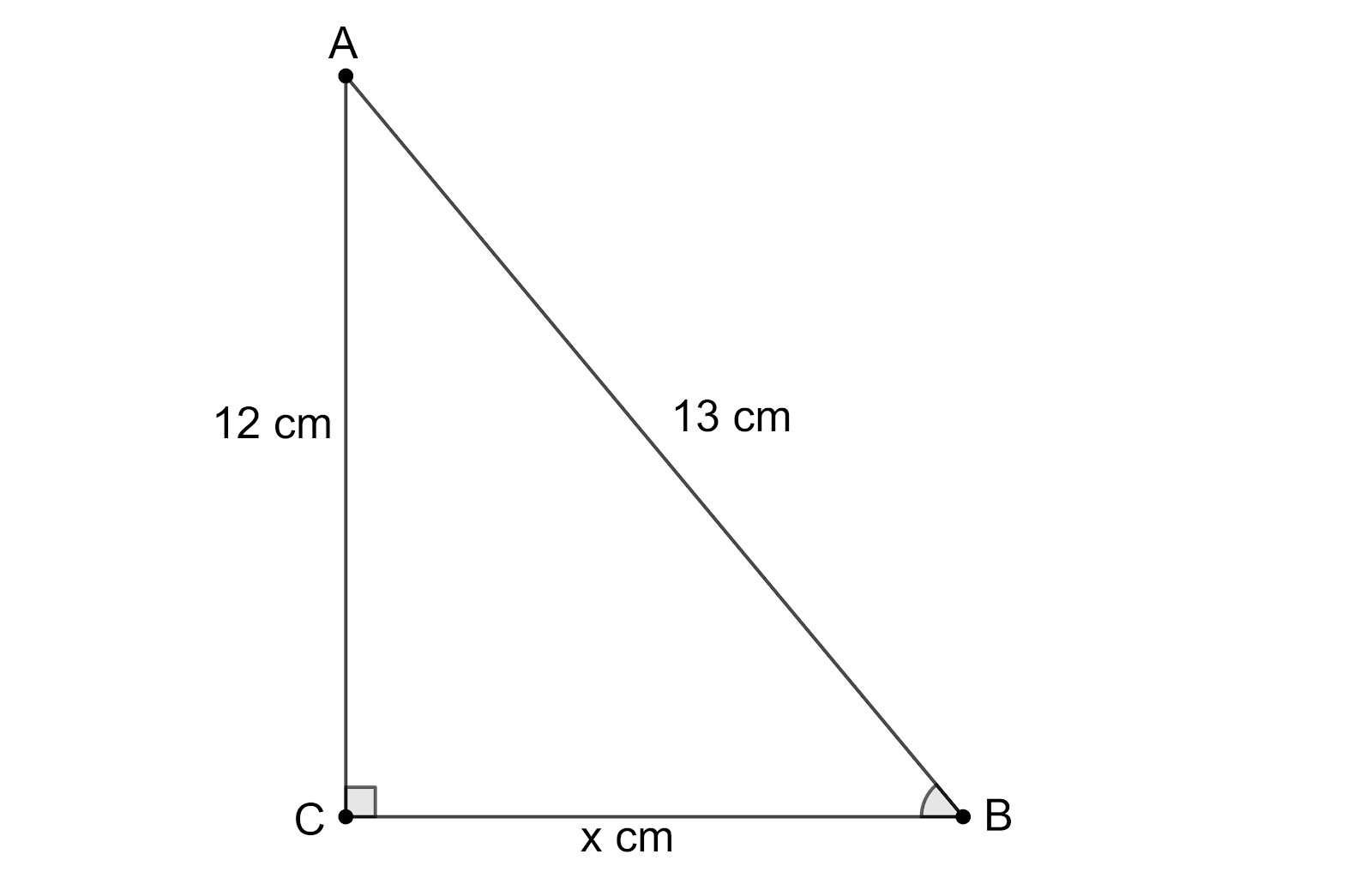
We know that $\sin B=\dfrac{AC}{AB}=\dfrac{12}{13}$ and $\cos B=\dfrac{CB}{AB}=\dfrac{x}{13}$.
From trigonometric identities, we know that ${{\sin }^{2}}B+{{\cos }^{2}}B=1$.
So, we have ${{\left( \dfrac{12}{13} \right)}^{2}}+{{\left( \dfrac{x}{13} \right)}^{2}}=1$.
$\Rightarrow \dfrac{144}{169}+\dfrac{{{x}^{2}}}{169}=1$.
$\Rightarrow \dfrac{{{x}^{2}}}{169}=1-\dfrac{144}{169}$.
$\Rightarrow \dfrac{{{x}^{2}}}{169}=\dfrac{169-144}{169}$.
$\Rightarrow \dfrac{{{x}^{2}}}{169}=\dfrac{25}{169}$.
$\Rightarrow {{x}^{2}}=25$.
$\Rightarrow x=5$ cm.