Question

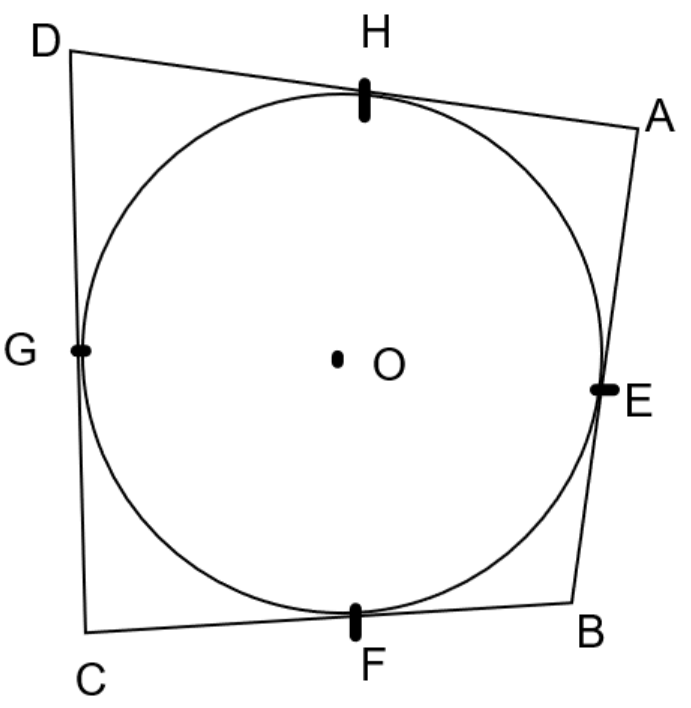
If the sides of a quadrilateral touch a circle, prove that the sum of opposite sides is equal to the sum of the other pairs.

Answer 1

Hint: We have to show that the sum of opposite sides of the quadrilateral which touches a circle is equal to the sum of the other pair of sides. We solve this question using the properties of tangents. First we will show a relation for all the tangents which will be formed and then we will substitute the values of the relations of the tangents such that on adding the tangents we get the relation that the sum of opposite sides of the quadrilateral are equal to the other pair.

Complete step-by-step solution:
Given :
$$ABCD$$ is a quadrilateral such that it touches the circle with centre $$O$$ at $$E$$, $$F$$, $$G$$ and $$H$$.
To prove :
$$AD+BC=AB+CD$$
Proof :
We know that the property of tangents of a circle states that the tangents drawn from the same external points onto the point on the circle are always equal in length.
So, we can state that using the above stated property of tangents that :
$$AE=AH--\left(1\right)$$
[Tangents originated from the same external point $$A$$]
$$BE=BF--\left(2\right)$$
[Tangents originated from the same external point $$B$$]
$$CG=CF--\left(3\right)$$
[Tangents originated from the same external point $$C$$]
$$DG=DH--\left(4\right)$$
[Tangents originated from the same external point $$D$$]
Now, we will add the equation $$\left(1\right)$$, $$\left(2\right)$$, $$\left(3\right)$$ and $$\left(4\right)$$ such that we will obtain the required relation.
On adding the equations, we get
$$AE+BE+CG+DG=AH+BF+CF+DH---\left(5\right)$$
Now, we also know that the sides of the quadrilateral can be written as :
$$CD=CG+DG$$
$$AB=AE+BE$$
similarly, for the other pair
$$BC=BF+CF$$
$$AD=AH+DH$$
Using the above sum of the sides of quadrilateral, we can write the equation $$\left(5\right)$$ as :
$$AB+CD=AD+BC$$
Hence proved that the sum of opposite sides is equal to the sum of the other pairs.

Note: We should take care of the relations of the tangents while adding the equation to obtain the relation for the sides of the quadrilateral. As if we reverse any one of the relation of the equation $$\left(1\right)$$, $$\left(2\right)$$, $$\left(3\right)$$ or $$\left(4\right)$$ we would not be able to get the desired result. As the sum of the sides would have a minus sign in them.
If we add the relation of the tangents as :
$$AH=AE$$ [which is opposite of equation $$\left(1\right)$$]
We will get the sum of the four equations as :
$$AH+BE+CG+DG=AE+BF+CF+DH$$
From this expression we won’t be able to prove the statement.