
If three acute angles of a quadrilateral measure 70° each, then the measure of the fourth angle is___________________.
Answer
426k+ views
Hint: Study the property of the quadrilateral. Learn about the measure of the four angles of the quadrilateral. The measure of four angles of a quadrilateral is 360 degrees.
Formula used: The sum of the four angles of a quadrilateral ABCD is,
Complete step by step solution:
We have given here a quadrilateral whose three angles are acute angles. Measure of each angle of the quadrilateral is
Now, we know that the sum of the four angles of a quadrilateral ABCD is,
So, the sum of three angles of the quadrilateral given here is,
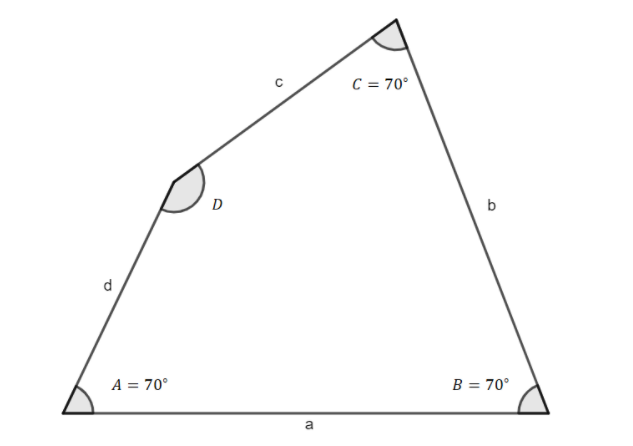
So, the fourth angle of the quadrilateral is the difference between 360 and the sum of the three angles.
Or,
So, the measure of the fourth angle is
So, the correct answer is “
Note: The sum of the three angles of a triangle is
Formula used: The sum of the four angles of a quadrilateral ABCD is,
Complete step by step solution:
We have given here a quadrilateral whose three angles are acute angles. Measure of each angle of the quadrilateral is
Now, we know that the sum of the four angles of a quadrilateral ABCD is,
So, the sum of three angles of the quadrilateral given here is,

So, the fourth angle of the quadrilateral is the difference between 360 and the sum of the three angles.
Or,
So, the measure of the fourth angle is
So, the correct answer is “
Note: The sum of the three angles of a triangle is
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Difference Between Plant Cell and Animal Cell

Given that HCF 306 657 9 find the LCM 306 657 class 9 maths CBSE

The highest mountain peak in India is A Kanchenjunga class 9 social science CBSE

What is the difference between Atleast and Atmost in class 9 maths CBSE

What is pollution? How many types of pollution? Define it




