
If two sides of a triangle are unequal, the angle opposite to the longer side is larger (or greater). You may prove this theorem by taking a point P on BC such that CA = CP.
Answer
440.1k+ views
4 likes
Hint: First write the theorem clearly and understand the term present in the theorem. Take a point opposite to the general angle, such that the distance of a point from the vertices is the same as the distance between the vertices. Use the theorems you know on the sides and prove the required theorem.
Complete step-by-step answer:
Given theorem in the question can be written in the form of:
If two sides of a triangle are unequal, the angle opposite to the longer side is larger.
Let us assume a triangle ABC such that the sides are as AB > AC.
By theorem, we need to prove that angle C > angle B.
Now, take a point P on line AB such that AP = AC. Join the two points C and P to set CP. Let us assume angle APC is
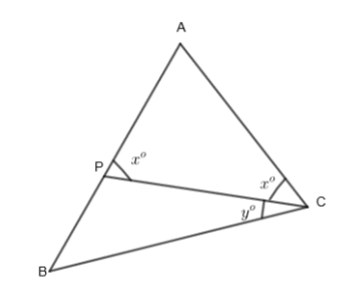
We know that AP = AC. If two sides are equal in a triangle, then the angles opposite to the sides are equal. Applying this condition to the triangle APC, we get the angle APC and angle ACP, all equal. As angle APC is
On line AB, we can say that it is a straight line which is equal to
By substituting the value of angle APC, we get the equation as,
By subtracting x on both the sides of the equation, we get it as,
We know the theorem that sum of all the angles in a triangle is
By substituting the value of BPC from the above equation, we get,
By substituting the value of PCB as per our assumption, we get,
By canceling out the common terms, we can write it as,
By adding x and subtracting y on both the sides, we get,
From the diagram, we can say that,
From the diagram, we can say that,
By substituting the values, we get it in the form of
From (i) and (ii), we can say the inequality in the form of
Hence, we have proved that the angle opposite to the greater side is larger.
Note: Be careful while marking the angles in the diagram. As when you miss any angle, you may not get the idea to proceed. The idea of making the angles as sum, the difference of angles is very important. This is derived from a small idea that the sum is greater than the difference if the numbers are positive. Here, the angles are positive.
Complete step-by-step answer:
Given theorem in the question can be written in the form of:
If two sides of a triangle are unequal, the angle opposite to the longer side is larger.
Let us assume a triangle ABC such that the sides are as AB > AC.

By theorem, we need to prove that angle C > angle B.
Now, take a point P on line AB such that AP = AC. Join the two points C and P to set CP. Let us assume angle APC is

We know that AP = AC. If two sides are equal in a triangle, then the angles opposite to the sides are equal. Applying this condition to the triangle APC, we get the angle APC and angle ACP, all equal. As angle APC is
On line AB, we can say that it is a straight line which is equal to
By substituting the value of angle APC, we get the equation as,
By subtracting x on both the sides of the equation, we get it as,
We know the theorem that sum of all the angles in a triangle is
By substituting the value of BPC from the above equation, we get,
By substituting the value of PCB as per our assumption, we get,
By canceling out the common terms, we can write it as,
By adding x and subtracting y on both the sides, we get,
From the diagram, we can say that,
From the diagram, we can say that,
By substituting the values, we get it in the form of
From (i) and (ii), we can say the inequality in the form of
Hence, we have proved that the angle opposite to the greater side is larger.
Note: Be careful while marking the angles in the diagram. As when you miss any angle, you may not get the idea to proceed. The idea of making the angles as sum, the difference of angles is very important. This is derived from a small idea that the sum is greater than the difference if the numbers are positive. Here, the angles are positive.
Latest Vedantu courses for you
Grade 10 | CBSE | SCHOOL | English
Vedantu 10 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
Recently Updated Pages
Master Class 4 Maths: Engaging Questions & Answers for Success

Master Class 4 English: Engaging Questions & Answers for Success

Master Class 4 Science: Engaging Questions & Answers for Success

Class 4 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Difference Between Plant Cell and Animal Cell

Given that HCF 306 657 9 find the LCM 306 657 class 9 maths CBSE

The highest mountain peak in India is A Kanchenjunga class 9 social science CBSE

What is the difference between Atleast and Atmost in class 9 maths CBSE

What is pollution? How many types of pollution? Define it




