
In a convex nonagon all the diagonals are drawn. These diagonals intersect each other at
Answer
499.5k+ views
Hint: Draw possible figures of the convex nonagon and use the concept of convexity to find that find out that diagonals can only intersect inside. Find how many vertices are required for one point of intersection of diagonals and then you can use a combination formula to get the result.
Complete step by step answer:
A nonagon is a polygon with 9 equal sides and 9 vertices. It is given in the question that the nonagon is convex; that means if we take any two points inside the nonagon and join the line segment all the points of the line segment will lie inside the nonagon. We also know that in a convex nonagon no angles are greater than straight angles.
The given question states that the diagonals intersect each other at
Let us consider the possible diagonals inside the nonagon. Let us take alternating vertices (leaving one vertex and joining the next ) and join them to find a point of intersection. We show this in the following figure
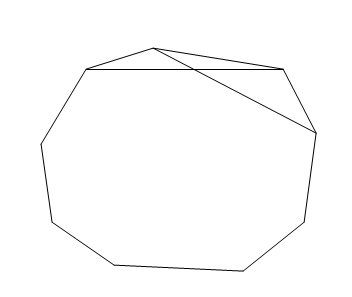
Let us join a pair of vertices who have two vertices between them. We take another such pair of vertices and join them to find a point of intersection. We show this in the following figure.
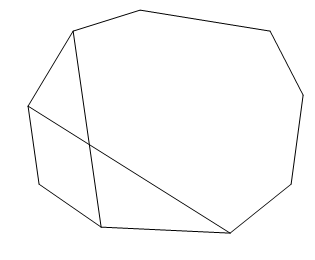
We can also join a pair of alternating vertices and a pair of vertices with two vertices in between them. We show this by the following figure.

We draw the figure to illustrate that there are no points of intersection of diagonals outside the nonagon. We see that the nonagon is convex which means the diagonal can only intersect in the interior of the nonagon.
We also observe from the figure that we need at least 4 vertices to find one point of intersection. So we can choose any 4 out 9 vertices and can find a point of intersection. So we use the combination formula
So the maximum value of
Note: The important word in this problem is convex which means we do not have to care about points of intersection outside the nonagon which is the case of concave nonagon. The extra information about points of intersection on and outside nonagon is there to distract only.
Complete step by step answer:
A nonagon is a polygon with 9 equal sides and 9 vertices. It is given in the question that the nonagon is convex; that means if we take any two points inside the nonagon and join the line segment all the points of the line segment will lie inside the nonagon. We also know that in a convex nonagon no angles are greater than straight angles.
The given question states that the diagonals intersect each other at
Let us consider the possible diagonals inside the nonagon. Let us take alternating vertices (leaving one vertex and joining the next ) and join them to find a point of intersection. We show this in the following figure

Let us join a pair of vertices who have two vertices between them. We take another such pair of vertices and join them to find a point of intersection. We show this in the following figure.

We can also join a pair of alternating vertices and a pair of vertices with two vertices in between them. We show this by the following figure.

We draw the figure to illustrate that there are no points of intersection of diagonals outside the nonagon. We see that the nonagon is convex which means the diagonal can only intersect in the interior of the nonagon.
We also observe from the figure that we need at least 4 vertices to find one point of intersection. So we can choose any 4 out 9 vertices and can find a point of intersection. So we use the combination formula
So the maximum value of
Note: The important word in this problem is convex which means we do not have to care about points of intersection outside the nonagon which is the case of concave nonagon. The extra information about points of intersection on and outside nonagon is there to distract only.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Basicity of sulphurous acid and sulphuric acid are

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 4 Maths: Engaging Questions & Answers for Success

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Difference Between Plant Cell and Animal Cell

Given that HCF 306 657 9 find the LCM 306 657 class 9 maths CBSE

The highest mountain peak in India is A Kanchenjunga class 9 social science CBSE

What is the difference between Atleast and Atmost in class 9 maths CBSE

What is pollution? How many types of pollution? Define it




