
In a hemispherical bowl of
Answer
490.8k+ views
Hint: Here it is given that there is a bowl of given radius with ice-cream. We have to find the volume of the bowl. We can say that a hemisphere is exactly half of a sphere. In general, a sphere makes exactly two hemispheres. Substitute the radius so we can find the volume of the hemisphere.
Formula used: Let us consider,
Then, the volume of the bowl is
Complete step-by-step answer:
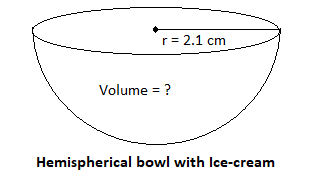
It is given that; the radius of the hemispherical bowl is
We have to find the volume of the bowl.
Let us consider,
Substitute
The volume of the bowl is
We will take
Simplifying, we get,
The volume of the bowl is
Simplifying again we get,
The volume of the hemispherical bowl is
Note: A sphere is defined as a set of points in three-dimension, and all the points lying on the surface are equidistant from the centre. When a plane cuts across the sphere at the centre or equal parts, it forms a hemisphere.
We can say that a hemisphere is exactly half of a sphere. In general, a sphere makes exactly two hemispheres.
For an example of the hemisphere is our earth. Our earth consists of two hemispheres, namely the Southern Hemisphere and the Northern Hemisphere.
Formula used: Let us consider,
Then, the volume of the bowl is
Complete step-by-step answer:

It is given that; the radius of the hemispherical bowl is
We have to find the volume of the bowl.
Let us consider,
Substitute
The volume of the bowl is
We will take
Simplifying, we get,
The volume of the bowl is
Simplifying again we get,
The volume of the hemispherical bowl is
Note: A sphere is defined as a set of points in three-dimension, and all the points lying on the surface are equidistant from the centre. When a plane cuts across the sphere at the centre or equal parts, it forms a hemisphere.
We can say that a hemisphere is exactly half of a sphere. In general, a sphere makes exactly two hemispheres.
For an example of the hemisphere is our earth. Our earth consists of two hemispheres, namely the Southern Hemisphere and the Northern Hemisphere.
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Difference Between Plant Cell and Animal Cell

Given that HCF 306 657 9 find the LCM 306 657 class 9 maths CBSE

The highest mountain peak in India is A Kanchenjunga class 9 social science CBSE

What is the difference between Atleast and Atmost in class 9 maths CBSE

What is pollution? How many types of pollution? Define it




