
In a quadrilateral define the following: Adjacent sides.
Answer
480k+ views
Hint: In this question we have to define the adjacent sides of the quadrilateral. For that we have to explain about adjacent sides. Then with help of the definition we can analyze the quadrilateral to find the required result.
Complete step-by-step solution:
For this question requirement first we have to know about the adjacent side.
First the meaning of adjacent is an adjective meaning next to.
Adjacent sides are sides of a polygon that have a common vertex. Usually found in triangles and other polygons, two of the sides that meet at a vertex of the polygon are called adjacent sides. In other words we can say, in geometry, two sides that meet to create an angle are said to be adjacent. In trigonometry the adjacent side of a right triangle is the side next to the angle in question.
These are explained about the adjacent sides.
Now we are going know about the adjacent side of quadrilateral,
Two sides of a quadrilateral which have a common endpoint are called its adjacent sides.
Consider the quadrilateral
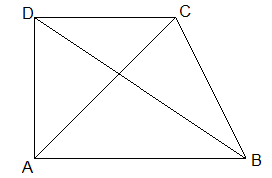
In quadrilateral
The four points
The four line segments
The line segments
From the property of quadrilateral and the definition of adjacent sides we will get,
In the given figure,
Note: We can observe that from the solution of the question, the center of a quadrilateral can be defined in several different ways. The vertex centroid comes from considering the quadrilateral as being empty but having equal mass at its vertices. The side centroid comes from considering the sides to have constant mass per unit length. The usual center called just centroid (center of area) comes from considering the surface of the quadrilateral as having constant density. These three points are generally not all the same point.
Complete step-by-step solution:
For this question requirement first we have to know about the adjacent side.
First the meaning of adjacent is an adjective meaning next to.
Adjacent sides are sides of a polygon that have a common vertex. Usually found in triangles and other polygons, two of the sides that meet at a vertex of the polygon are called adjacent sides. In other words we can say, in geometry, two sides that meet to create an angle are said to be adjacent. In trigonometry the adjacent side of a right triangle is the side next to the angle in question.
These are explained about the adjacent sides.
Now we are going know about the adjacent side of quadrilateral,
Two sides of a quadrilateral which have a common endpoint are called its adjacent sides.
Consider the quadrilateral

In quadrilateral
The four points
The four line segments
The line segments
From the property of quadrilateral and the definition of adjacent sides we will get,
In the given figure,
Note: We can observe that from the solution of the question, the center of a quadrilateral can be defined in several different ways. The vertex centroid comes from considering the quadrilateral as being empty but having equal mass at its vertices. The side centroid comes from considering the sides to have constant mass per unit length. The usual center called just centroid (center of area) comes from considering the surface of the quadrilateral as having constant density. These three points are generally not all the same point.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 4 Maths: Engaging Questions & Answers for Success

Master Class 4 English: Engaging Questions & Answers for Success

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Difference Between Plant Cell and Animal Cell

Given that HCF 306 657 9 find the LCM 306 657 class 9 maths CBSE

The highest mountain peak in India is A Kanchenjunga class 9 social science CBSE

What is the difference between Atleast and Atmost in class 9 maths CBSE

What is pollution? How many types of pollution? Define it




