Question

In a right-angled triangle ABC, if the hypotenuse $AB=p$ , then what \[\overrightarrow{AB}\cdot \overrightarrow{AC}+\overrightarrow{BA}\cdot \overrightarrow{BC}+\overrightarrow{CA}\cdot \overrightarrow{CB}\] equals to
A. $p$
B. \[{{p}^{2}}\]
C. \[2{{p}^{2}}\]
D. \[\dfrac{{{p}^{2}}}{2}\]

Answer 1

Hint: We have to find the value of \[\overrightarrow{AB}\cdot \overrightarrow{AC}+\overrightarrow{BA}\cdot \overrightarrow{BC}+\overrightarrow{CA}\cdot \overrightarrow{CB}\] . From, the figure, \[\overrightarrow{AC}\bot \overrightarrow{CB}\] , \[\overrightarrow{CA}\bot \overrightarrow{CB}\] and using the rule of vectors, we will get \[\overrightarrow{CA}\cdot \overrightarrow{CB}=0\] . Substituting these values in \[\overrightarrow{AB}\cdot \overrightarrow{AC}+\overrightarrow{BA}\cdot \overrightarrow{BC}+\overrightarrow{CA}\cdot \overrightarrow{CB}\] and after some rearrangements, use the using the triangle law of vector addition \[\left( \overrightarrow{AB}=\overrightarrow{AC}+\overrightarrow{CB} \right)\] and the property $\overrightarrow{a\cdot }\overrightarrow{a}={{\left| \overrightarrow{a} \right|}^{2}}$ , the required value will be obtained.

Complete step by step answer:
We have to find the value of \[\overrightarrow{AB}\cdot \overrightarrow{AC}+\overrightarrow{BA}\cdot \overrightarrow{BC}+\overrightarrow{CA}\cdot \overrightarrow{CB}\] .

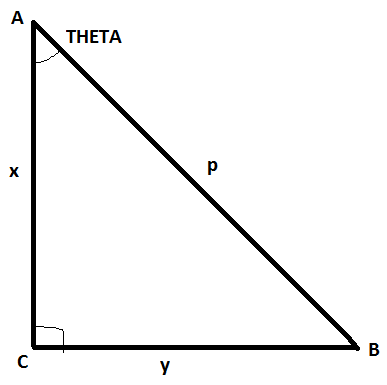
From the figure,
\[\overrightarrow{AC}\bot \overrightarrow{CB}\]
And \[\overrightarrow{CA}\bot \overrightarrow{CB}\]
We know that \[\overrightarrow{a}\cdot \overrightarrow{b}=\left| \overrightarrow{a} \right|\cdot \left| \overrightarrow{b} \right|\cdot \cos \theta \]
\[\Rightarrow \overrightarrow{CA}\cdot \overrightarrow{CB}=\left| \overrightarrow{CA} \right|\cdot \left| \overrightarrow{CB} \right|\cos \theta \]
This can be written as
\[\dfrac{\overrightarrow{CA}\cdot \overrightarrow{CB}}{\left| \overrightarrow{CA} \right|\cdot \left| \overrightarrow{CB} \right|}=\cos \theta \]
Given that the triangle is a right-angled triangle. Hence,
\[\dfrac{\overrightarrow{CA}\cdot \overrightarrow{CB}}{\left| \overrightarrow{CA} \right|\cdot \left| \overrightarrow{CB} \right|}=\cos 90=0\]
This can be written as
\[\overrightarrow{CA}\cdot \overrightarrow{CB}=0...(i)\]
Let us consider \[\overrightarrow{AB}\cdot \overrightarrow{AC}+\overrightarrow{BA}\cdot \overrightarrow{BC}+\overrightarrow{CA}\cdot \overrightarrow{CB}\]
Substituting \[(i)\] in the above equation, we will get
\[\overrightarrow{AB}\cdot \overrightarrow{AC}+\overrightarrow{BA}\cdot \overrightarrow{BC}+0\]
$=\overrightarrow{AB}\cdot \overrightarrow{AC}+\overrightarrow{BA}\cdot \overrightarrow{BC}$
We know that \[\overrightarrow{AB}=-\overrightarrow{BA}\] . So the above equation can be written as
\[\overrightarrow{AB}\cdot \overrightarrow{AC}-\overrightarrow{AB}\cdot \overrightarrow{BC}\]
Taking $\overrightarrow{AB}$ common, we will get
\[\overrightarrow{AB}\cdot \overrightarrow{(AC}-\overrightarrow{BC})\]
We know that \[\overrightarrow{BC}=-\overrightarrow{CB}\] . So the above equation can be written as
\[\overrightarrow{AB}\cdot \overrightarrow{(AC}+\overrightarrow{CB})...(ii)\]
Using triangle law of vector addition, that is, for a triangle shown below, $\overrightarrow{A}=\overrightarrow{B}+\overrightarrow{C}$

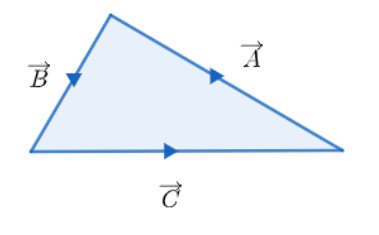
Similarly, we can express the same for the given triangle. That is,
\[\overrightarrow{AB}=\overrightarrow{AC}+\overrightarrow{CB}\]
Hence, $(ii)$ can be written as
\[\overrightarrow{AB}\cdot \overrightarrow{AB}\]
We know that $\overrightarrow{a\cdot }\overrightarrow{a}={{\left| \overrightarrow{a} \right|}^{2}}$
Hence, \[\overrightarrow{AB}\cdot \overrightarrow{AB}={{\left| \overrightarrow{AB} \right|}^{2}}\]
$={{\left| p \right|}^{2}}={{p}^{2}}$

So, the correct answer is “Option B”.

Note: The students can make an error if they don’t know how to write the dot product of two vectors. Also, the property of vectors and triangular law of vector addition is also very important to solve this question and hence need to be very thorough in this. There can also be a chance to make error in the equation \[\dfrac{\overrightarrow{CA}\cdot \overrightarrow{CB}}{\left| \overrightarrow{CA} \right|\cdot \left| \overrightarrow{CB} \right|}=\cos \theta \] by writing $\sin \theta $ instead of $\cos \theta $ .