
In an equilateral triangle , the inradius, circumradius, and one of the ex –radii are
In the ratio
$
{\text{A}}{\text{. 2:3:5}} \\
{\text{B}}{\text{. 1:2:3}} \\
{\text{C}}{\text{. 1:3:7}} \\
{\text{D}}{\text{. 3:7:9}} \\
$
Answer
491.1k+ views
Hint: To solve this question we have to have knowledge of inradius circumradius and ex-radius and then using the property of the solution of the triangle we have to find their ratio. And also keep in mind it is an equilateral triangle so all sides are the same.
Complete step-by-step answer:
We have given a equilateral triangle and we know inradius is denoted by ‘r’ and circumradius is denoted by ‘R’ and one of the ex-radius is ${r_1}$
We have to find $r:R:{r_1}$
Now we know,
Area of equilateral triangle =$\dfrac{{\sqrt 3 }}{4}{a^2}$
Perimeter of equilateral triangle = 3a =2s, $\therefore s = \dfrac{{3a}}{2}$
Now using the properties of solution of triangle
$r = \dfrac{\Delta }{s} = \dfrac{{\dfrac{{\sqrt 3 }}{4}{a^2}}}{{\dfrac{{3a}}{2}}} = \dfrac{a}{{2\sqrt 3 }}$
Now we know
$R = \dfrac{{abc}}{{4\Delta }}$ and here equilateral triangle triangle is given so a = b = c
$\therefore R = \dfrac{{{a^3}}}{{4 \times \dfrac{{\sqrt 3 }}{4}{a^2}}} = \dfrac{a}{{\sqrt 3 }}$
And we also know
${r_1} = \dfrac{\Delta }{{s - a}} = \dfrac{{\dfrac{{\sqrt 3 }}{4}{a^2}}}{{\dfrac{{3a}}{2} - a}} = \dfrac{{\sqrt 3 }}{4}{a^2} \times \dfrac{2}{a} = \dfrac{{\sqrt 3 }}{2}a$
Now we have
$r = \dfrac{a}{{2\sqrt 3 }},R = \dfrac{a}{{\sqrt 3 }},{r_1} = \dfrac{{\sqrt 3 }}{2}a$
So we have to find ratio of all these three
$r:R:{r_1} = \dfrac{a}{{2\sqrt 3 }}:\dfrac{a}{{\sqrt 3 }}:\dfrac{{\sqrt 3 }}{2}a$
On simplifying this we get,
1:2:3
Hence option B is the correct option.
Note: Whenever we get this type of question the key concept of solving is we should have remembered all this formulae ${r_1} = \dfrac{\Delta }{{s - a}},R = \dfrac{{abc}}{{4\Delta }},2s = {\text{perimeter}}$ then we can solve easily this question.
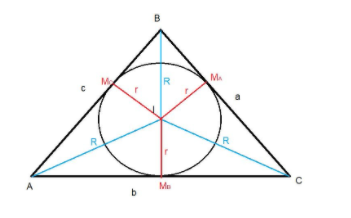
Complete step-by-step answer:
We have given a equilateral triangle and we know inradius is denoted by ‘r’ and circumradius is denoted by ‘R’ and one of the ex-radius is ${r_1}$
We have to find $r:R:{r_1}$
Now we know,
Area of equilateral triangle =$\dfrac{{\sqrt 3 }}{4}{a^2}$
Perimeter of equilateral triangle = 3a =2s, $\therefore s = \dfrac{{3a}}{2}$
Now using the properties of solution of triangle
$r = \dfrac{\Delta }{s} = \dfrac{{\dfrac{{\sqrt 3 }}{4}{a^2}}}{{\dfrac{{3a}}{2}}} = \dfrac{a}{{2\sqrt 3 }}$
Now we know
$R = \dfrac{{abc}}{{4\Delta }}$ and here equilateral triangle triangle is given so a = b = c
$\therefore R = \dfrac{{{a^3}}}{{4 \times \dfrac{{\sqrt 3 }}{4}{a^2}}} = \dfrac{a}{{\sqrt 3 }}$
And we also know
${r_1} = \dfrac{\Delta }{{s - a}} = \dfrac{{\dfrac{{\sqrt 3 }}{4}{a^2}}}{{\dfrac{{3a}}{2} - a}} = \dfrac{{\sqrt 3 }}{4}{a^2} \times \dfrac{2}{a} = \dfrac{{\sqrt 3 }}{2}a$
Now we have
$r = \dfrac{a}{{2\sqrt 3 }},R = \dfrac{a}{{\sqrt 3 }},{r_1} = \dfrac{{\sqrt 3 }}{2}a$
So we have to find ratio of all these three
$r:R:{r_1} = \dfrac{a}{{2\sqrt 3 }}:\dfrac{a}{{\sqrt 3 }}:\dfrac{{\sqrt 3 }}{2}a$
On simplifying this we get,
1:2:3
Hence option B is the correct option.
Note: Whenever we get this type of question the key concept of solving is we should have remembered all this formulae ${r_1} = \dfrac{\Delta }{{s - a}},R = \dfrac{{abc}}{{4\Delta }},2s = {\text{perimeter}}$ then we can solve easily this question.

Recently Updated Pages
Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Trending doubts
Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

What are the major means of transport Explain each class 12 social science CBSE

Which of the following properties of a proton can change class 12 physics CBSE

What is a transformer Explain the principle construction class 12 physics CBSE

Why is the cell called the structural and functional class 12 biology CBSE




