
In each pair of triangles in the following figure, parts bearing identical marks are congruent .State the test and correspondence of vertices by which triangles in each pair are congruent







Answer
420.3k+ views
Hint: In this question we need to check the conditions of two triangles to be congruent. Basically there are five conditions for two triangles to be congruent they are
Complete answer:
The triangles are said to be congruent if they are of the same shape and size. We may represent the congruence by the symbol
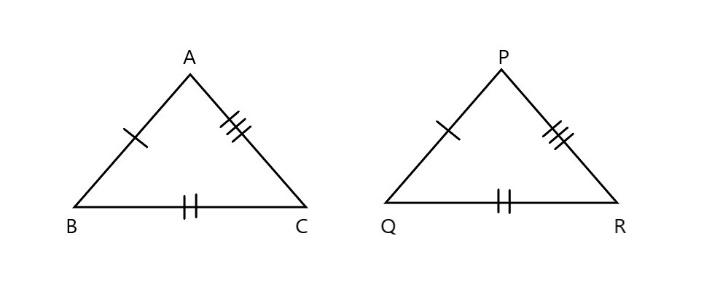
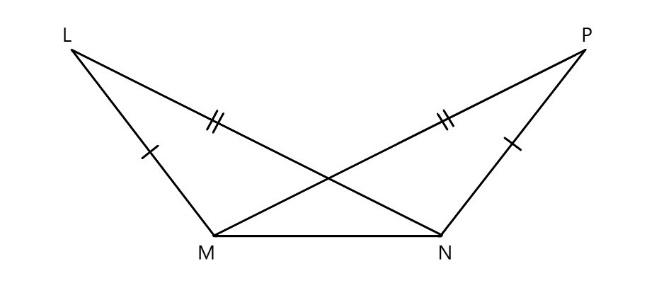
In the above figure in the given
Here all the corresponding sides are equal in length. Hence it follows
THUS, we can say that
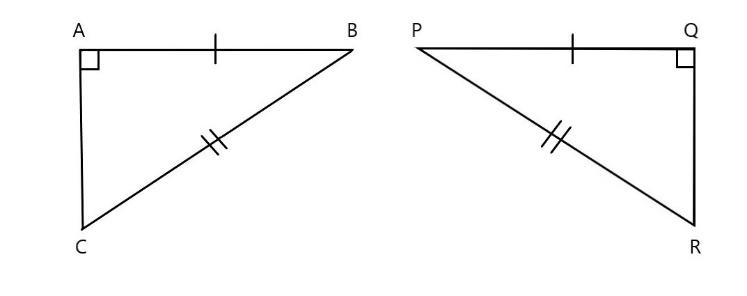
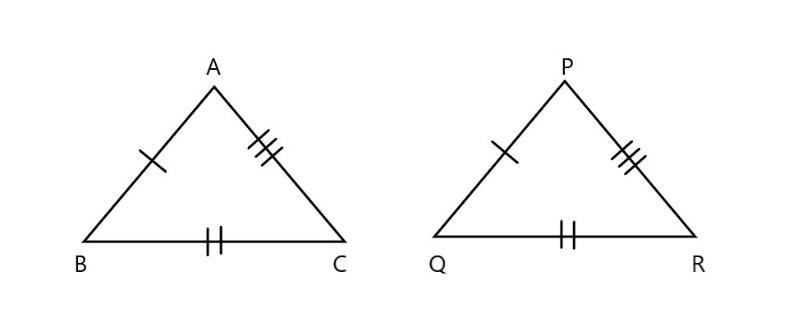
In the above figure in the given
Here all the corresponding sides are equal in length. Hence it follows
THUS, we can say that
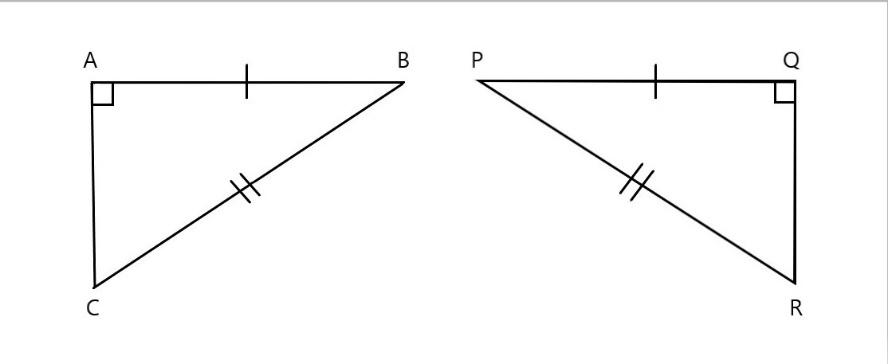
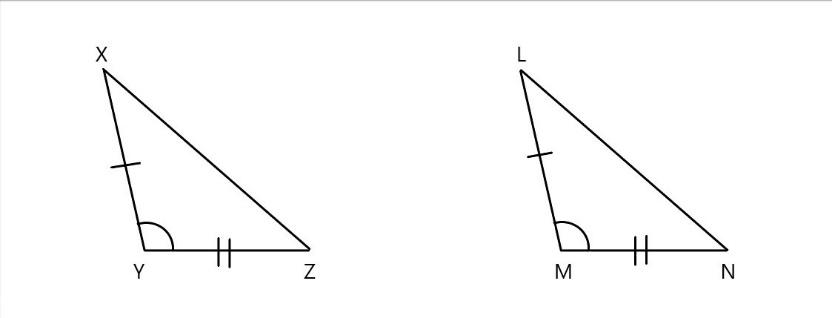
In the above figure in
Here all the corresponding sides are equal in length. Hence it follows
THUS, we can say that
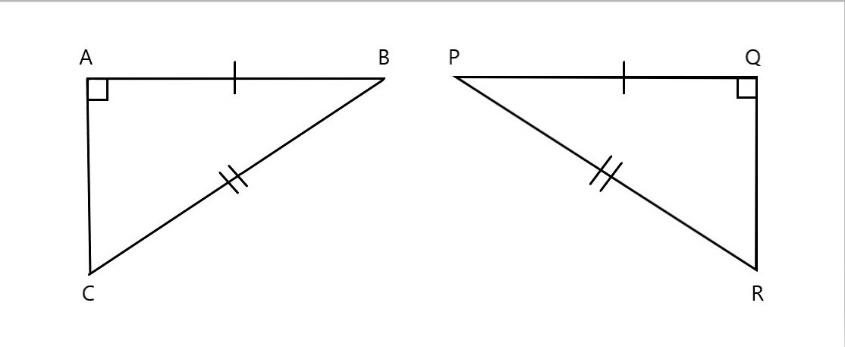
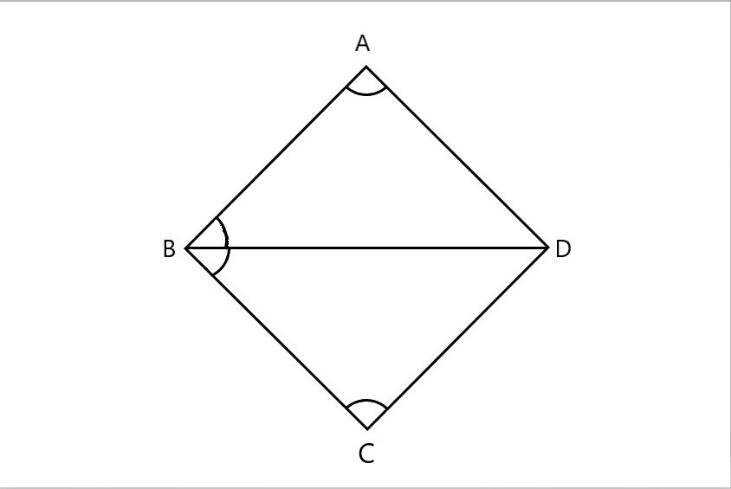
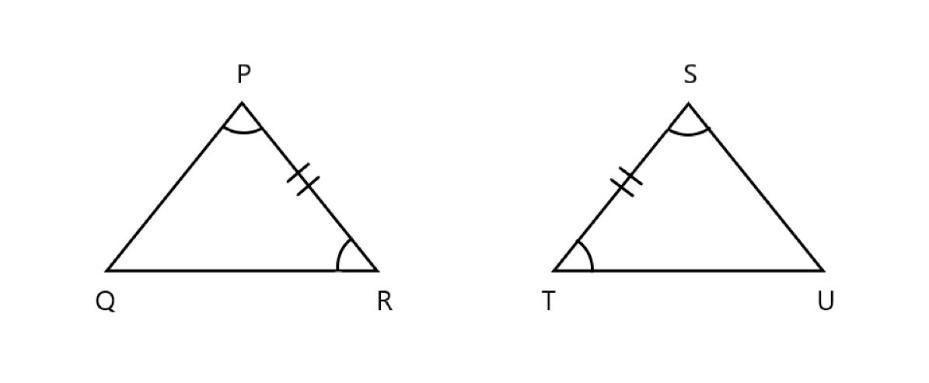
In the above figure in the given
Here all the corresponding sides are equal in length. Hence it follows
Thus, we can say that
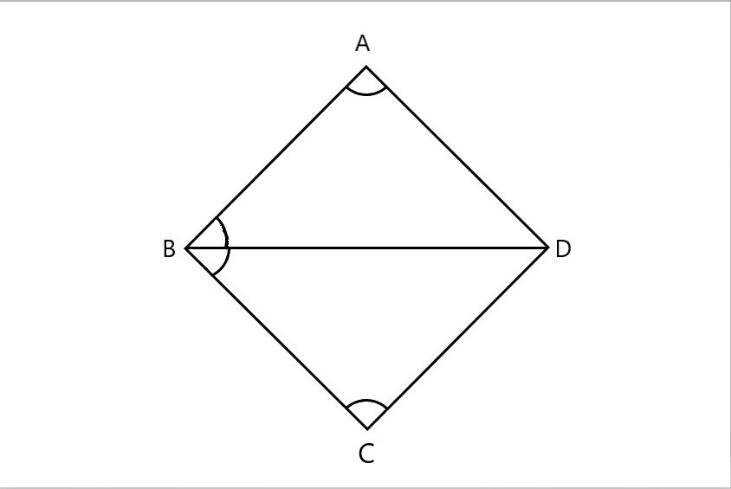
In the above figure in the given
Here all the corresponding sides are equal in length. Hence it follows
Thus, we can say that
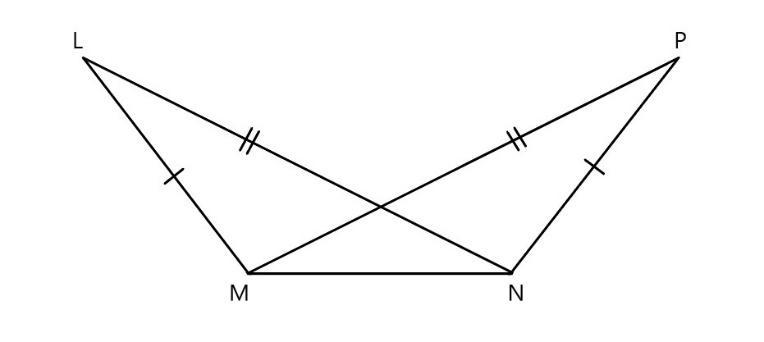
In the above figure in the given
Here all the corresponding sides are equal in length. Hence it follows
THUS, we can say that
Note:
Complete answer:
The triangles are said to be congruent if they are of the same shape and size. We may represent the congruence by the symbol

In the above figure in the given
Here all the corresponding sides are equal in length. Hence it follows
THUS, we can say that

In the above figure in the given
Here all the corresponding sides are equal in length. Hence it follows
THUS, we can say that

In the above figure in
Here all the corresponding sides are equal in length. Hence it follows
THUS, we can say that

In the above figure in the given
Here all the corresponding sides are equal in length. Hence it follows
Thus, we can say that

In the above figure in the given
Here all the corresponding sides are equal in length. Hence it follows
Thus, we can say that

In the above figure in the given
Here all the corresponding sides are equal in length. Hence it follows
THUS, we can say that
Note:
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Difference Between Plant Cell and Animal Cell

Given that HCF 306 657 9 find the LCM 306 657 class 9 maths CBSE

The highest mountain peak in India is A Kanchenjunga class 9 social science CBSE

What is the difference between Atleast and Atmost in class 9 maths CBSE

What is pollution? How many types of pollution? Define it




