
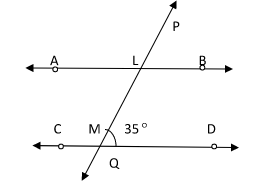
In figure, AB and CD are parallel lines intersected by a transversal PQ at L and M respectively. If

Answer
504.3k+ views
1 likes
Hint: Here we will have to apply concepts of –
Sum of all the angles on a straight line is
Complete step-by-step answer:
Given:
AB and CD are parallel lines (AB II CD) intersected by a transversal PQ at L and M respectively.
[ Transversal – A line that intersects two lines on the same plane intersecting at two different points.]
To find :
In this figure,
[ Anterior internal angles are a pair of angles on the inner side of each side of each of those two lines but on the opposite sides of the transversal]
We know that anterior interior angles are equal when two lines are parallel & there is a transversal.
AB and CD are parallel lines, AB II CD & PQ is transversal.
Hence, the value of
Now to find value of
We need to know that, Sum of all the angles on a straight line is
In the above figure, on line segment LM,
Hence the value of
Note: Alternate interior angles are equal, when lines are parallel to each other & there is a transversal so by implementing this concept we can get a linear equation to be solved carefully to get the ultimate answer.
Sum of all the angles on a straight line is
Complete step-by-step answer:
Given:
AB and CD are parallel lines (AB II CD) intersected by a transversal PQ at L and M respectively.
[ Transversal – A line that intersects two lines on the same plane intersecting at two different points.]
To find :
In this figure,
[ Anterior internal angles are a pair of angles on the inner side of each side of each of those two lines but on the opposite sides of the transversal]
We know that anterior interior angles are equal when two lines are parallel & there is a transversal.
AB and CD are parallel lines, AB II CD & PQ is transversal.
Hence, the value of
Now to find value of
We need to know that, Sum of all the angles on a straight line is
In the above figure, on line segment LM,
Hence the value of
Note: Alternate interior angles are equal, when lines are parallel to each other & there is a transversal so by implementing this concept we can get a linear equation to be solved carefully to get the ultimate answer.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Questions & Answers - Ask your doubts

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Science: Engaging Questions & Answers for Success

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Difference Between Plant Cell and Animal Cell

Given that HCF 306 657 9 find the LCM 306 657 class 9 maths CBSE

The highest mountain peak in India is A Kanchenjunga class 9 social science CBSE

What is pollution? How many types of pollution? Define it

Differentiate between the Western and the Eastern class 9 social science CBSE




