Question

In parallelogram ABCD, the bisector of angle A meets DC in P and \[AB{\text{ }} = {\text{ }}2AD.\] Find \[\angle APB.\]

Answer 1

Hint: To solve this problem, we will first start with constructing a figure, then using basic properties of parallelogram, i.e., opposite angles are supplementary, we will get an equation. After that we will use the given condition, and by applying the values, we will get our required answer.

Complete step-by-step answer:
We have been given that in the parallelogram ABCD, the bisector of angle A meets DC in P and also, \[\;AB{\text{ }} = {\text{ }}2AD.\] We need to find the \[\angle APB.\]
Let us construct a figure, using the above information to understand better.

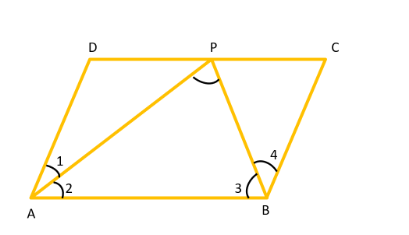
We know that in a parallelogram opposite angles are supplementary.
Therefore, from the figure, \[\angle 1{\text{ }} + {\text{ }}\angle 2{\text{ }} + {\text{ }}\angle 3{\text{ }} + {\text{ }}\angle 4{\text{ }} = {\text{ }}180^\circ .....eq.(1)\]
Now, from the figure we get that, \[\angle 1{\text{ }} = {\text{ }}\angle 2\] , because it is given that AP is the bisector of angle A.
Also, \[\angle 3{\text{ }} = {\text{ }}\angle 4,\] because BP is the bisector of angle B.
So, on putting the values in \[eq.\left( 1 \right),\] we get
\[
\Rightarrow {\angle 2{\text{ }} + {\text{ }}\angle 2{\text{ }} + {\text{ }}\angle 3{\text{ }} + {\text{ }}\angle 3{\text{ }} = {\text{ }}180^\circ } \\
\Rightarrow {2\left( {\angle 2{\text{ }} + {\text{ }}\angle 3} \right){\text{ }} = {\text{ }}180^\circ } \\
\Rightarrow {\angle 2{\text{ }} + {\text{ }}\angle 3{\text{ }} = {\text{ }}90^\circ \ldots .eq.\left( 2 \right)}
\]
We know that, sum of angles in a triangle equals to \[180^\circ .\]
So, in triangle APB.
\[\Rightarrow \angle 2{\text{ }} + {\text{ }}\angle 3{\text{ }} + {\text{ }}\angle APB{\text{ }} = {\text{ }}180^\circ \]
Now from \[eq.\left( 2 \right),\] we get
\[
\Rightarrow {90^\circ {\text{ }} + {\text{ }}\angle APB{\text{ }} = {\text{ }}180^\circ } \\
{\angle APB{\text{ }} = {\text{ }}180^\circ {\text{ }}-{\text{ }}90^\circ {\text{ }} = {\text{ }}90^\circ }
\]
Thus, \[\angle APB\] is \[90^\circ .\]

Note: To solve such questions, students should always construct a figure first. And also, in the solution, we have taken, BP is the bisector of angle B, because, in the parallelogram, we know that opposite sides are equal and parallel, therefore, AD \[ = \] CB, and AB \[ = \] CD. So, since it is given in the question that AP is the bisector of angle A, so we have considered that BP is the bisector of angle B here.