
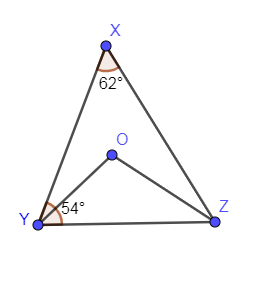
In the below figure,

Answer
495k+ views
Hint: We know that the sum of all the angles of a triangle are
Complete step by step answer:
We have given a figure below, in which
We are asked to find
First of all we are going to find the value of angle Z which we do by using the property of the triangle of XYZ that the sum of all angles of the triangle are
Substituting
Hence, we got the value of angle Z as
It is also given that OY and OZ are the angle bisectors of angles Y and Z respectively. This means that the values of
From the above, we got the value of
From the above, we got the value of
Now, to find the value of
From the above, we got the value of
Hence, we got the value of
Note: This question demands the knowledge of the property of a triangle which says that sum of all angles of the triangle are
The mistake that could happen in this problem is the calculation mistakes so be careful. Another thing through which you can check whether the angles that you calculated are correct or not by using these angles in the equation of sum of all the angles of the triangle as
For e.g. to check whether
Now, substituting the above value of angle Z in eq. (1) we get,
As you can see that L.H.S is equal to R.H.S so the value of
Complete step by step answer:
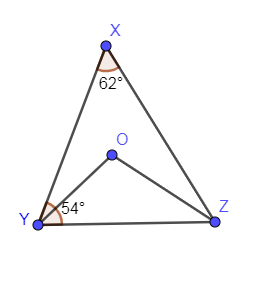
We have given a figure below, in which

We are asked to find
First of all we are going to find the value of angle Z which we do by using the property of the triangle of XYZ that the sum of all angles of the triangle are
Substituting
Hence, we got the value of angle Z as
It is also given that OY and OZ are the angle bisectors of angles Y and Z respectively. This means that the values of
From the above, we got the value of
From the above, we got the value of
Now, to find the value of
From the above, we got the value of
Hence, we got the value of
Note: This question demands the knowledge of the property of a triangle which says that sum of all angles of the triangle are
The mistake that could happen in this problem is the calculation mistakes so be careful. Another thing through which you can check whether the angles that you calculated are correct or not by using these angles in the equation of sum of all the angles of the triangle as
For e.g. to check whether
Now, substituting the above value of angle Z in eq. (1) we get,
As you can see that L.H.S is equal to R.H.S so the value of
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Difference Between Plant Cell and Animal Cell

Given that HCF 306 657 9 find the LCM 306 657 class 9 maths CBSE

The highest mountain peak in India is A Kanchenjunga class 9 social science CBSE

What is the difference between Atleast and Atmost in class 9 maths CBSE

What is pollution? How many types of pollution? Define it





