Question

In the displacement method, a concave lens is placed in between an object and a screen. If the magnification in the two positions are ${m_1}$ and ${m_2}$ (${m_1} > {m_2}$), and the distance between the two positions of the lens is x, the focal length of the lens is
$\begin{gathered}
  {\text{A}}{\text{. }}\dfrac{x}{{{m_1} + {m_2}}} \\
  {\text{B}}{\text{. }}\dfrac{x}{{{m_1} - {m_2}}} \\
  {\text{C}}{\text{. }}\dfrac{x}{{{{\left( {{m_1} + {m_2}} \right)}^2}}} \\
  {\text{D}}{\text{. }}\dfrac{x}{{{{\left( {{m_1} - {m_2}} \right)}^2}}} \\
\end{gathered} $

Answer 1

Hint: We can make use of the relations for magnification for the displacement method. Then by using the lens formula in the obtained expression and solving for the focal length, we shall be able to obtain the required value of focal length.

Complete step by step answer:
We are given that a concave lens is placed in between an object and a screen and we try to find out the focal length of this lens by using the displacement method. The following diagrams show the image formation in the displacement method.

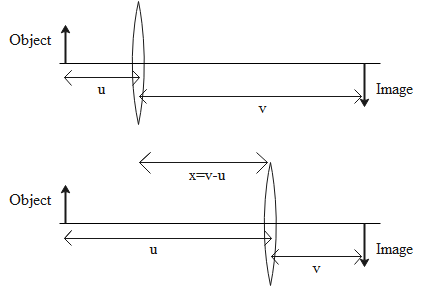
In the displacement method, the two magnifications obtained at two positions are given as follows:
$\begin{gathered}
  {m_1} = \dfrac{v}{u}{\text{ }}...{\text{(i)}} \\
  {m_2} = \dfrac{u}{v}{\text{ }}...{\text{(ii)}} \\
\end{gathered} $
Here u is the distance of the object from the lens while v is the distance of the image from the lens. For these magnifications, the following relation holds.
${m_1}.{m_2} = 1$
Now subtracting equation (ii) from equation (i), we get
${m_1} - {m_2} = \dfrac{v}{u} - \dfrac{u}{v} = \dfrac{{{v^2} - {u^2}}}{{uv}}$
From the diagram we get that $v - u = x$. Using this in above equation, we get
${m_1} - {m_2} = \dfrac{{\left( {v + u} \right)\left( {v - u} \right)}}{{uv}} = \dfrac{{\left( {v + u} \right)x}}{{uv}}{\text{ }}...{\text{(iii)}}$
Now we will use the lens formula, which is given as
$\dfrac{1}{f} = \dfrac{1}{v} - \dfrac{1}{u}$
For the first case, we have
Distance of object from lens $ = - u$
Distance of image from lens $ = v$
Therefore, we have
$\begin{aligned}
& \dfrac{1}{f} = \dfrac{1}{v} - \dfrac{1}{{ - u}} = \dfrac{1}{v} + \dfrac{1}{u} \\
& \dfrac{1}{f} = \dfrac{{u + v}}{{uv}} \\
\end{aligned} $
Using this in equation (iii), we get
$\begin{aligned}
& {m_1} - {m_2} = \dfrac{x}{f} \\
& f = \dfrac{x}{{{m_1} - {m_2}}} \\
\end{aligned} $

So, the correct answer is “Option B”.

Note: 1. We have used the following convention for the lens. All the distances to the left side of a lens are taken as negative while all the distances to the right hand side of the lens are taken as positive.
2. The focal length of a concave lens is negative while the focal length of a convex lens is positive.