Question

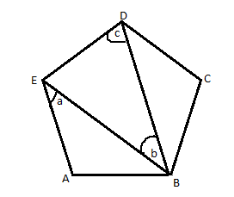
In the figure, ABCDE is a regular pentagon. Find the relation between a, b and c.

Answer 1

Hint- In order to find the value of a, b and c, we will use the property as all inner angles of a regular pentagon is ${108^0}$ and by using the data of the given figure we will proceed further.

Complete step-by-step answer:

As we know that, all inner angles of a regular pentagon is ${108^0}$
Therefore $\angle B = {108^0}$
The pentagon is divided into 3 equal parts and the angles also
Therefore the value of angle b is
$b = \dfrac{{{{108}^0}}}{3} = {36^0}{\text{ [}}\because \angle {\text{B = 3b]}}$
$\Delta EBD$ is an isosceles triangle and sum of all angles of and sum of all angles of triangle is ${180^0}$
Therefore
$b + c + \angle DEB = {180^0}$
As we know that in isosceles triangle the angles opposite to equal sides are equal
Therefore angle $c = \angle DEB$
$
   \Rightarrow b + c + c = {180^0} \\
   \Rightarrow 2c + b = {180^0} \\
$
Substituting the value of b in the above equation
$
   \Rightarrow 2c + {36^0} = {180^0} \\
   \Rightarrow 2c = {180^0} - {36^0} \\
   \Rightarrow c = \dfrac{{{{144}^0}}}{2} = {72^0} \\
 $
As we know that alternate angles are equal and
Here a and b are alternate angles therefore
$
  a = b \\
  a = {36^0} \\
$
Hence, angles a, b and c are ${36^0},{36^0}and{\text{ }}{72^0}$ respectively.

Note- In order to solve these types of questions, remember the properties of triangles, squares, pentagons etc. Also remember the properties of angles and parallel lines such as alternate angles, corresponding angles, vertically opposite angles and about transverse lines and more. Draw the figure first and try to solve for a single angle first and then proceed further.