Question

In the given figure AB is parallel to CD. Find the value of x.

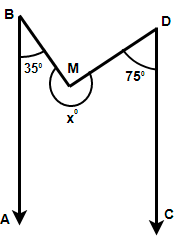
Answer 1

Hint: Draw a line MN parallel to AB and CD and divide angle x into two parts, name them as angle p and q. Now, consider the parallel line AB and MN, using the fact that the sum of co interior angles is 180 degrees from a relation between angle p and 35 degrees and solve for the value of p. Similarly, considering the parallel lines MN and CD, using the same fact of co interior angles form a relation between angle q and 75 degrees, solve for the value of q. Finally take the sum of p and q to get the value of x.

Complete step by step solution:
Here we have been provided with a figure shown below and we are asked to find the values of x.

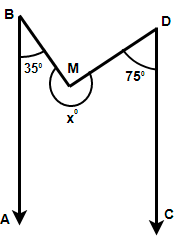
The above question is of the topic parallel lines and the transversal. Now, to solve this question we need to construct a line MN parallel to the lines AB and CD, so we get the figure as shown below.

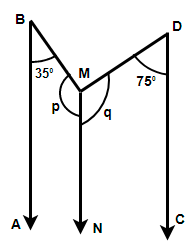
In the above figure we can see that angle x gets divided into two parts so we have named the first part as p and the second part as q. Let us consider the two parts separately one by one.
(1) Considering the parallel lines MN and AB we can see that angles BMN and ABM are co interior angles and we know that the sum of co interior angles is equal to 180 degrees. So we get,
$\begin{align}
  & \Rightarrow \angle BMN+\angle ABM={{180}^{\circ }} \\
 & \Rightarrow p+{{35}^{\circ }}={{180}^{\circ }} \\
 & \Rightarrow p={{180}^{\circ }}-{{35}^{\circ }} \\
 & \Rightarrow p={{145}^{\circ }} \\
\end{align}$
(1) Considering the parallel lines MN and CD. Here we have the angles CDM and DMN as the pair of co interior angles. So using the same fact of pair of co interior angles we get,
$\begin{align}
  & \Rightarrow \angle DMN+\angle CDM={{180}^{\circ }} \\
 & \Rightarrow q+{{75}^{\circ }}={{180}^{\circ }} \\
 & \Rightarrow q={{180}^{\circ }}-{{75}^{\circ }} \\
 & \Rightarrow q={{105}^{\circ }} \\
\end{align}$
Now, since angle x was split into angles p and q so the value of x will be equal to the sum of the value of these two angles obtained. So we have,
$\begin{align}
  & \Rightarrow x=p+q \\
 & \Rightarrow x={{145}^{\circ }}+{{105}^{\circ }} \\
 & \therefore x={{250}^{\circ }} \\
\end{align}$
Hence the measure of angle x is 250 degrees.

Note: Note that you do not have to use the protractor to measure the value of angle x. This is because it may be possible that the value of other angles is not according to the slope and given randomly. In such cases you may get a different value according to the angles given in the question and as measured from the protractor. So the answer obtained using the protractor will be wrong.