
Is parallelogram a rectangle? Can you call a rectangle a parallelogram?
Answer
488.4k+ views
Hint: From the given question, we have to discuss only the geometrical shapes of a rectangle and a parallelogram. First, we have to discuss some properties of a rectangle and properties of a parallelogram.
Complete step-by-step answer:
For the result, first we have to discuss some properties of a rectangle and properties of a parallelogram.
A rectangle is a quadrilateral with four right angles. It can also be defined as an equiangular quadrilateral, since equiangular means that all of its angles are equal
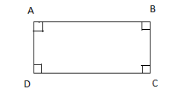
Properties of a rectangle are given below:
A parallelogram is a quadrilateral with both pairs of opposite sides parallel. In a quadrilateral, opposite sides do not share a vertex and opposite angles do not share a side.
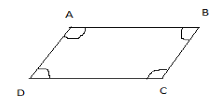
Properties of a parallelogram are given below:
From the given question, now we are going to discuss only the geometrical shapes of a rectangle and a parallelogram.
From the above discussed properties of a rectangle and properties of a parallelogram, we are going to conclude the required first result in the following manner.
The main characteristic of a rectangle is that the opposite sides must be equal and all the angles must be
Now, we are going to conclude the second result in the following manner.
The main characteristic of a parallelogram is that the opposite sides must be parallel to each other. In a rectangle the opposite sides are parallel to one another.
Therefore, we have to conclude the required result.
Yes, every parallelogram cannot be a rectangle but every rectangle is a parallelogram.
Note: A square is similar to a rectangle. Square has 4 sides and 2 internal diagonals with internal angles equal to 90. The only difference between square and rectangle is that, in a square all the sides are equal but in a rectangle only the opposite sides are equal.
Complete step-by-step answer:
For the result, first we have to discuss some properties of a rectangle and properties of a parallelogram.
A rectangle is a quadrilateral with four right angles. It can also be defined as an equiangular quadrilateral, since equiangular means that all of its angles are equal

Properties of a rectangle are given below:
A parallelogram is a quadrilateral with both pairs of opposite sides parallel. In a quadrilateral, opposite sides do not share a vertex and opposite angles do not share a side.

Properties of a parallelogram are given below:
From the given question, now we are going to discuss only the geometrical shapes of a rectangle and a parallelogram.
From the above discussed properties of a rectangle and properties of a parallelogram, we are going to conclude the required first result in the following manner.
The main characteristic of a rectangle is that the opposite sides must be equal and all the angles must be
Now, we are going to conclude the second result in the following manner.
The main characteristic of a parallelogram is that the opposite sides must be parallel to each other. In a rectangle the opposite sides are parallel to one another.
Therefore, we have to conclude the required result.
Yes, every parallelogram cannot be a rectangle but every rectangle is a parallelogram.
Note: A square is similar to a rectangle. Square has 4 sides and 2 internal diagonals with internal angles equal to 90. The only difference between square and rectangle is that, in a square all the sides are equal but in a rectangle only the opposite sides are equal.
Latest Vedantu courses for you
Grade 9 | CBSE | SCHOOL | English
Vedantu 9 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
Recently Updated Pages
Express the following as a fraction and simplify a class 7 maths CBSE

The length and width of a rectangle are in ratio of class 7 maths CBSE

The ratio of the income to the expenditure of a family class 7 maths CBSE

How do you write 025 million in scientific notatio class 7 maths CBSE

How do you convert 295 meters per second to kilometers class 7 maths CBSE

Write the following in Roman numerals 25819 class 7 maths CBSE

Trending doubts
Full Form of IASDMIPSIFSIRSPOLICE class 7 social science CBSE

What does R mean in math class 7 maths CBSE

How many crores make 10 million class 7 maths CBSE

Fill in the blanks with appropriate modals a Drivers class 7 english CBSE

The southernmost point of the Indian mainland is known class 7 social studies CBSE

Convert 200 Million dollars in rupees class 7 maths CBSE





