Question

It is required to make a closed cylindrical tank of height $1m$ and base diameter $140cm$ from a metal sheet. How many square meters of the sheet are required for the same? Assume $\pi = 22/7$

Answer 1

Hint: The cylinder is a closed cylinder so, we need to find the total surface area of the cylinder which is the same as the area of metal sheet required to make that cylinder. The total surface area of a cylinder is the sum of areas of two circular lateral surfaces and the curved surface. In numerical form, total surface area of the cylinder = $2\pi rh + 2\pi {r^2} = 2\pi r(r + h)$ where h is the height of the cylinder and r is the radius of the lateral surface of the cylinder.

Complete step by step solution:

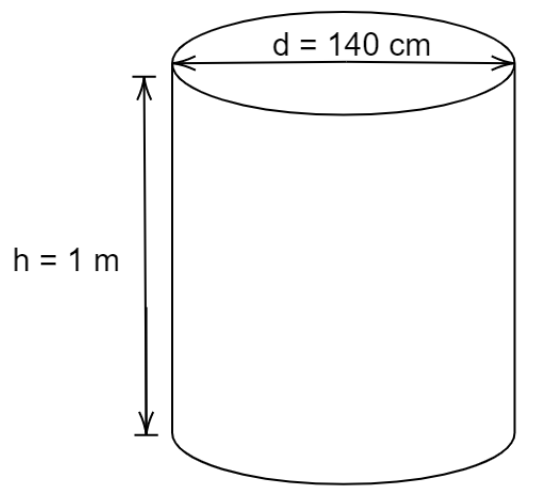
The height of the closed cylindrical tank is $h = 1m$.
The diameter of the base of the cylindrical tank is $d = 140cm$.
$\therefore $The radius of the base will be half of the diameter.
$\therefore $the radius = $r = \dfrac{d}{2} = \dfrac{{140}}{2} = 70cm$
Converting the radius from cm to m,
We know, $1m = 100cm$,
$\therefore $$70cm = \dfrac{{70}}{{100}}m = 0.7m$
The radius of the base is $0.7m$.
Now, the area of metal sheet required is the total surface area of the cylinder.
The formula for total surface area of cylinder is $TSA = 2\pi r(r + h)$
Area of metal sheet required
= total surface area of cylinder
= $2\pi r(r + h)$
Substituting the value of radius and height in the above formula,
= $2 \times \dfrac{{22}}{7} \times 0.7 \times (0.7 + 1)$
Adding the terms inside the bracket,
= $2 \times \dfrac{{22}}{7} \times 0.7 \times 1.7$
We can write $0.7$ and $1.7$ in decimal form,
= $2 \times \dfrac{{22}}{7} \times \dfrac{7}{{10}} \times \dfrac{{17}}{{10}}$
Cancelling $7$ from numerator and denominator,
= $2 \times \dfrac{{22}}{1} \times \dfrac{1}{{10}} \times \dfrac{{17}}{{10}}$
Multiplying the numerator terms and denominator terms,
= $\dfrac{{2 \times 22 \times 1 \times 17}}{{10 \times 10}}$
Multiplying the terms,
= $\dfrac{{748}}{{100}}$
Dividing the terms,
= $74.8{m^2}$
Therefore, the area of metal sheet required is $74.8{m^2}$.

Note: While solving the mensuration problems, draw diagrams for the ease of understanding and write all the given values first so that it becomes easy to substitute all the values without any mistake. The calculations should be accurate because if any one value gets wrong then the whole problem gets wrong.