
When the length of each side of a cube is increased by 3 cm, its volume is increased by
A.
B.
C.
D.
Answer
526.2k+ views
Hint: The given problem is related to absolute change. Consider the length of each side as x cm. Calculate its volume in terms of x. On increasing the length by 3 cm, it becomes (x+3) cm. Calculate the volume in terms of x. The difference in volume is given as
Complete Step-by-step answer:
We will consider the length of the side of the cube as x cm. We know, the volume of a cube of side a cm is given as
This cube can be visualized as shown:
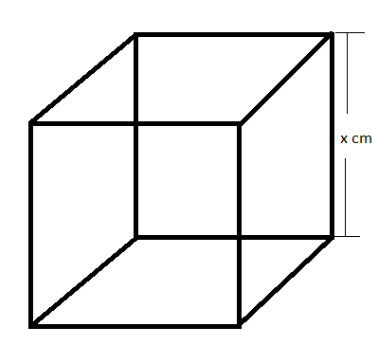
Now, it is given that the length of each side is increased by 3 cm. So, the new length of each side of the cube becomes (x + 3) cm. So, the new volume of the cube is
This cube can be visualized as shown:
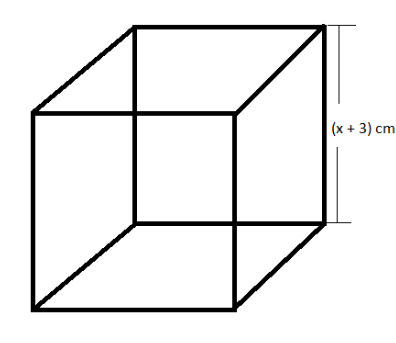
Now, we know, the expansion of
The above equation is quadratic in x. We can solve it by splitting the middle term. We have to split 3x into two terms such that their sum is equal to 3x and their product is equal to
So, either x = 15 or x = -18. We know, x is the length of the side of the cube. So, it cannot be negative. So, x = 15 cm. So, the original volume of the cube is equal to
Now, on decreasing the length of the side by 20%, the resulting side becomes (15 – 20% of 15) cm. So, the resulting side is equal to 15 – 3 = 12 cm. So, the resultant volume becomes
Hence, option B. is the correct option.
Note: Be careful while doing calculations. The problem contains the use of identities which should be remembered properly, as getting them wrong can lead to wrong answers. Also, the absolute change in the value of an entity is calculated as (final value – initial value) and not (initial value – final value).
Complete Step-by-step answer:
We will consider the length of the side of the cube as x cm. We know, the volume of a cube of side a cm is given as
This cube can be visualized as shown:

Now, it is given that the length of each side is increased by 3 cm. So, the new length of each side of the cube becomes (x + 3) cm. So, the new volume of the cube is
This cube can be visualized as shown:

Now, we know, the expansion of
The above equation is quadratic in x. We can solve it by splitting the middle term. We have to split 3x into two terms such that their sum is equal to 3x and their product is equal to
So, either x = 15 or x = -18. We know, x is the length of the side of the cube. So, it cannot be negative. So, x = 15 cm. So, the original volume of the cube is equal to
Now, on decreasing the length of the side by 20%, the resulting side becomes (15 – 20% of 15) cm. So, the resulting side is equal to 15 – 3 = 12 cm. So, the resultant volume becomes
Hence, option B. is the correct option.
Note: Be careful while doing calculations. The problem contains the use of identities which should be remembered properly, as getting them wrong can lead to wrong answers. Also, the absolute change in the value of an entity is calculated as (final value – initial value) and not (initial value – final value).
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Questions & Answers - Ask your doubts

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Science: Engaging Questions & Answers for Success

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Difference Between Plant Cell and Animal Cell

Given that HCF 306 657 9 find the LCM 306 657 class 9 maths CBSE

The highest mountain peak in India is A Kanchenjunga class 9 social science CBSE

What is pollution? How many types of pollution? Define it

Differentiate between the Western and the Eastern class 9 social science CBSE




