Question

Locate $\sqrt{10}$ on the number line.

Answer 1

Hint: In this question we will use the methods of representing a number on number line. Every real number is represented by a unique point on the number line. Also every point on the number line represents a unique real number. We will locate a number on the number line by using Pythagoras theorem.

Complete step-by-step solution:
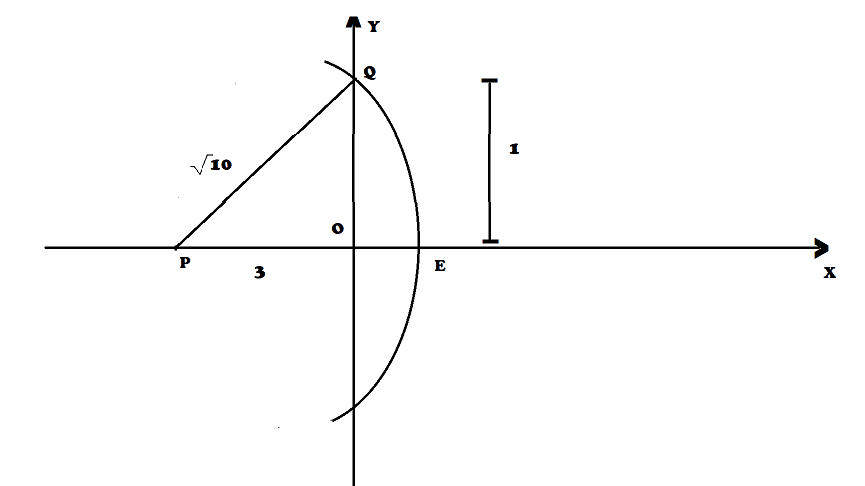
Here , we have to locate $\sqrt{10}$ on number line,
We will do this by using the Pythagoras theorem.
Here, we can write $\sqrt{10}$ as:
$\Rightarrow \sqrt{10}=\sqrt{9+1}$
$\Rightarrow \sqrt{10}=\sqrt{{(3)}^2+{(1)}^2}$ …….(i)
We know that , according to Pythagoras theorem ,
$\Rightarrow$ (hypotenuse) = $\sqrt{{(base)}^2+{(perpendicular)}^2}$.
By comparing this with equation (i) ,we get
Hypotenuse = $\sqrt{10}$ , perpendicular = 1 and base = 3.
Now, according to this, the steps of construction are as follows :
Step 1. : Take a line segment PO =3 units on the x –axis. (consider 1 unit =2 cm) .
Step 2: Draw a perpendicular on O and draw a line OQ = 1 unit.
Step 3: Now join PQ with $\sqrt{10}$.
Step 4: Take P as centre and PQ as radius, draw an arc which cuts the x- axis at point E.
Step 5: Now the line segment PQ in the figure represents $\sqrt{10}$ units .
Hence, through these steps we had located $\sqrt{10}$ on the number line.

Note: In this type of question we should remember some basic points like we should know Pythagoras theorem and basic knowledge of number line and then by using the Pythagoras theorem we will find out the things like hypotenuse, perpendicular and base. Hence by doing step by step construction, we will locate the given number on the number line.