Question

Make a diagram to show how hypermetropia is corrected. The near point of the hypermetropic eye is $1m$. What is the power of the lens required to correct this defect? Assume that the near point of eye is $25cm$.

Answer 1

Hint: In case of hypermetropia, the image of the object placed at normal near point is formed at the back of the retina. For correcting hypermetropia, the lens which can converge the light rays at the retina of the human eye will be considered. For finding the power of the lens used, we need to find the focal length of the lens used.

Formula used:
For finding the focal length of lens, $\dfrac{1}{f}=\dfrac{1}{v}-\dfrac{1}{u}$
For finding the power of lens, $P=\dfrac{1}{f}$

Complete step-by-step answer:
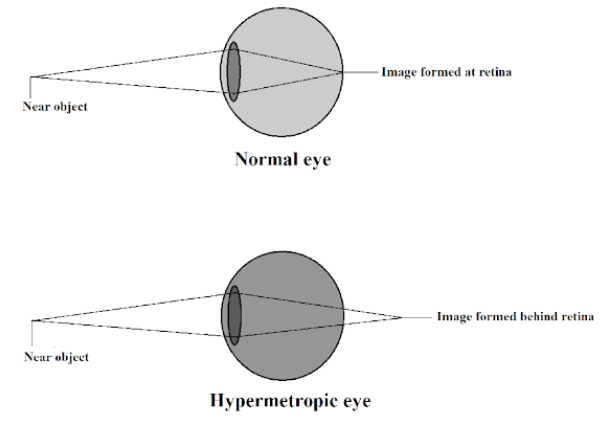
Hypermetropia is also known as farsightedness. A person with hypermetropia can see distant objects clearly but cannot see the near objects distinctly.
This effect can be corrected by using a convex lens of appropriate power. The convex lens converges the incident light rays so that the final image of the object from the human eye lens is formed at the retina of the eye.

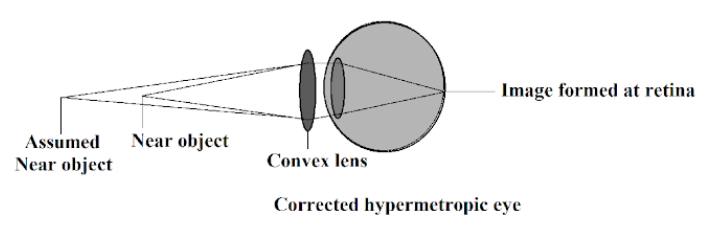
The object placed at $25cm$ from the correcting lens must produce a virtual image at $1m$ or $100cm$.
Object distance, $u=-25cm$
Virtual image distance, $v=-100cm$
Using lens formula,
$\dfrac{1}{f}=\dfrac{1}{v}-\dfrac{1}{u}$
Putting the values, we get,
$\begin{align}
  & \dfrac{1}{f}=\dfrac{1}{-100}-\dfrac{1}{-25} \\
 & =\dfrac{1}{-100}+\dfrac{1}{25} \\
 & =+\dfrac{3}{100}
\end{align}$
Therefore,
$\begin{align}
  & f=+\dfrac{100}{3}cm \\
 & f=+\dfrac{1}{3}m \\
\end{align}$
Therefore, power of lens is,
$\begin{align}
  & P=\dfrac{1}{f}=+3 \\
 & P=+3D \\
\end{align}$
Hence, the power of the lens required to correct the defect is $+3D$.

Note: While calculating the power of the lens in Dioptres, focal length must be taken in metres only. For correcting hypermetropia, convex lens is used and focal length of convex lens is considered as positive. Therefore, the power of the lens used for correcting hypermetropia is positive.