
What do you mean by a linear pair of angles?
Answer
516k+ views
Hint: We will first understand the arm and vertex arms and vertex of an angle. Then, we will understand the conditions of linear pairs of angles and put them together to form it.
Complete step-by-step answer:
Angle is a geometric figure which has two rays known as arms of angle meeting at the same endpoint known as vertex of an angle.
Now, a linear pair of angles are two angles which are placed together with four conditions.
First condition is that both the angles should have a common vertex. That is, there will be only one vertex for both the angles.
Second condition is that they should have a common arm. This means that one of the arms of both the angles should be shared by both the angles. That is, they should together have three arms.
Third condition is that the interior part of angles should not overlap. That is, one angle should not cross the other. Therefore, both the angles should be on the opposite side of the common arm.
Fourth condition is that the sum of both the angles should be
Hence the linear pair of angles can be drawn as:
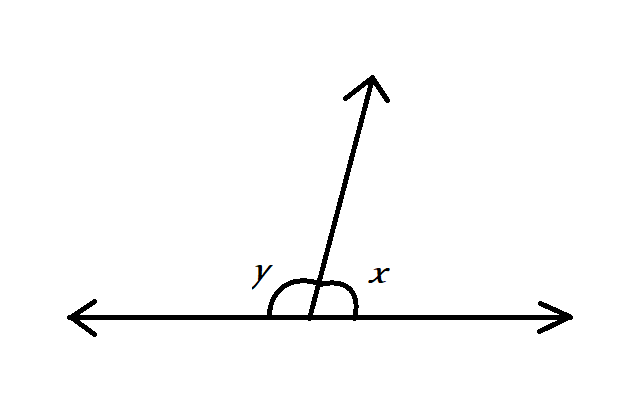
Here angle
Note: Alternative way to understand that, when two straight lines interest, then the pair of angles between them on the upper side or lower side of one of the lines is a linear pair of angels.
Complete step-by-step answer:
Angle is a geometric figure which has two rays known as arms of angle meeting at the same endpoint known as vertex of an angle.
Now, a linear pair of angles are two angles which are placed together with four conditions.
First condition is that both the angles should have a common vertex. That is, there will be only one vertex for both the angles.
Second condition is that they should have a common arm. This means that one of the arms of both the angles should be shared by both the angles. That is, they should together have three arms.
Third condition is that the interior part of angles should not overlap. That is, one angle should not cross the other. Therefore, both the angles should be on the opposite side of the common arm.
Fourth condition is that the sum of both the angles should be
Hence the linear pair of angles can be drawn as:

Here angle
Note: Alternative way to understand that, when two straight lines interest, then the pair of angles between them on the upper side or lower side of one of the lines is a linear pair of angels.
Recently Updated Pages
Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 4 Maths: Engaging Questions & Answers for Success

Master Class 4 English: Engaging Questions & Answers for Success

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Difference Between Plant Cell and Animal Cell

Given that HCF 306 657 9 find the LCM 306 657 class 9 maths CBSE

The highest mountain peak in India is A Kanchenjunga class 9 social science CBSE

What is the difference between Atleast and Atmost in class 9 maths CBSE

What is pollution? How many types of pollution? Define it





