
What is the measure of each exterior angle of a regular 15-sided polygon?
Answer
427.5k+ views
Hint: Here in this question, we have to find each exterior angle of a regular 15-sided polygon. To solve this, remember the sum of the measures of the external angles of any polygon is
Complete step-by-step answer:
Regular polygon is a polygon in which all angles are equal in measure (equiangular) and all sides have the same length (equilateral). Regular polygons either be convex or Star. The Exterior Angle is the angle between any side of a geometrical shape, and a line extended from the next side.
(In other words, when we add interior angle and exterior angle we get straight line that is
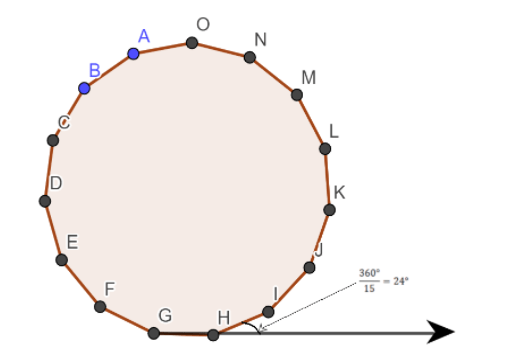
If a 15-sided polygon is regular, then all the sides are equal in length, and fifteen angles are of equal measures
A 15-sided regular polygon is also called as a “pentadecagon” or “pentakaidecagon” or “15-gon”
As we know the sum of the measures of the external angles of any polygon is
If the polygon has
For 15-sided regular polygon, number of sides
Hence, The measure of each exterior angle of a regular 15-sided polygon is
So, the correct answer is “
Note: If any regular polygon given its number of sides we can solve by above methods but if the polygon given its interior angle then we need to use each exterior angle =
Complete step-by-step answer:
Regular polygon is a polygon in which all angles are equal in measure (equiangular) and all sides have the same length (equilateral). Regular polygons either be convex or Star. The Exterior Angle is the angle between any side of a geometrical shape, and a line extended from the next side.
(In other words, when we add interior angle and exterior angle we get straight line that is

If a 15-sided polygon is regular, then all the sides are equal in length, and fifteen angles are of equal measures
A 15-sided regular polygon is also called as a “pentadecagon” or “pentakaidecagon” or “15-gon”
As we know the sum of the measures of the external angles of any polygon is
If the polygon has
For 15-sided regular polygon, number of sides
Hence, The measure of each exterior angle of a regular 15-sided polygon is
So, the correct answer is “
Note: If any regular polygon given its number of sides we can solve by above methods but if the polygon given its interior angle then we need to use each exterior angle =
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Difference Between Plant Cell and Animal Cell

Given that HCF 306 657 9 find the LCM 306 657 class 9 maths CBSE

The highest mountain peak in India is A Kanchenjunga class 9 social science CBSE

What is the difference between Atleast and Atmost in class 9 maths CBSE

What is pollution? How many types of pollution? Define it




