
What is the measure of one angle of a regular 12 – sided polygon?
Answer
441k+ views
Hint: To find the measure of one angle of a regular 12 – sided polygon, first of all we will find the central angle which is constructed by joining two consecutive vertices of the polygon with the centre. We will find this angle by dividing
Complete step by step answer:
We have given a regular 12 – sided polygon which we have constructed in the below:
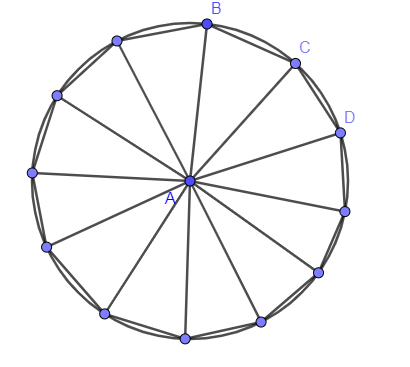
Now, we are going to find the internal angle which is formed by joining the two consecutive vertices with the centre which we can see in the above figure,
The numerator and the denominator of the above fraction will get divided by 12 and we get,
Now, in
Let us assume the above two angles as x and we are showing this angle in the above figure and we get,

Now, we know that sum of all the angles of a triangle is
Subtracting
Now, as the given polygon is a regular polygon so all the neighboring angles are also equal so the above figure will look as:
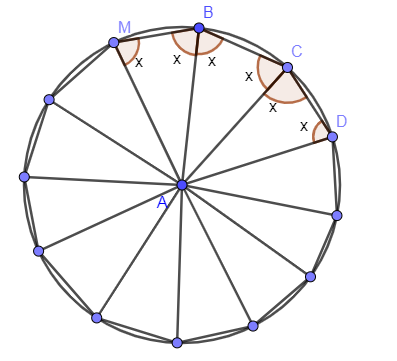
From the figure, you can see that
Hence, the measure of one angle of a regular 12 – sided polygon is equal to
Note: The point to be noted is that this is the regular polygon so all the 12 sides and the 12 angles are equal to this polygon. From this problem, we have learnt that the measure of any one angle of a regular 12 – sided polygon is
Complete step by step answer:
We have given a regular 12 – sided polygon which we have constructed in the below:

Now, we are going to find the internal angle which is formed by joining the two consecutive vertices with the centre which we can see in the above figure,
The numerator and the denominator of the above fraction will get divided by 12 and we get,
Now, in
Let us assume the above two angles as x and we are showing this angle in the above figure and we get,

Now, we know that sum of all the angles of a triangle is
Subtracting
Now, as the given polygon is a regular polygon so all the neighboring angles are also equal so the above figure will look as:

From the figure, you can see that
Hence, the measure of one angle of a regular 12 – sided polygon is equal to
Note: The point to be noted is that this is the regular polygon so all the 12 sides and the 12 angles are equal to this polygon. From this problem, we have learnt that the measure of any one angle of a regular 12 – sided polygon is
Recently Updated Pages
Express the following as a fraction and simplify a class 7 maths CBSE

The length and width of a rectangle are in ratio of class 7 maths CBSE

The ratio of the income to the expenditure of a family class 7 maths CBSE

How do you write 025 million in scientific notatio class 7 maths CBSE

How do you convert 295 meters per second to kilometers class 7 maths CBSE

Write the following in Roman numerals 25819 class 7 maths CBSE

Trending doubts
A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

What are the public facilities provided by the government? Also explain each facility

Difference between mass and weight class 10 physics CBSE

Statistics in singular sense includes A Collection class 10 maths CBSE

Change the following sentences into negative and interrogative class 10 english CBSE




