Question

One angle of an isosceles obtuse-angled triangle is \[{35^ \circ }\]. Find the measure of its obtuse angle in degrees.

Answer 1

Hint: Here in this question, we have to find the obtuse angle of an isosceles triangle as we know the isosceles triangle has two sides of equal length and it angles also same, then by the sum of internal angles of triangle is \[{180^ \circ }\] on substitute the value of two angles and by further simplification we get the third angle which is obtuse.

Complete step by step solution:
A triangle with two sides of equal length is an isosceles triangle. The two equal sides of an isosceles triangle are known as ‘legs’ whereas the third or unequal side is known as the ‘base’.
Consider a triangle \[\Delta \,ABC\] is a isosceles obtuse- angled triangle angle $\angle A$ is a obtuse angle, in triangle the side AB=AC by the given data one of the angle take $\angle B = 35^\circ$.

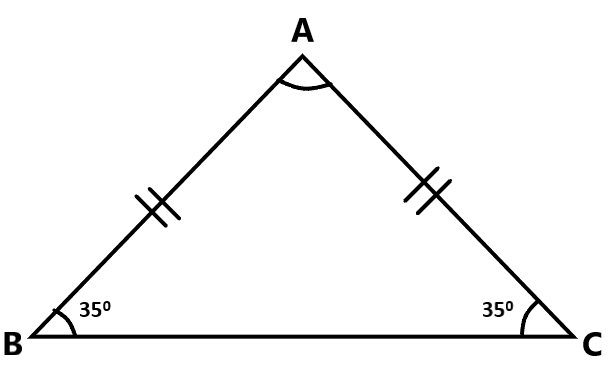
Then by one of the properties of a triangle i.e., “Angles opposite to equal sides in an isosceles triangle are always of equal measure”. In the given isosceles triangle, if $AB = AC$ then $\angle B = \angle C = 35^\circ$.
Now we have to find the obtuse angle i.e., $\angle A=?$
As we know the, sum of internal angles of triangle is \[{180^ \circ }\], then
$\angle A + \angle B + \angle C =180^\circ$
On substituting the corresponding angles, we get
$\Rightarrow \angle A + 35^\circ + 35^\circ =180^\circ $
On simplification,
$\Rightarrow \angle A + 70^\circ =180^\circ $
Subtract \[{70^ \circ }\] on both sides, we get
$\Rightarrow \angle A + 70^\circ - 70^\circ =180^\circ - 70^\circ $

$\Rightarrow \angle A = 110^\circ$
Hence, the measure of each angle of a given isosceles obtuse-angled triangle is \[{35^ \circ }\], \[{35^ \circ }\] and \[{110^ \circ }\].
Therefore, the measure of the obtuse angle in the triangle is $110^\circ$.

Note:
While solving these types of questions, we have to know some basic definitions, properties, axioms and postulates of triangles like if any triangle having two sides are equal whose opposite angles with respect to the lines are equal. And remember the sum of internal angles of triangles always should be equal to \[{180^ \circ }\].