
What is the pascal triangle upto
Answer
430.8k+ views
Hint: One of the most interesting Number Patterns in Pascal's Triangle (named after Blaise Pascal, a famous French Mathematician and Philosopher). To build the triangle, start with "
Complete step by step solution:
The rows of Pascal's triangle are numbered starting with row
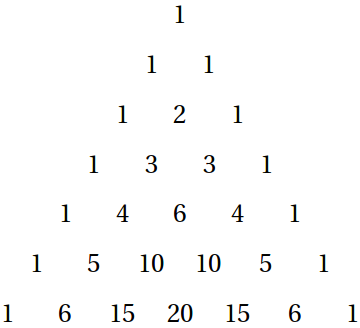
The formula to find the entry of an element in the nth row and
The elements of the following rows and columns can be found using the formula given below.
Here,
The above notation can be written as:
Pascal’s Triangle Binomial Expansion: Pascal’s triangle defines the coefficients which appear in binomial expansions. That means the nth row of Pascal’s triangle comprises the coefficients of the expanded expression of the polynomial
The expansion of
Hence the
It can be expanded as follows:
Note:
1) Pascal's Triangle can show the probability of any combination. For example, if you toss a coin three times, there is only one combination that will give you three heads (HHH), but there are three that will give two heads and one tail (HHT, HTH, THH), also three that give one head and two tails (HTT, THT, TTH) and one for all Tails (TTT). This is the pattern.
2By adding the numbers in the diagonals of the Pascal triangle the Fibonacci sequence can be obtained.
Complete step by step solution:
The rows of Pascal's triangle are numbered starting with row

The formula to find the entry of an element in the nth row and
The elements of the following rows and columns can be found using the formula given below.
Here,
The above notation can be written as:
Pascal’s Triangle Binomial Expansion: Pascal’s triangle defines the coefficients which appear in binomial expansions. That means the nth row of Pascal’s triangle comprises the coefficients of the expanded expression of the polynomial
The expansion of
Hence the
It can be expanded as follows:
Note:
1) Pascal's Triangle can show the probability of any combination. For example, if you toss a coin three times, there is only one combination that will give you three heads (HHH), but there are three that will give two heads and one tail (HHT, HTH, THH), also three that give one head and two tails (HTT, THT, TTH) and one for all Tails (TTT). This is the pattern.
2By adding the numbers in the diagonals of the Pascal triangle the Fibonacci sequence can be obtained.
Recently Updated Pages
Express the following as a fraction and simplify a class 7 maths CBSE

The length and width of a rectangle are in ratio of class 7 maths CBSE

The ratio of the income to the expenditure of a family class 7 maths CBSE

How do you write 025 million in scientific notatio class 7 maths CBSE

How do you convert 295 meters per second to kilometers class 7 maths CBSE

Write the following in Roman numerals 25819 class 7 maths CBSE

Trending doubts
A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Difference between mass and weight class 10 physics CBSE

SI unit of electrical energy is A Joule B Kilowatt class 10 physics CBSE

Statistics in singular sense includes A Collection class 10 maths CBSE

Identify the phrase s in the following sentence The class 10 english CBSE

Why is monsoon considered a unifying bond class 10 social science CBSE




