
Place A and B are 100km apart on a highway. One car starts from A and another from B at the same time. If the cars travel in the same direction at different speeds, they meet in 5 hours. If they travel towards each other, they meet in 1 hour. What are the speeds of two cars?
Answer
527.7k+ views
Hint: First of all, consider the speed of Car A and Car B as SA and SB respectively. When they are moving in the same direction, use (SA – SB)
Complete step-by-step answer:
When they are moving in opposite directions, use (SA + SB)
We are given that place A and B are 100km apart. Also A and B starts at same time from positions A and B respectively. When they move in the same direction, they meet in 5 hours and when they move in opposite directions, that is, towards each other, they meet in 1 hour. We have to find the speeds of Car A and Car B.
Let us diagrammatically consider the situation,
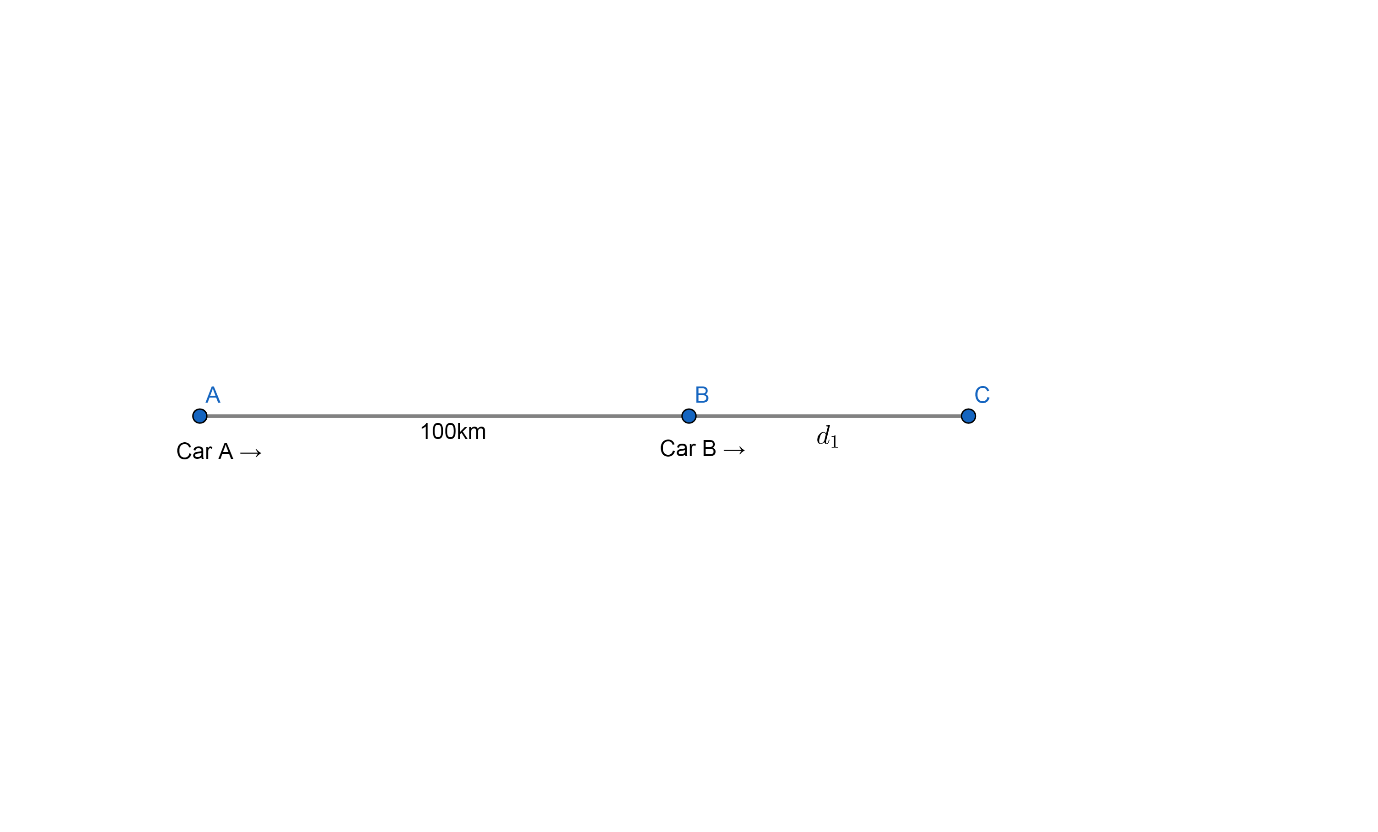
Case I: When both cars are moving in the same direction.
Let us consider that, these cars meet at point C.
Let BC=
Let the speed of Car A is SA and speed of Car B is SB.
In this case, we are given that Car A and Car B meet after 5 hours. Since they meet at C, therefore this means that Car A takes 5 hours to reach C and Car B also takes 5 hours to reach C. Hence we get,
Time taken by car A to reach C = Time taken by Car B to reach C = 5 hours
As we know that time taken
Therefore, we get time taken by Car A to reach C
By putting the value of distance travelled = AB +BC.
Speed of Car A = SA and time taken = 5 hours, we get,
Also, we get time taken by Car B to reach C
By putting the value of distance travelled =
Speed of Car B = SB and time taken = 5 hours, we get,
By cross multiplying above equation, we get,
By putting the value of
By cross multiplying above equation, we get,
By taking out 5 common in above equation, we get,
SA – SB = 20 …………..(2)
Case II: When cars are moving towards each other or in opposite directions.
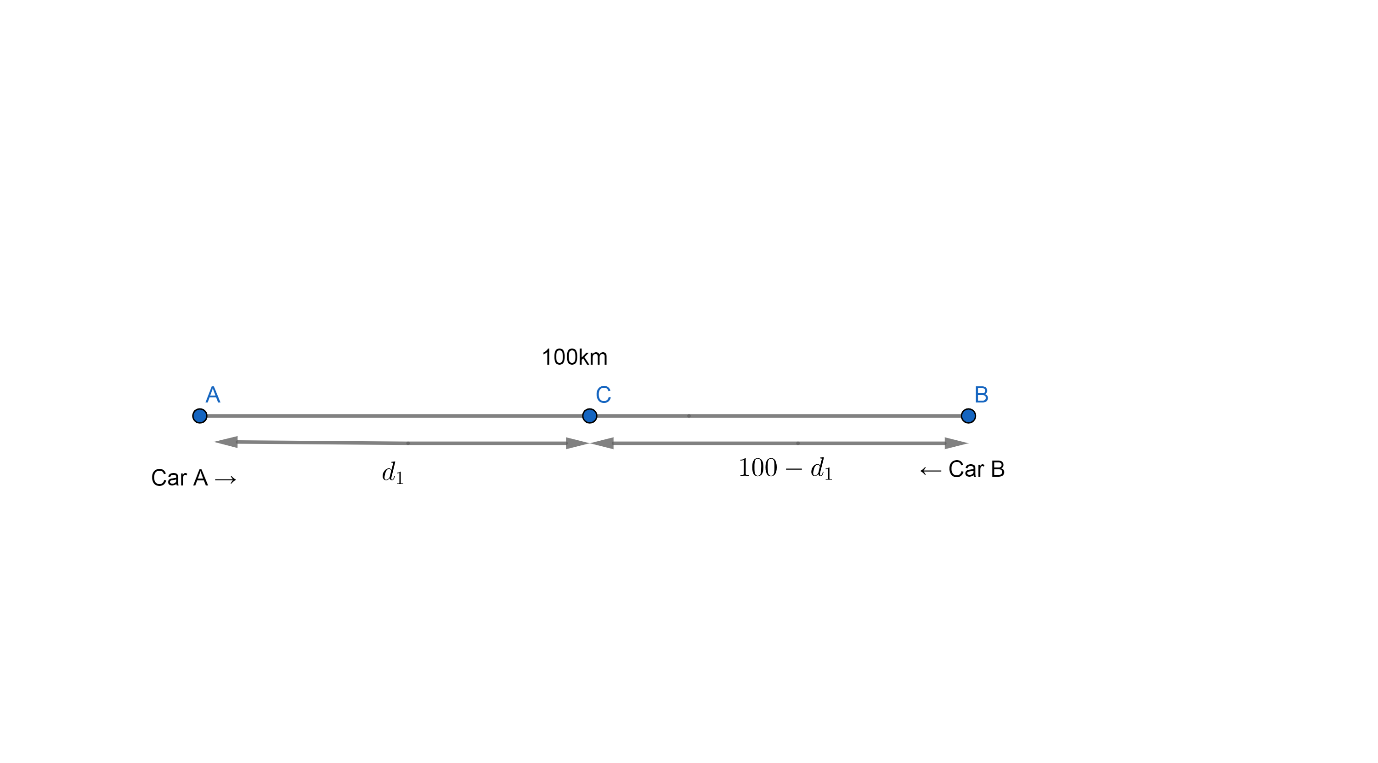
Let us consider that these cars meet at C.
Let AC =
Since AC + CB = 100km
Therefore, CB = 100 -
In this case, we are given that Car A and Car B meet after 1 hour
Since they meet at C, therefore this means that Car A takes 1 hour to reach C and Car B also takes 1 hour to reach C. Hence we get,
Time taken by Car A to reach C = Time taken by Car B to reach C = 1 hour
As we know that time taken
Therefore, we get time taken by Car A to reach C
By putting the value of distance travelled = AC =
Speed of Car A = SA and time taken = 1 hour, we get,
By cross multiplying above equation, we get,
Also, we get time taken by Car B to reach C
By putting the value of distance travelled = BC =
Speed of Car B = SB and time taken = 1 hour, we get,
By cross multiplying above equation, we get,
By putting the value of
(100 – SA) = SB
Or (SA + SB) = 100……………… (4)
By adding equation (2) & (4), we get
(SA + SB) + (SA – SB) = 20 +100
= 2SA = 120
Therefore we get
By putting the value of SA in equation (4) we get,
60 + SB = 100
SB = 100 – 60 = 40 km/hr
Therefore we get the speed of Car A as 60 km/hr and Car B as 40 km/hr.
Note: Students must note that whenever two cars are moving in the same direction, they will never meet at a point between them. In the same way, if they are moving towards each other, they will always meet at a point between them. Also students can cross check their answer by putting SA and SB back to equations and checking if they are satisfying the equations or not.
Here students can also calculate SA and SB by using direct formula that is
Where D is the distance between A and B.
Complete step-by-step answer:
When they are moving in opposite directions, use (SA + SB)
We are given that place A and B are 100km apart. Also A and B starts at same time from positions A and B respectively. When they move in the same direction, they meet in 5 hours and when they move in opposite directions, that is, towards each other, they meet in 1 hour. We have to find the speeds of Car A and Car B.
Let us diagrammatically consider the situation,

Case I: When both cars are moving in the same direction.
Let us consider that, these cars meet at point C.
Let BC=
Let the speed of Car A is SA and speed of Car B is SB.
In this case, we are given that Car A and Car B meet after 5 hours. Since they meet at C, therefore this means that Car A takes 5 hours to reach C and Car B also takes 5 hours to reach C. Hence we get,
Time taken by car A to reach C = Time taken by Car B to reach C = 5 hours
As we know that time taken
Therefore, we get time taken by Car A to reach C
By putting the value of distance travelled = AB +BC.
Speed of Car A = SA and time taken = 5 hours, we get,
Also, we get time taken by Car B to reach C
By putting the value of distance travelled =
Speed of Car B = SB and time taken = 5 hours, we get,
By cross multiplying above equation, we get,
By putting the value of
By cross multiplying above equation, we get,
By taking out 5 common in above equation, we get,
SA – SB = 20 …………..(2)
Case II: When cars are moving towards each other or in opposite directions.

Let us consider that these cars meet at C.
Let AC =
Since AC + CB = 100km
Therefore, CB = 100 -
In this case, we are given that Car A and Car B meet after 1 hour
Since they meet at C, therefore this means that Car A takes 1 hour to reach C and Car B also takes 1 hour to reach C. Hence we get,
Time taken by Car A to reach C = Time taken by Car B to reach C = 1 hour
As we know that time taken
Therefore, we get time taken by Car A to reach C
By putting the value of distance travelled = AC =
Speed of Car A = SA and time taken = 1 hour, we get,
By cross multiplying above equation, we get,
Also, we get time taken by Car B to reach C
By putting the value of distance travelled = BC =
Speed of Car B = SB and time taken = 1 hour, we get,
By cross multiplying above equation, we get,
By putting the value of
(100 – SA) = SB
Or (SA + SB) = 100……………… (4)
By adding equation (2) & (4), we get
(SA + SB) + (SA – SB) = 20 +100
= 2SA = 120
Therefore we get
By putting the value of SA in equation (4) we get,
60 + SB = 100
SB = 100 – 60 = 40 km/hr
Therefore we get the speed of Car A as 60 km/hr and Car B as 40 km/hr.
Note: Students must note that whenever two cars are moving in the same direction, they will never meet at a point between them. In the same way, if they are moving towards each other, they will always meet at a point between them. Also students can cross check their answer by putting SA and SB back to equations and checking if they are satisfying the equations or not.
Here students can also calculate SA and SB by using direct formula that is
Where D is the distance between A and B.
Recently Updated Pages
Express the following as a fraction and simplify a class 7 maths CBSE

The length and width of a rectangle are in ratio of class 7 maths CBSE

The ratio of the income to the expenditure of a family class 7 maths CBSE

How do you write 025 million in scientific notatio class 7 maths CBSE

How do you convert 295 meters per second to kilometers class 7 maths CBSE

Write the following in Roman numerals 25819 class 7 maths CBSE

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE

Draw a diagram of a flower and name the parts class 12 biology ICSE




