PQ is a normal chord of the parabola \[{{y}^{2}}=4ax\] at P, A being the vertex of the parabola. Through P a line is drawn parallel to AQ meeting the x-axis in R. Then line length of AR is
A. Equal to the length of the latus rectum.
B. Equal to the Focal distance of the point P.
C. Equal to twice the Focal distance of the point P.
D. Equal to the distance of the point P from the directrix.
Answer
Verified357k+ views
Hint: First, we will assume the two points P and Q in the parametric form for the parabola $y^2=4ax$. We know that the slope of the tangent can be calculated by differentiating the equation of the curve at the point P. Then, we know that the product of slopes of two perpendicular lines \[=-1\]. After that use the equation that is \[(y-{{y}_{1}})=m(x-{{x}_{1}})\] then by substituting the value of m, \[{{x}_{1}}\] and \[{{y}_{1}}\] in the above equation to get the required answer.
Complete answer:
Suppose two points of the normal chord lying on parabola as \[\left( a{{t}^{2}}_{1},2a{{t}^{2}}_{1} \right)\] and \[\left( a{{t}^{2}}_{2},2a{{t}^{2}}_{2} \right)\]
.Get the slope of the chord by differentiating the curve (Parabola) at the point where normal is drawn. Get the slope of a line using the relation, \[m=\dfrac{{{y}_{2}}-{{y}_{1}}}{{{x}_{2}}-{{x}_{1}}}\] where, \[\left( {{x}_{1}},{{y}_{1}} \right)\] and \[\left( {{x}_{2}},{{y}_{2}} \right)\] are the points lying on the line.
As we need to find the line length of AR with the axis of parabola, \[{{y}^{2}}=4ax\] with the help of given information in the problem.
We know the axis of the parabola \[{{y}^{2}}=4ax\] is x – axis, as it is symmetric about x – axis. So, we need to determine the line length AR along the x – axis.
As, we need to find the slope of a chord of, which is normal at one end that is at point P So, let the two ends of the chord are \[\left( a{{t}^{2}}_{1},2a{{t}^{2}}_{1} \right)\] and \[\left( a{{t}^{2}}_{2},2a{{t}^{2}}_{2} \right)\].
Parametric coordinates for \[{{y}^{2}}=4ax\]at \[\left( a{{t}^{2}}_{1},2a{{t}^{2}}_{1} \right)\]
So, diagram is represented as
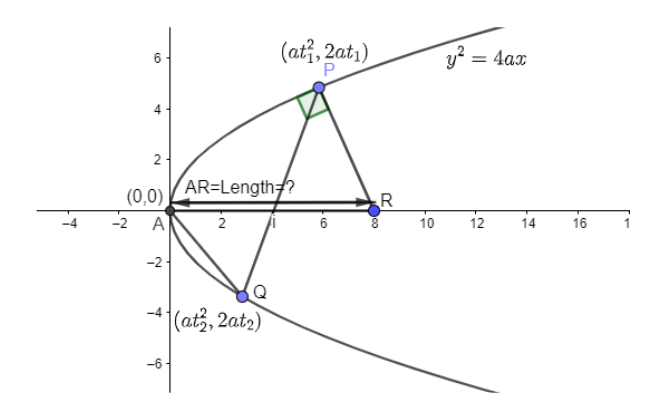
Diagram from Geogebra
Consider PQ is acting as a normal at the point P.
We know the slope of tangent at point P can be given as,
\[{{\left. \dfrac{dy}{dx} \right|}_{\left( a{{t}^{2}}_{1},2a{{t}^{2}}_{1} \right)}}--(i)\]
Where, we need to use relation, \[{{y}^{2}}=4ax\].
So, differentiating, \[{{y}^{2}}=4ax\], we get:
\[\dfrac{d\left( {{y}^{2}} \right)}{dx}=\dfrac{d\left( 4ax \right)}{dx}\]
By simplifying this we get:
\[2y\dfrac{dy}{dx}=4a\]
Where, we know that
\[\dfrac{d\left( {{x}^{n}} \right)}{dx}=n{{x}^{n-1}}\]
Hence, we get:
\[y\dfrac{dy}{dx}=2\]
By dividing by ‘y’ on both sides we get:
\[\dfrac{dy}{dx}=\dfrac{2}{y}\]
Now, we can get slope of tangent at point P as,
\[{{\left. \dfrac{dy}{dx} \right|}_{\left( a{{t}^{2}}_{1},2a{{t}^{2}}_{1} \right)}}=\dfrac{2}{2{{t}_{1}}}=\dfrac{1}{{{t}_{1}}}--(ii)\]
Now, we know tangent is perpendicular to the normal at the point of tangency for any conic. And as, we know the relation between slope of two perpendicular lines is given as, Product of two perpendicular lines \[=-1--(iii)\]
So, we get,
\[\text{Slope of normal}\,\text{at}\,\text{P}\,\,\times \,\,\text{Slope of tangent}\,\text{at}\,\text{P}=-1\]
From equation (ii) we get:
\[\text{Slope of normal}\,\text{at}\,\text{P}\,\,\times \,\,\left( \dfrac{1}{{{t}_{1}}} \right)=-1\]
By simplifying this we get:
\[\text{Slope of normal}\,\text{at}\,\text{P}\,\,=-{{t}_{1}}\]
Now, we can calculate slope of normal at P that is slope of line PQ by formula,
\[\text{Slope}=\dfrac{{{y}_{2}}-{{y}_{1}}}{{{x}_{2}}-{{x}_{1}}}--(iv)\]
So, we get:
Slope of normal at P\[=\dfrac{2a{{t}_{2}}-2a{{t}_{1}}}{a{{t}^{2}}_{2}-a{{t}^{2}}_{1}}\]
By taking the a common and get cancelled in numerator and denominator we get:
\[\Rightarrow \dfrac{2{{t}_{2}}-2{{t}_{1}}}{{{t}^{2}}_{2}-{{t}^{2}}_{1}}\]
By taking the 2 common we get:
\[\Rightarrow \dfrac{2\left( {{t}_{2}}-{{t}_{1}} \right)}{{{t}^{2}}_{2}-{{t}^{2}}_{1}}\]
By using the property of \[\left( a-b \right)\left( a+b \right)={{a}^{2}}-{{b}^{2}}\] we get:
\[\Rightarrow \dfrac{2\left( {{t}_{2}}-{{t}_{1}} \right)}{\left( {{t}_{2}}-{{t}_{1}} \right)\left( {{t}_{2}}+{{t}_{1}} \right)}\]
By cancelling \[\left( {{t}_{2}}-{{t}_{1}} \right)\] on numerator and denominator we get:
\[\Rightarrow \dfrac{2}{\left( {{t}_{2}}+{{t}_{1}} \right)}\]
As, we have already calculated the slope of normal at point P as \[-{{t}_{1}}\]
So, we get:
\[\Rightarrow \dfrac{2}{\left( {{t}_{2}}+{{t}_{1}} \right)}=-{{t}_{1}}\]
By simplifying and cross multiplication, we get:
\[\Rightarrow -2={{t}_{1}}\left( {{t}_{2}}+{{t}_{1}} \right)\]
By further solving this we get:
\[\Rightarrow -2={{t}_{1}}{{t}_{2}}+{{t}^{2}}_{1}\]
By rearranging the term, we get:
\[\Rightarrow -2-{{t}^{2}}_{1}={{t}_{1}}{{t}_{2}}--(v)\]
Now, we can also calculate the slopes of AQ by using equation (iii).
So, we get slope of AQ from the equation (iv) as,
Slope of AQ \[=\dfrac{2a{{t}_{2}}-0}{a{{t}^{2}}_{2}-0}\]
By simplifying this we get:
\[\Rightarrow \dfrac{2{{t}_{2}}}{{{t}^{2}}_{2}}=\dfrac{2}{{{t}_{2}}}\]
Therefore, Slope of AQ \[=\dfrac{2}{{{t}_{2}}}\].
Equation of the line PR parallel to AQ is given as
\[\Rightarrow (y-2a{{t}_{1}})=\dfrac{2}{{{t}_{2}}}(x-a{{t}^{2}}_{1})\]
Since, it meets x-axis in R that is \[y=0\]
\[\Rightarrow (0-2a{{t}_{1}})=\dfrac{2}{{{t}_{2}}}(x-a{{t}^{2}}_{1})\]
By simplifying this we get:
\[\Rightarrow (-2a{{t}_{1}})=\dfrac{2}{{{t}_{2}}}(x-a{{t}^{2}}_{1})\]
Here, 2 gets cancelled we get:
\[\Rightarrow (-a{{t}_{1}})=\dfrac{1}{{{t}_{2}}}(x-a{{t}^{2}}_{1})\]
By cross multiplying we get:
\[\Rightarrow x=a{{t}^{2}}_{1}-a{{t}_{1}}{{t}_{2}}\]
Substitute the value of equation (v) on above equation we get:
\[\Rightarrow x=a{{t}^{2}}_{1}-a\left( -2-{{t}^{2}}_{1} \right)\]
By further simplifying this we get:
\[\Rightarrow x=2a+2a{{t}^{2}}_{1}\]
By taking 2 common we get:
\[\Rightarrow x=2\left( a+a{{t}^{2}}_{1} \right)\]
From the above equation we can say that the line length AR is twice the focal distance of point P.
So, the correct option is “option C”.
Note:
In these types of the problem, always careful about the option which is given one may confuse between the option B and Option C and they may tick the option B that is wrong because while find the answer you may get the answer as twice the focal distance of point P hence, you should the tick the option C only. Hence, you have to read the option carefully and also compare the answer you get with the given option then tick that option to get the correct answer. Parallel lines are the lines that never meet each other. They have the exact same gap between them throughout. In this question you must know about the condition of parallel: that if two lines are parallel then, they have no solution pair of linear equations is inconsistent. If two lines are coincident then, they have an infinite solution and a pair of linear equations is consistent; If two lines are intersecting then, they have a unique solution and a pair of linear equations is consistent.
Complete answer:
Suppose two points of the normal chord lying on parabola as \[\left( a{{t}^{2}}_{1},2a{{t}^{2}}_{1} \right)\] and \[\left( a{{t}^{2}}_{2},2a{{t}^{2}}_{2} \right)\]
.Get the slope of the chord by differentiating the curve (Parabola) at the point where normal is drawn. Get the slope of a line using the relation, \[m=\dfrac{{{y}_{2}}-{{y}_{1}}}{{{x}_{2}}-{{x}_{1}}}\] where, \[\left( {{x}_{1}},{{y}_{1}} \right)\] and \[\left( {{x}_{2}},{{y}_{2}} \right)\] are the points lying on the line.
As we need to find the line length of AR with the axis of parabola, \[{{y}^{2}}=4ax\] with the help of given information in the problem.
We know the axis of the parabola \[{{y}^{2}}=4ax\] is x – axis, as it is symmetric about x – axis. So, we need to determine the line length AR along the x – axis.
As, we need to find the slope of a chord of, which is normal at one end that is at point P So, let the two ends of the chord are \[\left( a{{t}^{2}}_{1},2a{{t}^{2}}_{1} \right)\] and \[\left( a{{t}^{2}}_{2},2a{{t}^{2}}_{2} \right)\].
Parametric coordinates for \[{{y}^{2}}=4ax\]at \[\left( a{{t}^{2}}_{1},2a{{t}^{2}}_{1} \right)\]
So, diagram is represented as
Diagram from Geogebra
Consider PQ is acting as a normal at the point P.
We know the slope of tangent at point P can be given as,
\[{{\left. \dfrac{dy}{dx} \right|}_{\left( a{{t}^{2}}_{1},2a{{t}^{2}}_{1} \right)}}--(i)\]
Where, we need to use relation, \[{{y}^{2}}=4ax\].
So, differentiating, \[{{y}^{2}}=4ax\], we get:
\[\dfrac{d\left( {{y}^{2}} \right)}{dx}=\dfrac{d\left( 4ax \right)}{dx}\]
By simplifying this we get:
\[2y\dfrac{dy}{dx}=4a\]
Where, we know that
\[\dfrac{d\left( {{x}^{n}} \right)}{dx}=n{{x}^{n-1}}\]
Hence, we get:
\[y\dfrac{dy}{dx}=2\]
By dividing by ‘y’ on both sides we get:
\[\dfrac{dy}{dx}=\dfrac{2}{y}\]
Now, we can get slope of tangent at point P as,
\[{{\left. \dfrac{dy}{dx} \right|}_{\left( a{{t}^{2}}_{1},2a{{t}^{2}}_{1} \right)}}=\dfrac{2}{2{{t}_{1}}}=\dfrac{1}{{{t}_{1}}}--(ii)\]
Now, we know tangent is perpendicular to the normal at the point of tangency for any conic. And as, we know the relation between slope of two perpendicular lines is given as, Product of two perpendicular lines \[=-1--(iii)\]
So, we get,
\[\text{Slope of normal}\,\text{at}\,\text{P}\,\,\times \,\,\text{Slope of tangent}\,\text{at}\,\text{P}=-1\]
From equation (ii) we get:
\[\text{Slope of normal}\,\text{at}\,\text{P}\,\,\times \,\,\left( \dfrac{1}{{{t}_{1}}} \right)=-1\]
By simplifying this we get:
\[\text{Slope of normal}\,\text{at}\,\text{P}\,\,=-{{t}_{1}}\]
Now, we can calculate slope of normal at P that is slope of line PQ by formula,
\[\text{Slope}=\dfrac{{{y}_{2}}-{{y}_{1}}}{{{x}_{2}}-{{x}_{1}}}--(iv)\]
So, we get:
Slope of normal at P\[=\dfrac{2a{{t}_{2}}-2a{{t}_{1}}}{a{{t}^{2}}_{2}-a{{t}^{2}}_{1}}\]
By taking the a common and get cancelled in numerator and denominator we get:
\[\Rightarrow \dfrac{2{{t}_{2}}-2{{t}_{1}}}{{{t}^{2}}_{2}-{{t}^{2}}_{1}}\]
By taking the 2 common we get:
\[\Rightarrow \dfrac{2\left( {{t}_{2}}-{{t}_{1}} \right)}{{{t}^{2}}_{2}-{{t}^{2}}_{1}}\]
By using the property of \[\left( a-b \right)\left( a+b \right)={{a}^{2}}-{{b}^{2}}\] we get:
\[\Rightarrow \dfrac{2\left( {{t}_{2}}-{{t}_{1}} \right)}{\left( {{t}_{2}}-{{t}_{1}} \right)\left( {{t}_{2}}+{{t}_{1}} \right)}\]
By cancelling \[\left( {{t}_{2}}-{{t}_{1}} \right)\] on numerator and denominator we get:
\[\Rightarrow \dfrac{2}{\left( {{t}_{2}}+{{t}_{1}} \right)}\]
As, we have already calculated the slope of normal at point P as \[-{{t}_{1}}\]
So, we get:
\[\Rightarrow \dfrac{2}{\left( {{t}_{2}}+{{t}_{1}} \right)}=-{{t}_{1}}\]
By simplifying and cross multiplication, we get:
\[\Rightarrow -2={{t}_{1}}\left( {{t}_{2}}+{{t}_{1}} \right)\]
By further solving this we get:
\[\Rightarrow -2={{t}_{1}}{{t}_{2}}+{{t}^{2}}_{1}\]
By rearranging the term, we get:
\[\Rightarrow -2-{{t}^{2}}_{1}={{t}_{1}}{{t}_{2}}--(v)\]
Now, we can also calculate the slopes of AQ by using equation (iii).
So, we get slope of AQ from the equation (iv) as,
Slope of AQ \[=\dfrac{2a{{t}_{2}}-0}{a{{t}^{2}}_{2}-0}\]
By simplifying this we get:
\[\Rightarrow \dfrac{2{{t}_{2}}}{{{t}^{2}}_{2}}=\dfrac{2}{{{t}_{2}}}\]
Therefore, Slope of AQ \[=\dfrac{2}{{{t}_{2}}}\].
Equation of the line PR parallel to AQ is given as
\[\Rightarrow (y-2a{{t}_{1}})=\dfrac{2}{{{t}_{2}}}(x-a{{t}^{2}}_{1})\]
Since, it meets x-axis in R that is \[y=0\]
\[\Rightarrow (0-2a{{t}_{1}})=\dfrac{2}{{{t}_{2}}}(x-a{{t}^{2}}_{1})\]
By simplifying this we get:
\[\Rightarrow (-2a{{t}_{1}})=\dfrac{2}{{{t}_{2}}}(x-a{{t}^{2}}_{1})\]
Here, 2 gets cancelled we get:
\[\Rightarrow (-a{{t}_{1}})=\dfrac{1}{{{t}_{2}}}(x-a{{t}^{2}}_{1})\]
By cross multiplying we get:
\[\Rightarrow x=a{{t}^{2}}_{1}-a{{t}_{1}}{{t}_{2}}\]
Substitute the value of equation (v) on above equation we get:
\[\Rightarrow x=a{{t}^{2}}_{1}-a\left( -2-{{t}^{2}}_{1} \right)\]
By further simplifying this we get:
\[\Rightarrow x=2a+2a{{t}^{2}}_{1}\]
By taking 2 common we get:
\[\Rightarrow x=2\left( a+a{{t}^{2}}_{1} \right)\]
From the above equation we can say that the line length AR is twice the focal distance of point P.
So, the correct option is “option C”.
Note:
In these types of the problem, always careful about the option which is given one may confuse between the option B and Option C and they may tick the option B that is wrong because while find the answer you may get the answer as twice the focal distance of point P hence, you should the tick the option C only. Hence, you have to read the option carefully and also compare the answer you get with the given option then tick that option to get the correct answer. Parallel lines are the lines that never meet each other. They have the exact same gap between them throughout. In this question you must know about the condition of parallel: that if two lines are parallel then, they have no solution pair of linear equations is inconsistent. If two lines are coincident then, they have an infinite solution and a pair of linear equations is consistent; If two lines are intersecting then, they have a unique solution and a pair of linear equations is consistent.
Recently Updated Pages
Using the following information to help you answer class 12 chemistry CBSE
Basicity of sulphurous acid and sulphuric acid are
Master Class 12 Economics: Engaging Questions & Answers for Success
Master Class 12 Maths: Engaging Questions & Answers for Success
Master Class 12 Biology: Engaging Questions & Answers for Success
Master Class 12 Physics: Engaging Questions & Answers for Success
Trending doubts
Which are the Top 10 Largest Countries of the World?
Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE
Draw a labelled sketch of the human eye class 12 physics CBSE
What is the Full Form of PVC, PET, HDPE, LDPE, PP and PS ?
What is a transformer Explain the principle construction class 12 physics CBSE
What are the major means of transport Explain each class 12 social science CBSE