Question

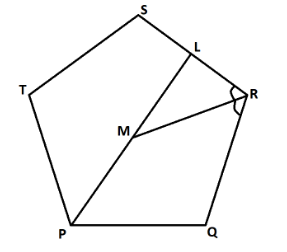
$PQRST$ is a regular pentagon and bisector of $\angle TPQ$ meets $SR$ at $L$ . If the bisector of $\angle SRQ$ meets $PL$ at $M$ . Find $\angle RML$ .

A) ${56^ \circ }$
B) ${40^ \circ }$
C) ${36^ \circ }$
D) ${54^ \circ }$

Answer 1

Hint: Use the formula to calculate the interior angle of the given pentagon so that we will have measure of each interior angle of the given pentagon. Then use the given information about the bisectors of the angles and then observe that we have calculated each angle of a quadrilateral drawn inside the regular pentagon.

Complete step-by-step answer:
For any polygon with $n$ sides, the interior angle is given as follows:
$\theta = \dfrac{{\left( {n - 2} \right) \times 180}}{n}$
Note that the angle is measured in degrees.
In the given case it is a pentagon, so we will substitute $n = 5$ in the above formula and write the following:
$ \Rightarrow $$\theta = \dfrac{{\left( {5 - 2} \right) \times 180}}{5}$
On simplifying, we write that each of the interior angles measures ${108^ \circ }$.
It is given that $PL$ is the bisector of angle $\angle TPQ$.
The measure of the angle $\angle LPQ = {54^ \circ }$. … (1)
The angle $\angle RQP$ is an interior angle, therefore $\angle RQP = {108^ \circ }$. … (2)
Similarly, angle $\angle LRQ$ is also an interior angle, therefore $\angle LRQ = {108^ \circ }$ … (3)
Now observe that the quadrilateral $\square LRQP$ has the sum of all its measures ${360^ \circ }$.
Therefore,
$ \Rightarrow $$\angle LPQ + \angle PQR + \angle QRL + \angle RLP = {360^ \circ }$
Now substitute the values from (1), (2) and (3) we get,
$ \Rightarrow $${54^ \circ } + {108^ \circ } + {108^ \circ } + \angle RLP = {360^ \circ }$
Rearranging the terms, we write,
$\angle RLP = {90^ \circ }$
It is also given that the $RM$ is an angle bisector, therefore $\angle LRM = {54^ \circ }$. … (4)
Now in the triangle $\vartriangle LRM$ we can write the following:
$ \Rightarrow $$\angle LRM + \angle RML + \angle MLR = {180^ \circ }$
Now substitute the obtained values in the above equation.
$ \Rightarrow $${54^ \circ } + \angle RML + {90^ \circ } = {180^ \circ }$
Rearranging the terms, we write the following:
$ \Rightarrow $$\angle RML = {36^ \circ }$

Thus, the correct answer is C.

Note: The first thing to notice here is that the given polygon is regular so we can calculate the interior angle by using direct formula. After that notice where we can form a rectangle or a triangle etc so that we can use it to solve the given problem. Make sure that you don’t make any mistake in the calculation.