
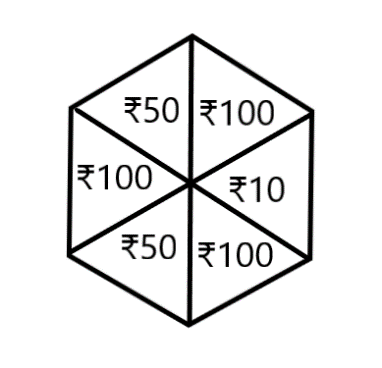
What is the probability of the spinner landing on${\text{Rs 100}}$
A.$\dfrac{1}{2}$
B.$\dfrac{1}{3}$
C.$\dfrac{1}{6}$
D.$\dfrac{5}{6}$

Answer
396.3k+ views
Hint: In order to find the probability of the spinner landing on ${\text{Rs 100}}$, find the total possible outcomes from the spinning wheel by counting all the values, then calculate the favourable outcomes that is the number of chances we can get ${\text{Rs 100}}$. Divide them to get the probability.
Formula used:
${\text{Probability = }}\dfrac{{{\text{Number of Favourable outcomes}}}}{{{\text{Number of Total possible favourable outcomes}}}}$
Complete answer:
We have a spinning wheel with some values of Rs written on it.
We need to find the probability of the spinner landing on ${\text{Rs 100}}$.For, that we need to find the possible outcomes and favourable outcomes.
We can see that there are a total of six values written on the spinner wheel, so any value can be obtained from this if the wheel is rotated.
So, total number of possible outcomes $ = 6$.
We need ${\text{Rs 100}}$, so there are three ${\text{Rs 100}}$, written on the spinning wheel, so when we rotate it, there are three chances of getting ${\text{Rs 100}}$.
Therefore, Number of Favourable outcomes $ = 3$
From the definition of Probability, we know that:
$\Rightarrow$ ${\text{Probability = }}\dfrac{{{\text{Number of Favourable outcomes}}}}{{{\text{Number of Total possible favourable outcomes}}}}$
Substituting the obtained value in it and, we get:
$\Rightarrow$ ${\text{Probability = }}\dfrac{3}{6}$
Simplifying it more, we get:
$\Rightarrow$ ${\text{Probability = }}\dfrac{1}{2}$
Hence, the probability of the spinner landing on ${\text{Rs 100}}$ is $\dfrac{1}{2}$, which is the option A.
Therefore, Option A is correct.
Note:
1.Probability is nothing but the number of favourable outcomes divided by the total number of possible outcomes. It is also written as ${\text{Probability = }}\dfrac{{N\left( E \right)}}{{N\left( S \right)}}$, where n(E) is the number of possible events and n(E) is the number of sample space.
2.Using the same method, we can find the probability of getting any other number on the spinning wheel.
Formula used:
${\text{Probability = }}\dfrac{{{\text{Number of Favourable outcomes}}}}{{{\text{Number of Total possible favourable outcomes}}}}$
Complete answer:
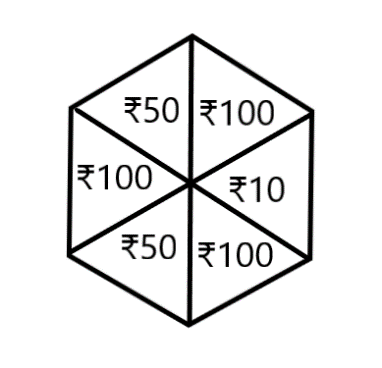
We have a spinning wheel with some values of Rs written on it.

We need to find the probability of the spinner landing on ${\text{Rs 100}}$.For, that we need to find the possible outcomes and favourable outcomes.
We can see that there are a total of six values written on the spinner wheel, so any value can be obtained from this if the wheel is rotated.
So, total number of possible outcomes $ = 6$.
We need ${\text{Rs 100}}$, so there are three ${\text{Rs 100}}$, written on the spinning wheel, so when we rotate it, there are three chances of getting ${\text{Rs 100}}$.
Therefore, Number of Favourable outcomes $ = 3$
From the definition of Probability, we know that:
$\Rightarrow$ ${\text{Probability = }}\dfrac{{{\text{Number of Favourable outcomes}}}}{{{\text{Number of Total possible favourable outcomes}}}}$
Substituting the obtained value in it and, we get:
$\Rightarrow$ ${\text{Probability = }}\dfrac{3}{6}$
Simplifying it more, we get:
$\Rightarrow$ ${\text{Probability = }}\dfrac{1}{2}$
Hence, the probability of the spinner landing on ${\text{Rs 100}}$ is $\dfrac{1}{2}$, which is the option A.
Therefore, Option A is correct.
Note:
1.Probability is nothing but the number of favourable outcomes divided by the total number of possible outcomes. It is also written as ${\text{Probability = }}\dfrac{{N\left( E \right)}}{{N\left( S \right)}}$, where n(E) is the number of possible events and n(E) is the number of sample space.
2.Using the same method, we can find the probability of getting any other number on the spinning wheel.
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Types of lever in which effort is in between fulcrum class 12 physics CBSE

Which are the Top 10 Largest Countries of the World?

A two input XOR Gate produces a high output only when class 12 physics CBSE

What is a transformer Explain the principle construction class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Draw a labelled sketch of the human eye class 12 physics CBSE




