Question

How do I prove that if a quadrilateral has consecutive angles that are supplementary, then it is a parallelogram?

Answer 1

Hint:
In this question, we have been asked to prove that if a quadrilateral has consecutive angles that are supplementary, then it is a parallelogram. Using the property that if two lines are cut by a transversal and the interior angles on the same side of the transversal are supplementary, then the lines are parallel, proving both the pair of sides to be parallel. Once proven parallel, we can say that the quadrilateral is a parallelogram.

Complete step by step solution:
We have been asked to prove that if a quadrilateral has consecutive angles that are supplementary, then it is a parallelogram. We will start by writing what is given and is to be proved.

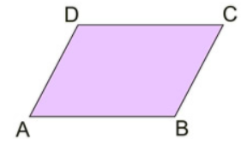
It is given that:
1) A quadrilateral ABCD.
2) $\measuredangle A$ supplements $\measuredangle B$, $\measuredangle B$ supplements $\measuredangle C$, $\measuredangle C$ supplements $\measuredangle D$ and $\measuredangle D$ supplements $\measuredangle A$.
To prove: Quadrilateral ABCD is a parallelogram.
Proof: Consider AC and BD. AB is the transversal of AC and BD.
$\measuredangle A$ and $\measuredangle B$ are two angles on the same side of the transversal AB.
According to a property, if two lines are cut by a transversal and the interior angles on the same side of the transversal are supplementary, then the lines are parallel.
Here, it is given that $\measuredangle A$ supplements $\measuredangle B$.
$ \Rightarrow \measuredangle A + \measuredangle B = {180^ \circ }$
Therefore, the lines AC and BD are parallel to each other.
Now, consider AB and CD. AC is the transversal of AB and CD.
$\measuredangle A$ and $\measuredangle D$ are two angles on the same side of the transversal AB.
According to a property, if two lines are cut by a transversal and the interior angles on the same side of the transversal are supplementary, then the lines are parallel.
Here, it is given that $\measuredangle D$ supplements $\measuredangle A$.
$ \Rightarrow \measuredangle A + \measuredangle D = {180^ \circ }$
Therefore, the lines AB and CD are parallel to each other.
Now, since both the pairs of opposite sides are parallel, ABCD is a parallelogram.
Hence proved.

Final solution: Hence, if a quadrilateral has consecutive angles that are supplementary, then it is a parallelogram.

Note:
In this question, it should be noted that $\measuredangle A$ supplements $\measuredangle B$, $\measuredangle B$ supplements $\measuredangle C$, $\measuredangle C$ supplements $\measuredangle D$ and $\measuredangle D$ supplements $\measuredangle A$. If opposite angles are supplementary, then it is not a parallelogram.
Example:

Here, $\angle {127^ \circ } + \angle {53^ \circ } = \angle {180^ \circ }$ and $\angle {117^ \circ } + \angle {63^ \circ } = \angle {180^ \circ }$.
So, opposite angles are supplementary. But for parallelograms, opposite angles should be equal and opposite sides should be parallel.
Thus, all consecutive angles should be supplementary.
Final solution: Hence, if a quadrilateral has consecutive angles that are supplementary, then it is a parallelogram.