
Prove that in a parallelogram, the bisector of any two consecutive angles intersect at the right angle.
Answer
502.8k+ views
Hint: Parallelogram is a simple quadrilateral with two pairs of parallel sides. The opposite sides of a parallelogram are equal in length, and also opposite angles of a parallelogram are equal. One of the properties of a parallelogram says that the sum of any two consecutive angles is 180; hence in this question, find the value of the two consecutive angles and then find their bisecting angles.
In this question, we need to prove that the bisector of any two consecutive angles intersect at the right angle for which we need to follow the above discussed property of the parallelogram.
Complete step by step answer:
In the given figure
Side AD is parallel to BC, and AB is parallel to DC where the side BC is transversal, hence
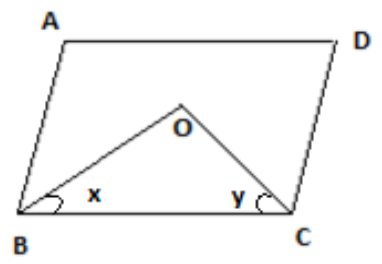
Since one of the properties of parallelogram say the sum of the consecutive angle of a parallelogram is a supplementary angle hence, we can write,
Now draw a line OB bisecting
Hence in the
This can be written as
Hence by solving
Since
So the value of
Therefore we can say the bisector of any two consecutive angles intersect at the right angle.
Hence proved.
Note: A property of that parallelogram says that if a parallelogram has all sides equal, then their diagonal bisector intersects perpendicularly. An angle bisector is a ray that splits an angle into two consecutive congruent, smaller angles.
In this question, we need to prove that the bisector of any two consecutive angles intersect at the right angle for which we need to follow the above discussed property of the parallelogram.
Complete step by step answer:
In the given figure
Side AD is parallel to BC, and AB is parallel to DC where the side BC is transversal, hence

Since one of the properties of parallelogram say the sum of the consecutive angle of a parallelogram is a supplementary angle hence, we can write,
Now draw a line OB bisecting
Hence in the
This can be written as
Hence by solving
Since
So the value of
Therefore we can say the bisector of any two consecutive angles intersect at the right angle.
Hence proved.
Note: A property of that parallelogram says that if a parallelogram has all sides equal, then their diagonal bisector intersects perpendicularly. An angle bisector is a ray that splits an angle into two consecutive congruent, smaller angles.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Questions & Answers - Ask your doubts

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Science: Engaging Questions & Answers for Success

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Difference Between Plant Cell and Animal Cell

Given that HCF 306 657 9 find the LCM 306 657 class 9 maths CBSE

The highest mountain peak in India is A Kanchenjunga class 9 social science CBSE

What is pollution? How many types of pollution? Define it

Differentiate between the Western and the Eastern class 9 social science CBSE




