Question

Prove that moment of couple = Force x Couple Arm

Answer 1

Hint: Since firstly we have to know due to the force which type of motion is taking place. The body AB is pivoted at point O. So the body will do rotational motion. Now we find torque for both the forces, if they make the same sense of rotation we add them or if they have opposite senses of rotation we can subtract them to obtain the final result.

Complete step by step answer:
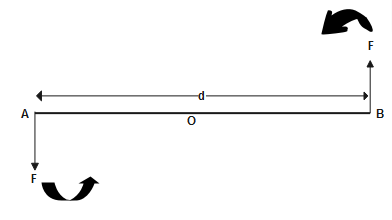
 Since here two forces F are acting in opposite directions from point A and B respectively. Since these forces are equal in magnitude and opposite indirection but their line of action is not same so these two forces will never cancel out but these pair of forces will provide the rotational motion to the system AB, such pair of forces is called couple, this couple produce torque on the system and system will rotate either clockwise or anticlockwise.
Here in the figure, Forces F are acting at point A and B respectively and due to these forces the system will rotate about point O which means these forces provide torque to the system about point O.
Since O is the centre of AB.
 \[\Rightarrow \]\[AO=BO=\dfrac{d}{2}\].
Now we will calculate torque due to these forces about point O.
Let us assume the torque due to force F at A is given by\[{{\tau }_{1}}\].
Let us assume the torque due to force F at B is given by\[{{\tau }_{2}}\].
Since Mathematical formula for torque is given by :-
\[Torque(\tau )=Force(F)\times dis\tan c{{e}_{\bot }}\]
So Torque of Force F acting at point A is –
\[{{\tau }_{1}}=F\times \dfrac{d}{2}(Anticlockwise)\] (Equation 1)
So Torque of Force F acting at point B is –
\[{{\tau }_{2}}=F\times \dfrac{d}{2}(Anticlockwise)\](Equation 2)
Since both the forces acting in the same direction as torque is the vector quantity so total torque is identified by simply adding both the torques.
\[{{\tau }_{net}}={{\tau }_{1}}+{{\tau }_{2}}\]
Put the value of torques from Equation 1 and 2, we get
\[{{\tau }_{net}}=(F\times \dfrac{d}{2})+(F\times \dfrac{d}{2})(Anticlockwise)\]
\[\therefore {{\tau }_{net}}=F\times d\]
Where F is the force
d is also defined as a couple arm.
So above formula can be written as:-
\[\tau =Force\times \text{Couple arm}\].

Note: When two forces are equal and opposite will not may or may not cancel each other, it all depends on their line of action. When they have the same line of action then these forces will cancel out and the system remains in equilibrium and when they have different lines of action they do not cancel out but they provide rotational motion to the system.