
Radius of the curved road on the national highway is $R$. Width of the road is $b$. The outer edge of the road is raised by $h$ with respect to the inner edge so that a car with velocity $v$ can pass safely over it. The value of $h$ is
(A) $\dfrac{{{v^2}b}}{{Rg}}$
(B) $\dfrac{v}{{Rgb}}$
(C) $\dfrac{{{v^2}R}}{{bg}}$
(D) $\dfrac{{{v^2}b}}{R}$
Answer
132.3k+ views
Hint: In this question we must apply the concept of equilibrium of the moving bodies. A body will be in equilibrium if all the forces acting on that body or object are balanced. In this question the centripetal force and the contact forces are to balance.
Complete step by step answer:
In this question, we have given the radius of the curved road is $R$, the width of the road is $b$, the outer edge of the road is raised by $h$ with respect to the inner edge, and the velocity of the car is $v$. We need to calculate the value of $h$.
First, we will draw the diagram based on the situation given in the question is shown below,
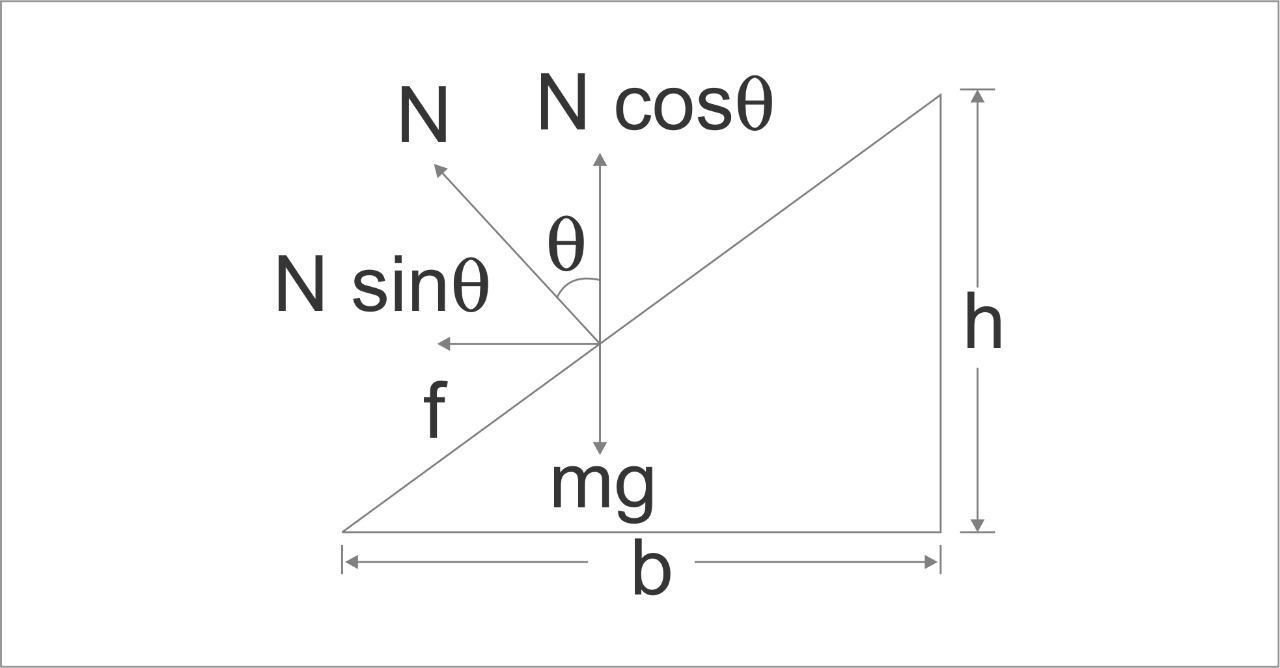
Figure-(1)
In the above diagram, $N$ represents the normal reaction of the car and the slope angle is $\theta $.
Now, we apply the concept of equilibrium for horizontal forces as well as the vertical forces.
From the above diagram, the horizontal forces can be balanced as,
$ \Rightarrow N\sin \theta = \dfrac{{m{v^2}}}{R}......\left( 1 \right)$
Here, $N$ is the normal force, $R$ is the radius of the road, $v$ is the velocity, $m$ is the mass of the vehicle and $\theta $ is the angle between the elevations of the road from the horizontal.
Similarly, the vertical forces can be balanced as,
$ \Rightarrow N\cos \theta = mg......\left( 2 \right)$
Now we divide equation (1) by equation (2) as,
$ \Rightarrow \dfrac{{N\sin \theta }}{{N\cos \theta }} = \dfrac{{\dfrac{{m{v^2}}}{R}}}{{mg}}$
Further, we solve the above equation.
$ \Rightarrow \tan \theta = \dfrac{{{v^2}}}{{Rg}}......\left( 3 \right)$
Now we calculate $\tan \theta $ from the diagram.
$ \Rightarrow \tan \theta = \dfrac{h}{b}......\left( 4 \right)$
Here, $h$ is the height of the road and $b$ is the width of road.
Now we compare equation (3) and (4) as,
$ \Rightarrow \dfrac{h}{b} = \dfrac{{{v^2}}}{{Rg}}$
After simplification we get,
$\therefore h = \dfrac{{{v^2}b}}{{Rg}}$
Therefore, the correct option is A.
Note: In this question, diagram plays an important role in the calculation. So, the diagrams should be made carefully. While balancing the forces it is necessary to look at the sign of forces because difference in sign will lead to wrong answers.
Complete step by step answer:
In this question, we have given the radius of the curved road is $R$, the width of the road is $b$, the outer edge of the road is raised by $h$ with respect to the inner edge, and the velocity of the car is $v$. We need to calculate the value of $h$.
First, we will draw the diagram based on the situation given in the question is shown below,

Figure-(1)
In the above diagram, $N$ represents the normal reaction of the car and the slope angle is $\theta $.
Now, we apply the concept of equilibrium for horizontal forces as well as the vertical forces.
From the above diagram, the horizontal forces can be balanced as,
$ \Rightarrow N\sin \theta = \dfrac{{m{v^2}}}{R}......\left( 1 \right)$
Here, $N$ is the normal force, $R$ is the radius of the road, $v$ is the velocity, $m$ is the mass of the vehicle and $\theta $ is the angle between the elevations of the road from the horizontal.
Similarly, the vertical forces can be balanced as,
$ \Rightarrow N\cos \theta = mg......\left( 2 \right)$
Now we divide equation (1) by equation (2) as,
$ \Rightarrow \dfrac{{N\sin \theta }}{{N\cos \theta }} = \dfrac{{\dfrac{{m{v^2}}}{R}}}{{mg}}$
Further, we solve the above equation.
$ \Rightarrow \tan \theta = \dfrac{{{v^2}}}{{Rg}}......\left( 3 \right)$
Now we calculate $\tan \theta $ from the diagram.
$ \Rightarrow \tan \theta = \dfrac{h}{b}......\left( 4 \right)$
Here, $h$ is the height of the road and $b$ is the width of road.
Now we compare equation (3) and (4) as,
$ \Rightarrow \dfrac{h}{b} = \dfrac{{{v^2}}}{{Rg}}$
After simplification we get,
$\therefore h = \dfrac{{{v^2}b}}{{Rg}}$
Therefore, the correct option is A.
Note: In this question, diagram plays an important role in the calculation. So, the diagrams should be made carefully. While balancing the forces it is necessary to look at the sign of forces because difference in sign will lead to wrong answers.
Recently Updated Pages
Sign up for JEE Main 2025 Live Classes - Vedantu

JEE Main Books 2023-24: Best JEE Main Books for Physics, Chemistry and Maths

JEE Main 2023 April 13 Shift 1 Question Paper with Answer Key

JEE Main 2023 April 11 Shift 2 Question Paper with Answer Key

JEE Main 2023 April 10 Shift 2 Question Paper with Answer Key

JEE Main 2023 (April 11th Shift 2) Physics Question Paper with Answer Key

Trending doubts
JEE Main 2025 Session 2: Application Form (Out), Exam Dates (Released), Eligibility & More

JEE Main 2025: Derivation of Equation of Trajectory in Physics

Electric Field Due to Uniformly Charged Ring for JEE Main 2025 - Formula and Derivation

Class 11 JEE Main Physics Mock Test 2025

Current Loop as Magnetic Dipole and Its Derivation for JEE

Inertial and Non-Inertial Frame of Reference - JEE Important Topic

Other Pages
JEE Advanced Marks vs Ranks 2025: Understanding Category-wise Qualifying Marks and Previous Year Cut-offs

Units and Measurements Class 11 Notes: CBSE Physics Chapter 1

NCERT Solutions for Class 11 Physics Chapter 2 Motion In A Straight Line

Important Questions for CBSE Class 11 Physics Chapter 1 - Units and Measurement

Motion In A Plane: Line Class 11 Notes: CBSE Physics Chapter 3

Waves Class 11 Notes: CBSE Physics Chapter 14




