
Rain is falling vertically with a speed of 30 m/s. A woman rides a bicycle with a speed of 10m/s in the north to south direction. What is the direction in which she should hold her umbrella then?
Answer
502.2k+ views
Hint: This is a question of vector addition and parallelogram law of vector addition can be applied to it. By using the properties of the right angle triangle we need to find the angle (tan
Formula used:
The angle is obtained by the relation:
Where
Complete step by step answer:
A rough diagram of rain and cycle motion as vectors can be drawn as:
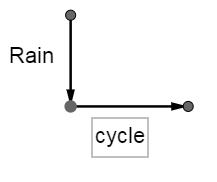
Thus, if we see the rain vector in a reversed position, we can use parallelogram law of vector addition.
Parallelogram law:
When two vectors have a common tail, their resultant is obtained by parallelogram law. If two vectors are such that the tail of one coincides with the head of another, we can reverse the sign of one of the vectors like in our case; we have to put a vector with a minus sign for the case of rain velocity.
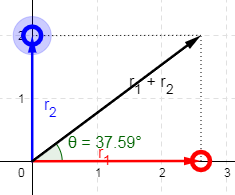
Thus, to find out the value of
But, we want to find the angle that the umbrella has to make with the vertical axis, so we use another triangle that has
In our case, by using this example, we can write:
Here, rain is for velocity of rain and cycle is for the velocity of the cycle.
Without considering the direction of rainfall and bicyclist as only magnitude of the vector is required, we may write:
The angle that the umbrella should make with the vertical axis is approximately
Note:
Since we were asked to find the direction in which the umbrella is to be held, we had to find the
Formula used:
The angle is obtained by the relation:
Where
Complete step by step answer:
A rough diagram of rain and cycle motion as vectors can be drawn as:

Thus, if we see the rain vector in a reversed position, we can use parallelogram law of vector addition.
Parallelogram law:
When two vectors have a common tail, their resultant is obtained by parallelogram law. If two vectors are such that the tail of one coincides with the head of another, we can reverse the sign of one of the vectors like in our case; we have to put a vector with a minus sign for the case of rain velocity.

Thus, to find out the value of
But, we want to find the angle that the umbrella has to make with the vertical axis, so we use another triangle that has
In our case, by using this example, we can write:
Here, rain is for velocity of rain and cycle is for the velocity of the cycle.
Without considering the direction of rainfall and bicyclist as only magnitude of the vector is required, we may write:
The angle that the umbrella should make with the vertical axis is approximately
Note:
Since we were asked to find the direction in which the umbrella is to be held, we had to find the
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
How much is 23 kg in pounds class 11 chemistry CBSE

Difference between physical and chemical change class 11 chemistry CBSE

Number of oneone functions from A to B where nA 4 and class 11 maths CBSE

Whales are warmblooded animals which live in cold seas class 11 biology CBSE

How many squares are there in a chess board A 1296 class 11 maths CBSE

In the tropical evergreen forest one of the common class 11 social science CBSE




