
Represent
Answer
491.1k+ views
Hint: Here we will use the Pythagoras Theorem for the representation of irrational numbers on the number line which states that:
It
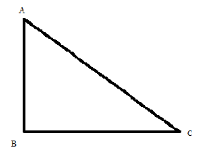
Then, the formula for finding the hypotenuses is as below:
Complete step-by-step answer:
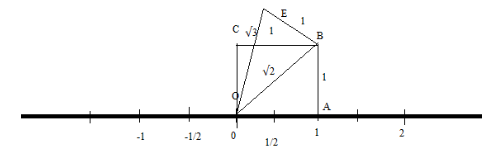
Step 1: First of all, we will draw a number line as shown below where
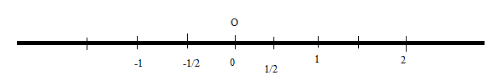
Step 2: Now, for representing
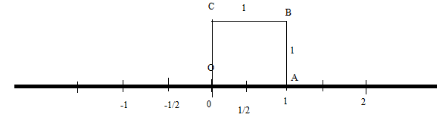
Here,
Step 3: Now, by joining the points
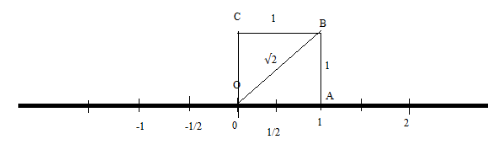
By using Pythagoras theorem in the triangle
By substituting the values of
By opening the brackets and adding inside the root, we get:
Step 4: Now, by again drawing a perpendicular from a point
By applying Pythagoras theorem in the right-angled triangle
By substituting the values of
By opening the brackets inside the root, we get:
By doing the addition of the brackets inside the root, we get:
Step 5: Now, we will draw an arc from the center point
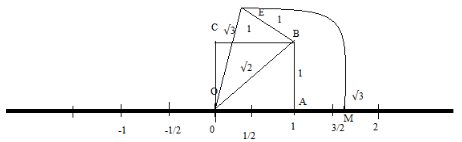
The point
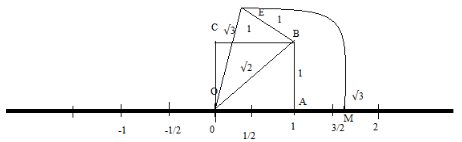
Note: Students need to take care while drawing the diagrams or by taking numbers on the number line. Sometimes, they take positive integers on the left side and negative integers on the right-hand side from the Centre of the number line which can lead to the incorrect answer.
It

Then, the formula for finding the hypotenuses is as below:
Complete step-by-step answer:
Step 1: First of all, we will draw a number line as shown below where
Step 2: Now, for representing

Here,
Step 3: Now, by joining the points

By using Pythagoras theorem in the triangle
By substituting the values of
By opening the brackets and adding inside the root, we get:
Step 4: Now, by again drawing a perpendicular from a point

By applying Pythagoras theorem in the right-angled triangle
By substituting the values of
By opening the brackets inside the root, we get:
By doing the addition of the brackets inside the root, we get:
Step 5: Now, we will draw an arc from the center point

The point

Note: Students need to take care while drawing the diagrams or by taking numbers on the number line. Sometimes, they take positive integers on the left side and negative integers on the right-hand side from the Centre of the number line which can lead to the incorrect answer.

Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Questions & Answers - Ask your doubts

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Science: Engaging Questions & Answers for Success

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Difference Between Plant Cell and Animal Cell

Given that HCF 306 657 9 find the LCM 306 657 class 9 maths CBSE

The highest mountain peak in India is A Kanchenjunga class 9 social science CBSE

What is pollution? How many types of pollution? Define it

Differentiate between the Western and the Eastern class 9 social science CBSE




