
Show that the torque on an electric dipole placed in a uniform electric field is
Answer
515.1k+ views
Hint: Here, we will proceed by writing down the forces experienced by the charges in the electric dipole placed in a uniform electric field. Then, we will obtain the expression corresponding to the torque experienced by these charges.
Formulas Used:
Complete Step-by-Step solution:
To show:
The force measure which causes an object to rotate about an axis is called the torque. Torque is a quantity of vectors, and its direction depends on the axis direction of force. The magnitude of the torque vector can be written as
A pair of electric charges with an equal magnitude but opposite charges separated by a distance is known as an electric dipole. The electric dipole moment is defined as the product of the magnitude of either charge and the distance between these charges. The electric dipole moment is a vector quantity having a defined direction from the negative charge to the positive charge.
The magnitude of the electric dipole moment p is given by
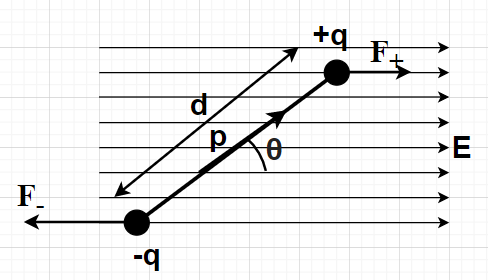
Consider an electric dipole with charges +q and –q forming a dipole since they are separated by distance d. Let it be placed in a uniform electric field of strength E such that the axis of the dipole forms an angle
The forces on these charges (+q and –q) when placed in the electric field having E as the electric field intensity are given by
Since,
The components of the forces exerted on the charges perpendicular to the dipole are given by
Since the magnitudes of the forces are equal. These charges are separated by a distance d, the torque on the dipole is given by:
Torque = Force
Since dipole moment is given by p = qd
Cross product of any two vectors
Using the formula
The above equation is the required equation which needed to be proved.
Note- The direction of the dipole moment is from the positive to the negative charge. Clearly, from the equation
Formulas Used:
Complete Step-by-Step solution:

To show:
The force measure which causes an object to rotate about an axis is called the torque. Torque is a quantity of vectors, and its direction depends on the axis direction of force. The magnitude of the torque vector can be written as
A pair of electric charges with an equal magnitude but opposite charges separated by a distance is known as an electric dipole. The electric dipole moment is defined as the product of the magnitude of either charge and the distance between these charges. The electric dipole moment is a vector quantity having a defined direction from the negative charge to the positive charge.
The magnitude of the electric dipole moment p is given by
Consider an electric dipole with charges +q and –q forming a dipole since they are separated by distance d. Let it be placed in a uniform electric field of strength E such that the axis of the dipole forms an angle
The forces on these charges (+q and –q) when placed in the electric field having E as the electric field intensity are given by
Since,
The components of the forces exerted on the charges perpendicular to the dipole are given by
Since the magnitudes of the forces are equal. These charges are separated by a distance d, the torque on the dipole is given by:
Torque = Force
Since dipole moment is given by p = qd
Cross product of any two vectors
Using the formula
The above equation is the required equation which needed to be proved.
Note- The direction of the dipole moment is from the positive to the negative charge. Clearly, from the equation
Recently Updated Pages
Express the following as a fraction and simplify a class 7 maths CBSE

The length and width of a rectangle are in ratio of class 7 maths CBSE

The ratio of the income to the expenditure of a family class 7 maths CBSE

How do you write 025 million in scientific notatio class 7 maths CBSE

How do you convert 295 meters per second to kilometers class 7 maths CBSE

Write the following in Roman numerals 25819 class 7 maths CBSE

Trending doubts
A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

What are the public facilities provided by the government? Also explain each facility

Difference between mass and weight class 10 physics CBSE

SI unit of electrical energy is A Joule B Kilowatt class 10 physics CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE




