
State the name of a regular polygon of 4 sides.
a. Square
b. Trapezium
c. Rectangle
d. Parallelogram
Answer
511.5k+ views
Hint: In order to solve this question, we will consider the properties of regular polygon and will check which of the quadrilateral satisfies all the properties, that will be the correct answer.
Complete step-by-step answer:
We will first see the properties of a regular polygon. So, we know that regular polygons are those which have all the sides of equal length. Also, they have length of every diagonals with
Now, if we consider square, trapezium, rectangle and parallelogram and then consider each property of regular polygon, then the quadrilateral satisfying all the properties will be the correct answer.
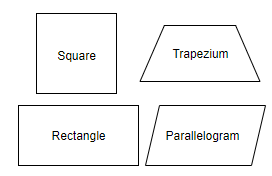
So, we know that all sides of regular polygon are equal and among all the quadrilaterals given, only square has all the sides equal.
We know that the length of every diagonal with the
We also know that the angles between two consecutive edges are also equal for a regular polygon. So, this condition is true for square and rectangle.
Now, we can see that square is the only quadrilateral which satisfies all the conditions of a regular polygon. Hence, square is the only quadrilateral which is a regular polygon of 4 sides. Therefore, option (a) is the correct answer.
Note: The possible mistake that one can make in this question, is by writing the answer as rectangle instead of square, as one might think that rectangle satisfies all the conditions, but actually rectangle satisfies only 2 out of the 3 conditions, whereas square satisfies all the 3 conditions. So, we need to remember that regular polygon is a polygon which has all the sides of equal length and all diagonals with the
Complete step-by-step answer:
We will first see the properties of a regular polygon. So, we know that regular polygons are those which have all the sides of equal length. Also, they have length of every diagonals with
Now, if we consider square, trapezium, rectangle and parallelogram and then consider each property of regular polygon, then the quadrilateral satisfying all the properties will be the correct answer.

So, we know that all sides of regular polygon are equal and among all the quadrilaterals given, only square has all the sides equal.
We know that the length of every diagonal with the
We also know that the angles between two consecutive edges are also equal for a regular polygon. So, this condition is true for square and rectangle.
Now, we can see that square is the only quadrilateral which satisfies all the conditions of a regular polygon. Hence, square is the only quadrilateral which is a regular polygon of 4 sides. Therefore, option (a) is the correct answer.
Note: The possible mistake that one can make in this question, is by writing the answer as rectangle instead of square, as one might think that rectangle satisfies all the conditions, but actually rectangle satisfies only 2 out of the 3 conditions, whereas square satisfies all the 3 conditions. So, we need to remember that regular polygon is a polygon which has all the sides of equal length and all diagonals with the
Recently Updated Pages
Express the following as a fraction and simplify a class 7 maths CBSE

The length and width of a rectangle are in ratio of class 7 maths CBSE

The ratio of the income to the expenditure of a family class 7 maths CBSE

How do you write 025 million in scientific notatio class 7 maths CBSE

How do you convert 295 meters per second to kilometers class 7 maths CBSE

Write the following in Roman numerals 25819 class 7 maths CBSE

Trending doubts
Full Form of IASDMIPSIFSIRSPOLICE class 7 social science CBSE

Fill in the blanks with appropriate modals a Drivers class 7 english CBSE

The southernmost point of the Indian mainland is known class 7 social studies CBSE

What crosssections do you get when you give a Vertical class 7 maths CBSE

What were the major teachings of Baba Guru Nanak class 7 social science CBSE

What are the controls affecting the climate of Ind class 7 social science CBSE





