
State true or false:
The side opposite to the equal angles of a triangle may be unequal.
a. true
b. false
Answer
490.5k+ views
1 likes
Hint: Given that Side opposite the equal angles of a triangle may be unequal, we check this statement by considering a triangle and draw a bisector from the unequal angle to divide the triangle into two. Then we can consider the properties of triangles to check the equality of two sides.
Complete step by step solution: Given that Side opposite the equal angles of a triangle may be unequal,
Let us consider a triangle XYZ with equal angles,
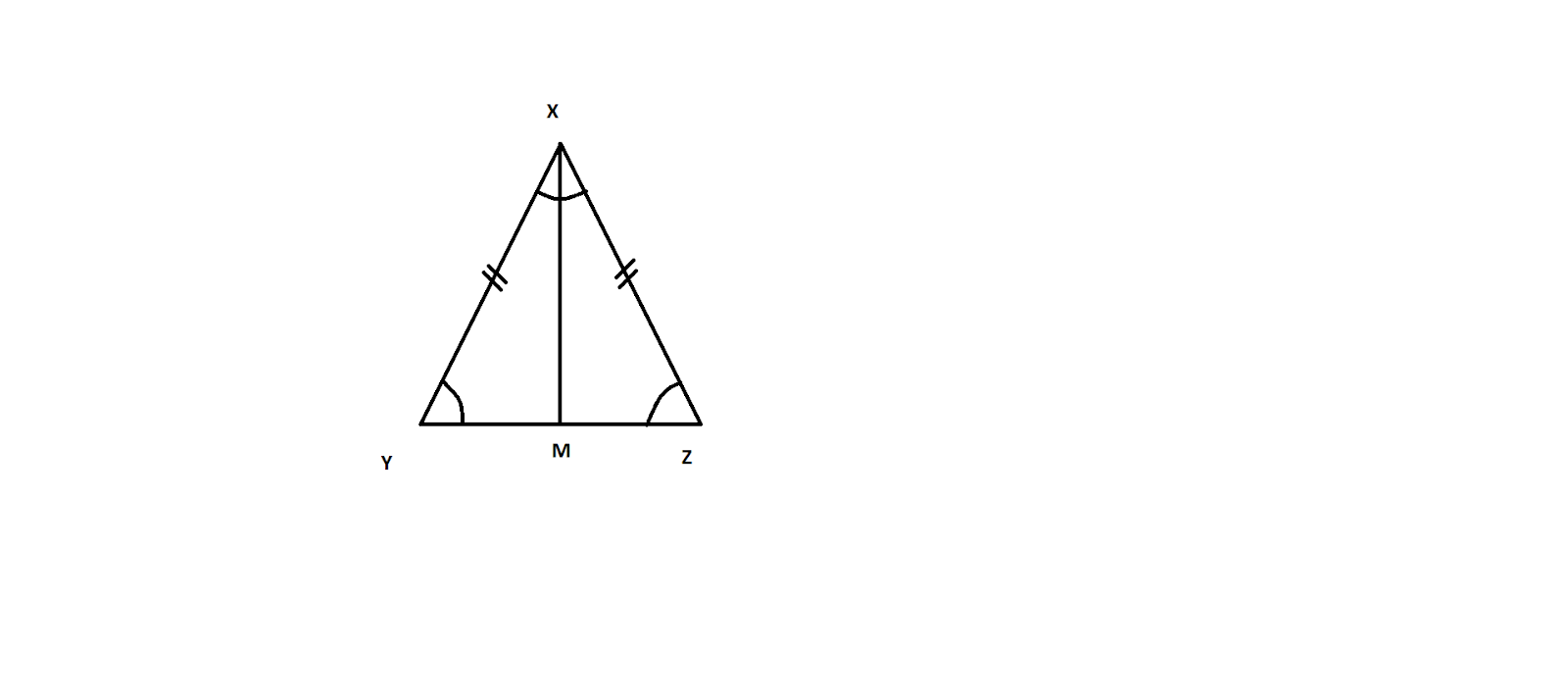
We can draw the angle bisector XM of
Now let us consider the triangles XMY and XMZ.
Consider the angles,
It is given that
As the side XM is common side for both the triangles, we can write,
Consider the angles,
As XM is the bisector of
Hence, from (1), (2) and (3) by AAS criteria, the triangles XMY and XMZ are congruent.
We know that corresponding parts of congruent triangles will be equal.
We get,
From the figure XY and XZ are the sides opposite to the equal angles.
So, the side opposite the equal angles of a triangle is equal
So, the given statement is FALSE.
Hence, option (b) is correct.
Note:
Here in the question we have been given that Side opposite the equal angles of a triangle may be unequal, so we can get confused seeing the word ‘maybe’, but as the statement is not sure and as we have proved that Side opposite the equal angles of a triangle is always equal, so we will consider the given statement is false.
The properties of congruence are what we have used here. There are three types of conditions on how we can use the properties of congruence. These are, AAS, SSS, SSA where S denotes the side and A denoting the angle.
Complete step by step solution: Given that Side opposite the equal angles of a triangle may be unequal,
Let us consider a triangle XYZ with equal angles,

We can draw the angle bisector XM of
Now let us consider the triangles XMY and XMZ.
Consider the angles,
It is given that
As the side XM is common side for both the triangles, we can write,
Consider the angles,
As XM is the bisector of
Hence, from (1), (2) and (3) by AAS criteria, the triangles XMY and XMZ are congruent.
We know that corresponding parts of congruent triangles will be equal.
We get,
From the figure XY and XZ are the sides opposite to the equal angles.
So, the side opposite the equal angles of a triangle is equal
So, the given statement is FALSE.
Hence, option (b) is correct.
Note:
Here in the question we have been given that Side opposite the equal angles of a triangle may be unequal, so we can get confused seeing the word ‘maybe’, but as the statement is not sure and as we have proved that Side opposite the equal angles of a triangle is always equal, so we will consider the given statement is false.
The properties of congruence are what we have used here. There are three types of conditions on how we can use the properties of congruence. These are, AAS, SSS, SSA where S denotes the side and A denoting the angle.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Questions & Answers - Ask your doubts

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Science: Engaging Questions & Answers for Success

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Difference Between Plant Cell and Animal Cell

Given that HCF 306 657 9 find the LCM 306 657 class 9 maths CBSE

The highest mountain peak in India is A Kanchenjunga class 9 social science CBSE

What is pollution? How many types of pollution? Define it

Differentiate between the Western and the Eastern class 9 social science CBSE




