
The angle measures of
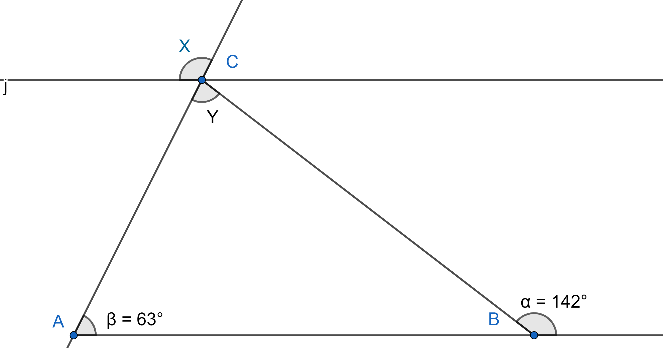
A)
B)
C)
D)

Answer
448.2k+ views
Hint: To solve this question we need to know the relation between the angles formed in the triangle. The angle formed on the straight line is
Complete step by step solution:
The question asks us to find the angles
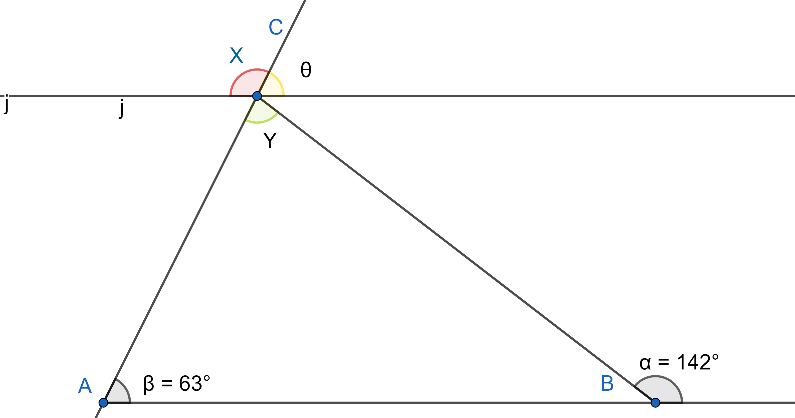
We will start with finding the angle
We can see in the figure
On substituting the values in equation (i), we get:
Now we will find the angle
To find angle
Substituting the value of
So, the correct answer is “Option C”.
Note: All the properties of the triangle should be known to us to solve the value for the angles. Angle
On putting the values we get:
So the method can also be used to find the angle of the triangle if the opposite exterior angle is given.
Complete step by step solution:
The question asks us to find the angles

We will start with finding the angle
We can see in the figure
On substituting the values in equation (i), we get:
Now we will find the angle
To find angle
Substituting the value of
So, the correct answer is “Option C”.
Note: All the properties of the triangle should be known to us to solve the value for the angles. Angle
On putting the values we get:
So the method can also be used to find the angle of the triangle if the opposite exterior angle is given.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Questions & Answers - Ask your doubts

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Science: Engaging Questions & Answers for Success

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Difference Between Plant Cell and Animal Cell

Given that HCF 306 657 9 find the LCM 306 657 class 9 maths CBSE

The highest mountain peak in India is A Kanchenjunga class 9 social science CBSE

What is pollution? How many types of pollution? Define it

Differentiate between the Western and the Eastern class 9 social science CBSE





