
The angular points of a triangle are$A{\text{( - 1, - 7), }}B(5,1),{\text{ }}C(1,4).$The equation of bisector of angle
$\angle ABC$ is
$
{\text{A}}{\text{. x}} = 7y + 2 \\
{\text{B}}{\text{. }}7y = x + 2 \\
{\text{C}}{\text{. }}y{\text{ = 7}}x{\text{ + 2}} \\
{\text{D}}{\text{. 7}}x = y + 2 \\
$
Answer
508.5k+ views
Hint: An angle bisector is a ray in the interior of an angle forming two congruent angles. In this problem you first need to find the coordinates of point $D$ which is on $AB$ using section formula and then find the equation of line $BD$.
Complete step-by-step answer:
Let $ABC$ is triangle with coordinates $A{\text{( - 1, - 7), }}B(5,1),{\text{ }}C(1,4).$Since, we know that the angle bisector of a triangle divides the opposite side into two segments that are proportional to the other sides of the triangle.
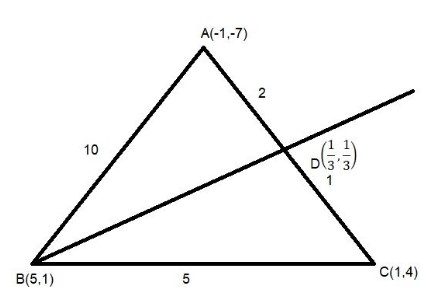
Since, the distance between two points is given by $\sqrt {{{({x_2} - {x_1})}^2} + {{({y_2} - {y_1})}^2}} $ where $({x_1},{y_1}){\text{ and }}({x_2},{y_2}){\text{ }}$are coordinates of two points.
Now, the length of $AB$
$
\Rightarrow \sqrt {{{\{ 5 - ( - 1)\} }^2} + {{\{ 1 - ( - 7)\} }^2}} \\
\Rightarrow \sqrt {{{(6)}^2} + {{(8)}^2}} \\
\Rightarrow 10 \\
$
And, the length of $BC$
$
\Rightarrow \sqrt {{{(1 - 5)}^2} + {{(4 - 1)}^2}} \\
\Rightarrow \sqrt {{{( - 4)}^2} + {{(3)}^2}} \\
\Rightarrow 5 \\
$
Now, the angular bisector of angle $\angle ABC$ is divides the opposite side $AC$ in ratio 10:5 or 2:1
By using Section Formula
If a point $P(x,y)$ divides the line joining two points $Q(a,b){\text{ and }}R(c,d)$ in the ratio $m:n$ internally, then the coordinates of $P(x,y)$ are given by
$ \Rightarrow P(x,y) = (\dfrac{{c.m + a.n}}{{m + n}},\dfrac{{d.m + b.n}}{{m + n}})$
Here $m=2$ , $n=1$ ,$A(-1,-7)$ and $C(1,4)$.
$ \Rightarrow P(x,y) = (\dfrac{{2.1 + 1.(-1)}}{{2 + 1}},\dfrac{{2.4 + 1.(-7)}}{{2 + 1}})$
Then, the coordinates of point $D$ on line $AB$
$
\Rightarrow D = (\dfrac{{ 2 - 1}}{3},\dfrac{{ 8 - 7}}{3}) \\
\Rightarrow D = (\dfrac{1}{3},\dfrac{1}{3}) \\
$
Since, the equation of line passing through two given points is given by$y - {y_1} = (\dfrac{{{y_2} - {y_1}}}{{{x_2} - {x_1}}})(x - {x_1})$where $({x_1},{y_1}){\text{ and (}}{x_2},{y_2})$ are coordinates of two points
Then equation of angular bisector$(BD)$ of angle $\angle ABC$ is given by
$
\Rightarrow {\text{ }}y - 1 = \dfrac{{(1 - \dfrac{1}{3})}}{{(5 - \dfrac{1}{3})}}(x - 5) \\
\Rightarrow y - 1 = \dfrac{2}{{14}}(x - 5) \\
\Rightarrow 7(y - 1) = (x - 5) \\
\Rightarrow x - 7y + 2 = 0 \\
\Rightarrow x + 2 = 7y \\
$
Hence, option B is correct.
Note: Whenever you get this type of question the key concept of solving this is to have knowledge about angular bisector ,its properties , Section Formula, formation of equation of line using two points which is given by $y - {y_1} = (\dfrac{{{y_2} - {y_1}}}{{{x_2} - {x_1}}})(x - {x_1})$ and how to apply them. You should make a rough diagram of the question to get a better understanding.
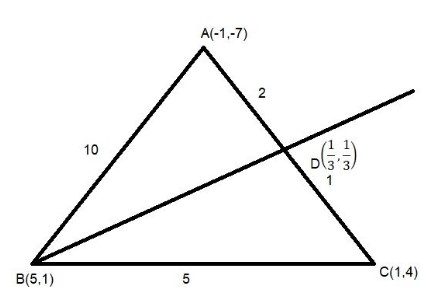
Complete step-by-step answer:
Let $ABC$ is triangle with coordinates $A{\text{( - 1, - 7), }}B(5,1),{\text{ }}C(1,4).$Since, we know that the angle bisector of a triangle divides the opposite side into two segments that are proportional to the other sides of the triangle.

Since, the distance between two points is given by $\sqrt {{{({x_2} - {x_1})}^2} + {{({y_2} - {y_1})}^2}} $ where $({x_1},{y_1}){\text{ and }}({x_2},{y_2}){\text{ }}$are coordinates of two points.
Now, the length of $AB$
$
\Rightarrow \sqrt {{{\{ 5 - ( - 1)\} }^2} + {{\{ 1 - ( - 7)\} }^2}} \\
\Rightarrow \sqrt {{{(6)}^2} + {{(8)}^2}} \\
\Rightarrow 10 \\
$
And, the length of $BC$
$
\Rightarrow \sqrt {{{(1 - 5)}^2} + {{(4 - 1)}^2}} \\
\Rightarrow \sqrt {{{( - 4)}^2} + {{(3)}^2}} \\
\Rightarrow 5 \\
$
Now, the angular bisector of angle $\angle ABC$ is divides the opposite side $AC$ in ratio 10:5 or 2:1
By using Section Formula
If a point $P(x,y)$ divides the line joining two points $Q(a,b){\text{ and }}R(c,d)$ in the ratio $m:n$ internally, then the coordinates of $P(x,y)$ are given by
$ \Rightarrow P(x,y) = (\dfrac{{c.m + a.n}}{{m + n}},\dfrac{{d.m + b.n}}{{m + n}})$
Here $m=2$ , $n=1$ ,$A(-1,-7)$ and $C(1,4)$.
$ \Rightarrow P(x,y) = (\dfrac{{2.1 + 1.(-1)}}{{2 + 1}},\dfrac{{2.4 + 1.(-7)}}{{2 + 1}})$
Then, the coordinates of point $D$ on line $AB$
$
\Rightarrow D = (\dfrac{{ 2 - 1}}{3},\dfrac{{ 8 - 7}}{3}) \\
\Rightarrow D = (\dfrac{1}{3},\dfrac{1}{3}) \\
$
Since, the equation of line passing through two given points is given by$y - {y_1} = (\dfrac{{{y_2} - {y_1}}}{{{x_2} - {x_1}}})(x - {x_1})$where $({x_1},{y_1}){\text{ and (}}{x_2},{y_2})$ are coordinates of two points
Then equation of angular bisector$(BD)$ of angle $\angle ABC$ is given by
$
\Rightarrow {\text{ }}y - 1 = \dfrac{{(1 - \dfrac{1}{3})}}{{(5 - \dfrac{1}{3})}}(x - 5) \\
\Rightarrow y - 1 = \dfrac{2}{{14}}(x - 5) \\
\Rightarrow 7(y - 1) = (x - 5) \\
\Rightarrow x - 7y + 2 = 0 \\
\Rightarrow x + 2 = 7y \\
$
Hence, option B is correct.
Note: Whenever you get this type of question the key concept of solving this is to have knowledge about angular bisector ,its properties , Section Formula, formation of equation of line using two points which is given by $y - {y_1} = (\dfrac{{{y_2} - {y_1}}}{{{x_2} - {x_1}}})(x - {x_1})$ and how to apply them. You should make a rough diagram of the question to get a better understanding.

Recently Updated Pages
Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 12 Economics: Engaging Questions & Answers for Success

Trending doubts
Which are the Top 10 Largest Countries of the World?

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

What is a transformer Explain the principle construction class 12 physics CBSE

Draw a labelled sketch of the human eye class 12 physics CBSE

What are the major means of transport Explain each class 12 social science CBSE

What is the Full Form of PVC, PET, HDPE, LDPE, PP and PS ?




