
The central angle of a semicircle is:
A.
B.
C.
D.
Answer
428.1k+ views
Hint: For solving this question you should know about the figure and area of a semicircle. A complete circle contains two semicircles in it. And we can prove it by an example. And then we can also find that the central angle of a semicircle is exactly half of the centre of a circle. So, for this we will prove the half area.
Complete step by step answer:
According to our question it is asked of us to find the central angle of a semicircle. So, if we take a circle with a radius of 7m and we see the diagram for this, then:
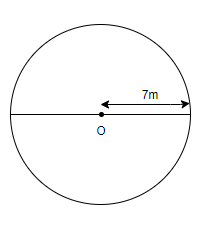
The circle’s centre is a point where the angle is
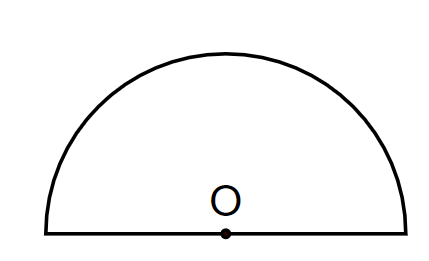
Here the centre is on a straight line and the angle on any point of a straight line is always
It is given that the radius of the circle is 7m. So, we know that the area of a circle is calculated by
Thus the area of a whole circle is:
So, the area of the whole circle is
We know that, to calculate the area of a semicircle, it is half of the whole circle. So, we can calculate it by the formula
So, the area of the semicircle is:
So, the area of the semicircle is exactly half of the whole circle and it is
So, the correct answer is “Option C”.
Note: The area of a semicircle is half the area of a circle of the same radius and a circle contains two semicircles in its area. We can directly do half of the complete area of the circle to calculate the area of a semicircle. And it is mandatory that the area will be of the same radius circle and semicircle of same radius.
Complete step by step answer:
According to our question it is asked of us to find the central angle of a semicircle. So, if we take a circle with a radius of 7m and we see the diagram for this, then:

The circle’s centre is a point where the angle is

Here the centre is on a straight line and the angle on any point of a straight line is always
It is given that the radius of the circle is 7m. So, we know that the area of a circle is calculated by
Thus the area of a whole circle is:
So, the area of the whole circle is
We know that, to calculate the area of a semicircle, it is half of the whole circle. So, we can calculate it by the formula
So, the area of the semicircle is:
So, the area of the semicircle is exactly half of the whole circle and it is
So, the correct answer is “Option C”.
Note: The area of a semicircle is half the area of a circle of the same radius and a circle contains two semicircles in its area. We can directly do half of the complete area of the circle to calculate the area of a semicircle. And it is mandatory that the area will be of the same radius circle and semicircle of same radius.
Latest Vedantu courses for you
Grade 10 | CBSE | SCHOOL | English
Vedantu 10 CBSE Pro Course - (2025-26)
₹ per year
Recently Updated Pages
Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 4 Maths: Engaging Questions & Answers for Success

Master Class 4 English: Engaging Questions & Answers for Success

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Difference Between Plant Cell and Animal Cell

Given that HCF 306 657 9 find the LCM 306 657 class 9 maths CBSE

The highest mountain peak in India is A Kanchenjunga class 9 social science CBSE

What is the difference between Atleast and Atmost in class 9 maths CBSE

What is pollution? How many types of pollution? Define it




