
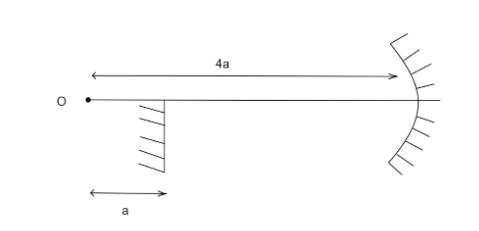
The diagram shows a mirror system. If after two reflections the image and the object O coincide with each other, then the value of the radius of curvature of the concave mirror is: (take $a = 3m$)
(A) $4m$
(B) $6m$
(C) $8m$
(D) $10m$
Answer
124.8k+ views
Hint The radius of the curvature of a mirror is twice of its focal length. Focal length can easily be calculated if the image and object position is given. For a plane mirror, the object distance is equal to image distance.
Step by step solution
Two conditions are given for this problem. First, the object is kept at O, i.e. a distance $4a$ from the concave mirror. Second, the final image after reflection from the plane mirror forms at O i.e. at a distance of $a$ behind the plane mirror.
Since object distance and image distance are always equal for a plane mirror, we can say that the object for the plane mirror is placed at position $P$
Using the given condition that reflection takes place twice, we can conclude that first, it reflects from the concave mirror and then from the plane mirror to make the final image at O. This implies that the object for the plane mirror is actually the image from the concave mirror.
So, we have the object distance and image distance for the concave mirror.
$u = - 4a$
And, $v = - 2a$.
Where, $u$ object distance
$v$ is the image distance.
The negative sign represents that these coordinates are on the left side of the pole of the mirror.
Using mirror formula,
$\dfrac{1}{v} + \dfrac{1}{u} = \dfrac{1}{f}$
Putting the values, we get
$\dfrac{1}{{ - 2a}} + \dfrac{1}{{ - 4a}} = \dfrac{1}{f}$
$ \Rightarrow \dfrac{{ - 2 - 1}}{{4a}} = \dfrac{1}{f}$
Solving this
$\dfrac{1}{f} = \dfrac{{ - 3}}{{4a}}$
We know that, radius of curvature of a mirror is given by twice its focal length.
$R = 2f$
$ \Rightarrow R = 2\left( {\dfrac{{ - 4a}}{3}} \right)$
Putting $a = 3m$, we get
$R = 8m$.
Hence, Option (C) is correct.
Note The basic concept is to remember the mirror formulas. You should be careful if you are taking the numeric value of the image and object distance because it can easily lead you to error. A better way is to solve it first and then put the numeric value.
Step by step solution
Two conditions are given for this problem. First, the object is kept at O, i.e. a distance $4a$ from the concave mirror. Second, the final image after reflection from the plane mirror forms at O i.e. at a distance of $a$ behind the plane mirror.
Since object distance and image distance are always equal for a plane mirror, we can say that the object for the plane mirror is placed at position $P$
Using the given condition that reflection takes place twice, we can conclude that first, it reflects from the concave mirror and then from the plane mirror to make the final image at O. This implies that the object for the plane mirror is actually the image from the concave mirror.
So, we have the object distance and image distance for the concave mirror.
$u = - 4a$
And, $v = - 2a$.
Where, $u$ object distance
$v$ is the image distance.
The negative sign represents that these coordinates are on the left side of the pole of the mirror.
Using mirror formula,
$\dfrac{1}{v} + \dfrac{1}{u} = \dfrac{1}{f}$
Putting the values, we get
$\dfrac{1}{{ - 2a}} + \dfrac{1}{{ - 4a}} = \dfrac{1}{f}$
$ \Rightarrow \dfrac{{ - 2 - 1}}{{4a}} = \dfrac{1}{f}$
Solving this
$\dfrac{1}{f} = \dfrac{{ - 3}}{{4a}}$
We know that, radius of curvature of a mirror is given by twice its focal length.
$R = 2f$
$ \Rightarrow R = 2\left( {\dfrac{{ - 4a}}{3}} \right)$
Putting $a = 3m$, we get
$R = 8m$.
Hence, Option (C) is correct.
Note The basic concept is to remember the mirror formulas. You should be careful if you are taking the numeric value of the image and object distance because it can easily lead you to error. A better way is to solve it first and then put the numeric value.
Recently Updated Pages
JEE Main 2021 July 20 Shift 2 Question Paper with Answer Key

JEE Atomic Structure and Chemical Bonding important Concepts and Tips

JEE Amino Acids and Peptides Important Concepts and Tips for Exam Preparation

JEE Main 2023 (April 8th Shift 2) Physics Question Paper with Answer Key

JEE Main 2023 (January 30th Shift 2) Maths Question Paper with Answer Key

JEE Main 2022 (July 25th Shift 2) Physics Question Paper with Answer Key

Trending doubts
The formula of the kinetic mass of a photon is Where class 12 physics JEE_Main

JEE Main 2023 January 24 Shift 2 Question Paper with Answer Keys & Solutions

Learn About Angle Of Deviation In Prism: JEE Main Physics 2025

JEE Main Login 2045: Step-by-Step Instructions and Details

Ideal and Non-Ideal Solutions Raoult's Law - JEE

Explain the construction and working of a GeigerMuller class 12 physics JEE_Main

Other Pages
Inertial and Non-Inertial Frame of Reference - JEE Important Topic

Charging and Discharging of Capacitor

Clemmenson and Wolff Kishner Reductions for JEE

Geostationary Satellites and Geosynchronous Satellites - JEE Important Topic

JEE Main 2022 June 29 Shift 2 Question Paper with Answer Keys & Solutions

Current Loop as Magnetic Dipole and Its Derivation for JEE




