
The diameter of a roller is 84 cm and its length is 120 cm. It takes 500 complete revolutions to move once over a level a playground. Find the area of the playground in
(a)
(b)
(c)
(d) none of the above
Answer
528k+ views
Hint: The roller is in the shape of a right circular cylinder. To find the area that the roller covers the playground in five revolutions, we need to find the lateral area of the roller. The lateral area of the roller is equal to the area of its curved surface.
Complete step-by-step solution -
The formula for finding the lateral area of a right circular cylinder is:
Since the roller is positioned horizontal as it rolls along the playground, then the length of the roller is actually its height.
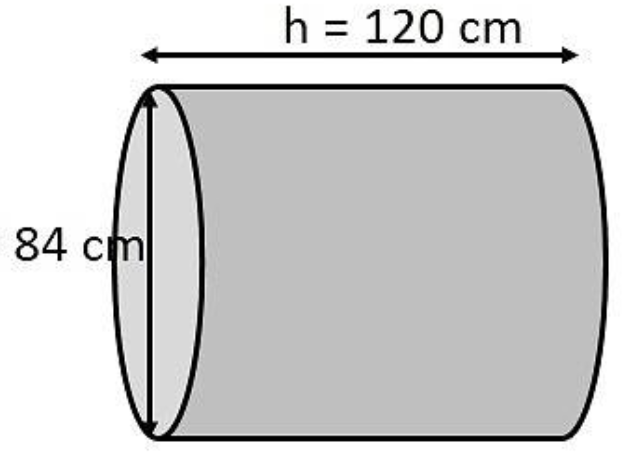
Now in the question diameter is mentioned and we need to find a radius which is half of diameter.
Diameter of the roller
Radius of the roller
Height of the roller
Now putting value of r and h in equation (1) to get the lateral area of the roller we get,
In question the given number of revolutions is 500 that is
Converting this area in
Hence the area covered by the roller in the 500 revolution is
Note: The key here is to understand that the roller is nothing but a cylinder. Knowing the formula of lateral area is important and radius is always half of the diameter. We in a hurry may substitute diameter which is 84 cm in place of radius which is 42 cm. We need to be careful with the units too because if a unit is missing some marks would be deducted by the examiner.
Complete step-by-step solution -
The formula for finding the lateral area of a right circular cylinder is:
Since the roller is positioned horizontal as it rolls along the playground, then the length of the roller is actually its height.

Now in the question diameter is mentioned and we need to find a radius which is half of diameter.
Diameter of the roller
Radius of the roller
Height of the roller
Now putting value of r and h in equation (1) to get the lateral area of the roller we get,
In question the given number of revolutions is 500 that is
Converting this area in
Hence the area covered by the roller in the 500 revolution is
Note: The key here is to understand that the roller is nothing but a cylinder. Knowing the formula of lateral area is important and radius is always half of the diameter. We in a hurry may substitute diameter which is 84 cm in place of radius which is 42 cm. We need to be careful with the units too because if a unit is missing some marks would be deducted by the examiner.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Questions & Answers - Ask your doubts

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Science: Engaging Questions & Answers for Success

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Difference Between Plant Cell and Animal Cell

Given that HCF 306 657 9 find the LCM 306 657 class 9 maths CBSE

The highest mountain peak in India is A Kanchenjunga class 9 social science CBSE

What is pollution? How many types of pollution? Define it

Differentiate between the Western and the Eastern class 9 social science CBSE




