
The equivalent resistance between diagonally opposite corners A and B is:

A.
B.
C.
D.

Answer
470.4k+ views
Hint: Use the formulae for the equivalent resistance for the resistors connected in series arrangement and parallel arrangement. Observe the given circuit and draw the equivalent circuit diagram of the given circuit. From this equivalent circuit diagram, determine the net resistance between the terminals A and B using the formula for equivalent resistance of series and parallel combination.
Formulae used:
The equivalent resistance
The equivalent resistance
Complete step by step answer:
We have given a circuit diagram in which the resistors of resistance
Therefore, the equivalent circuit diagram for the given circuit can be drawn as follows:
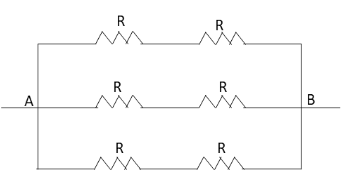
In the above circuit diagram, three rows in which two resistors are connected in series are in parallel. The equivalent resistance
Hence, the equivalent resistance of the two resistors connected in series is
Substitute
Therefore, the equivalent resistance between the diagonally opposite terminals is
Hence, the correct option is C.
Note: The students should be careful while drawing the equivalent circuit diagram of the given circuit. While drawing this equivalent circuit diagram, the students should check the potential difference across each resistor connected in the circuit. The potential difference across the resistors in the vertical arm CD of the given circuit diagram is zero. Hence, we should eliminate this branch while drawing this circuit diagram.
Formulae used:
The equivalent resistance
The equivalent resistance
Complete step by step answer:
We have given a circuit diagram in which the resistors of resistance
Therefore, the equivalent circuit diagram for the given circuit can be drawn as follows:

In the above circuit diagram, three rows in which two resistors are connected in series are in parallel. The equivalent resistance
Hence, the equivalent resistance of the two resistors connected in series is
Substitute
Therefore, the equivalent resistance between the diagonally opposite terminals is
Hence, the correct option is C.
Note: The students should be careful while drawing the equivalent circuit diagram of the given circuit. While drawing this equivalent circuit diagram, the students should check the potential difference across each resistor connected in the circuit. The potential difference across the resistors in the vertical arm CD of the given circuit diagram is zero. Hence, we should eliminate this branch while drawing this circuit diagram.
Latest Vedantu courses for you
Grade 10 | CBSE | SCHOOL | English
Vedantu 10 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
Recently Updated Pages
Express the following as a fraction and simplify a class 7 maths CBSE

The length and width of a rectangle are in ratio of class 7 maths CBSE

The ratio of the income to the expenditure of a family class 7 maths CBSE

How do you write 025 million in scientific notatio class 7 maths CBSE

How do you convert 295 meters per second to kilometers class 7 maths CBSE

Write the following in Roman numerals 25819 class 7 maths CBSE

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE

Draw a diagram of a flower and name the parts class 12 biology ICSE




