
The given figure shows a pentagon ABCDE , EG drawn parallel to DA meets BA produced at G and CF drawn to DB meets AB produced at F.

Prove that the area of pentagon ABCDE is equal to the area of triangle GDF.

Answer
485.4k+ views
Hint:
By considering the triangles DGA and AED , DBC and BFD and using the property that the triangles having the same base and lie between the same parallel line have the same area we get two equations and adding the Area of
Complete step by step solution:
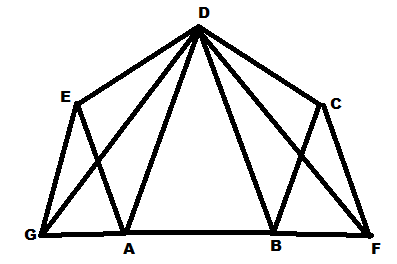
Let's consider the triangles DGA and AED
We can see that both the triangles have the same base AD and lie between the parallel lines AD and EG
So we get that
Area of
Now let's consider the triangles DBC and BFD
We can see that both the triangles have the same base DB and lie between the parallel lines BD and CF
So we get that
Area of
Now by adding (1) and (2) we get
Area of
Now adding Area of
Area of
From the diagram we get that
Area of
Area of pentagon ABCDE = Area of
Substituting (4) and (5) in (3) we get
Area of
Hence proved.
Note:
The sum of all the internal angles of a triangle is always
The length of any side of a triangle is shorter than the sum of the other two sides.
A triangle can always be split into two right triangles no matter how the triangle is constructed.
By considering the triangles DGA and AED , DBC and BFD and using the property that the triangles having the same base and lie between the same parallel line have the same area we get two equations and adding the Area of
Complete step by step solution:

Let's consider the triangles DGA and AED
We can see that both the triangles have the same base AD and lie between the parallel lines AD and EG
So we get that
Area of
Now let's consider the triangles DBC and BFD
We can see that both the triangles have the same base DB and lie between the parallel lines BD and CF
So we get that
Area of
Now by adding (1) and (2) we get
Area of
Now adding Area of
Area of
From the diagram we get that
Area of
Area of pentagon ABCDE = Area of
Substituting (4) and (5) in (3) we get
Area of
Hence proved.
Note:
The sum of all the internal angles of a triangle is always
The length of any side of a triangle is shorter than the sum of the other two sides.
A triangle can always be split into two right triangles no matter how the triangle is constructed.
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Difference Between Plant Cell and Animal Cell

Given that HCF 306 657 9 find the LCM 306 657 class 9 maths CBSE

The highest mountain peak in India is A Kanchenjunga class 9 social science CBSE

What is the difference between Atleast and Atmost in class 9 maths CBSE

What is pollution? How many types of pollution? Define it





