Question

The Gurgaon office of Microsoft has 1500 executives. Of these 900 subscribe to the Hindustan Times and 750 subscribe to the Hindus. 150 subscribe to both Hindustan Times and the Hindu. If an executive is picked at Random, what is the probability that he has subscribed to Hindustan times?
$
  (a)\dfrac{2}{5} \\
  (b)\dfrac{3}{5} \\
  (c)\dfrac{4}{5} \\
  (d)\dfrac{1}{5} \\
 $

Answer 1

Hint – In this question find the number of executives who have only subscribed the Hindustan times that is the number of executives who subscribed to Hindustan times subtracted with the number of executives who have subscribed both Hindustan times and The Hindu. Similarly find the number of executives who have only subscribed the Hindu that is the number of executives who subscribed to Hindu subtracted with the number of executives who have subscribed both Hindustan times and The Hindu. Then use the basic of probability that is $P = \dfrac{{{\text{favorable number of outcomes}}}}{{{\text{total number of outcomes}}}}$. This will help approaching the problems.

Complete step-by-step answer:
Given data:
Total executives in the Gurgaon office = 1500 executives.
Therefore, n (E) = 1500.
 Out of these 1500 executives 900 subscribe to the Hindustan times and 750 subscribes to The Hindu.
150 subscribes to both Hindustan times and The Hindu.
So the number of subscribers who only subscribe to Hindustan times = (900 – 150) = 750 executives.
And the number of subscribers who only subscribe The Hindu = (750 – 150) = 600 executives.
Now an executive is randomly picked we have to find out the probability that he has subscribed to the Hindustan times.
As we all know that the probability is the ratio of the favorable number of outcomes to the total number of outcomes so we have,
Therefore, the probability that the randomly picked executive has subscribe the Hindustan times is
$ \Rightarrow P = \dfrac{{{\text{favorable number of outcomes}}}}{{{\text{total number of outcomes}}}}$
Now the favorable number of outcomes is the subscribers of the Hindustan times = 900 (including the 150 subscribers of The Hindu).
And the favorable number of outcomes is the total number of executives = 1500.
So the probability, $P = \dfrac{{{\text{900}}}}{{{\text{1500}}}} = \dfrac{9}{{15}} = \dfrac{3}{5}$
So this the required probability that the randomly picked executive has subscribed to the Hindustan times.
So this is the required answer.
Hence option (B) is the correct answer.

Note – The trick point here was the concept that how we have taken the only number of executives who has subscribed to the Hindu or the total number of executives who has subscribed to the Hindustan Times. Consider this vein diagram

Consider A as Hindustan Times and B as Hindu so clearly A is comprised of some portion of A (marked in red) and some of the intersection of both A and B (marked in blue)

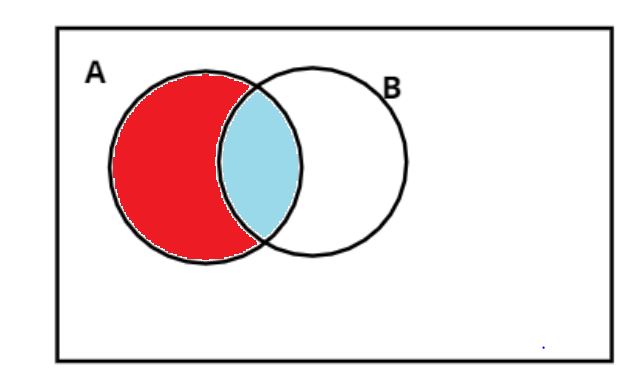
So to get the number of executives that are only subscribed to the Hindustan times that is the red region this blue region is to be removed from entire A and that’s how we have calculated executives who have only subscribed the Hindustan times.